1. Calculate the area of the
figure below. Round to the
hundredths place when
necessary.

Answers
Answer:
The area is 88.39 cm
Step-by-step explanation:
Here, we want to find the area of the semicircle given that the diameter of the semi circle is 15 cm
We start by getting the radius
Mathematically r = D/2
= 15/2 = 7.5
Area of semicircle is area of circle/2
= (pi * r^2)/2
= (22/7 * 7.5^2)/2 = 88.39 cm
Related Questions
A Right triangle has one acute angle of 43. what is the measure of the other acute angle
90 + 43 + x = 180
Answers
By Euclidean geometry, the measure of the missing angle, the other acute angle, of the right triangle is equal to 47°.
How to find the measure of the measing angle of the right triangleRight triangles are triangles of which one of its angles is a right angle and the other two angles are acute angles. According to the statement, the measure of an acute angle of the right triangle is equal to 43°.
By Euclidean geometry, the sum of the internal angles in any triangle equals a measure of 180°. Then, the measure of the missing angle is:
90° + α + β = 180°
If we know that α = 43°, then the value of β is:
α + β = 90°
43° + β = 90°
β = 47°
The measure of the missing angle is equal to 47°.
To learn more on right triangles: https://brainly.com/question/2869318
#SPJ1
What is the outlier for the data set?
A. 5
B. 9
C. 10
D. there is none

Answers
Answer:
The answer to your problem is, A. 5
Step-by-step explanation:
What is an outlier?
What just like it says an outlier it something “ away “ or “ out of civilization of its fellow numbers “
You can see that the hours of 8 - 10 they are all bunched up together
But you can see hour 5 seems a bit lonely
Which then we call he outlier
Thus the answer to your problem is, A. 5
Hey could y’all please help me with this question

Answers
The required cost of each shirt is $21.28.
What is Equation?A mathematical statement known as an equation is made up of two expressions joined together by the equal symbol. A formula would be 3x - 5 = 16, for instance. When this equation is solved, we discover that the value of the quantity x is 7.
According to question:The total cost of 3 identical shirts, including shipping, is $71.83. So we can write the equation as:
3s + 7.99 = 71.83
To solve for the cost of each shirt, we need to isolate the variable "s" on one side of the equation. We can start by subtracting 7.99 from both sides:
3s = 63.84
Then, we can divide both sides by 3:
s = 21.28
Therefore, the cost of each shirt is $21.28.
To know more about Equation visit:
brainly.com/question/28243079
#SPJ1
The value of y is directly proportional to the value of x. When x = 3.5, the value of y
is 14. What is the value of y when x = 7?
Answers
In the proportional relationship, the value of y when x = 7 is 28.
How to find the value of variables in a directly proportional relationship?Direct proportion or direct variation is the relation between two quantities where the ratio of the two is equal to a constant value.
Therefore, the value of y is directly proportional to the value of x. When x = 3.5, the value of y is 14.
Hence,
y ∝ x
y = kx
where
k = constant of proportionalitywhen y = 14 , x = 3.5
14 = 3.5k
k = 14 / 3.5
k = 4
Therefore, let's find the value of y when x = 7.
y = 4 × 7
y = 28
learn more on proportional relationship here: https://brainly.com/question/24993531
#SPJ1
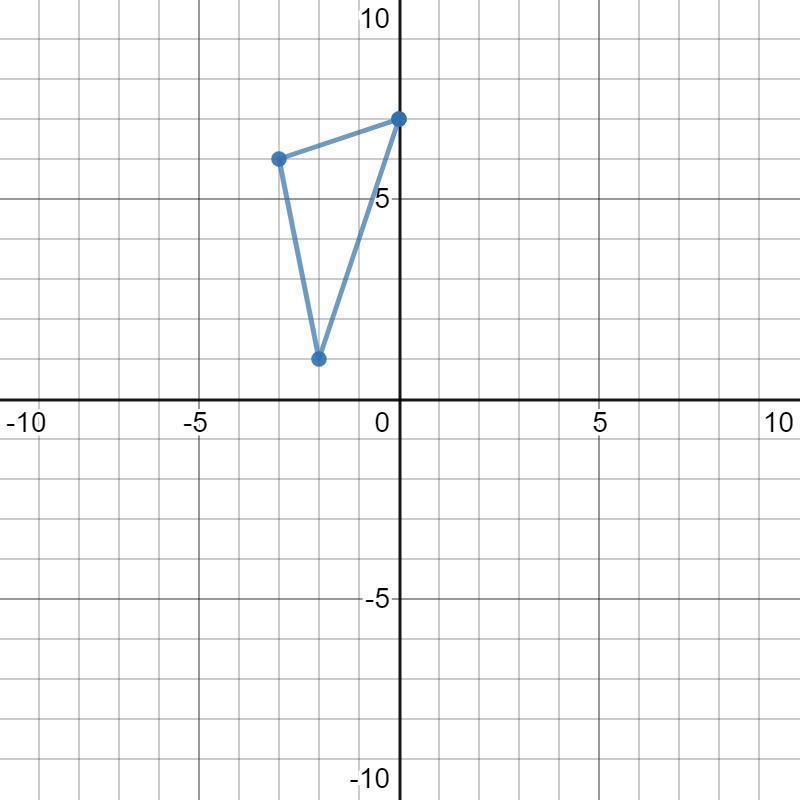
please help me Mrs. Smith has a large grid drawn on a whiteboard at the front of her classroom. She and her geometry students use an erasable marker to plot shapes on it. She plots Triangle 1, which is shown on the grid below.

Answers
The vertexes of the original triangle are the points (-2,4), (-4,-2) and (-5,3). After the transformation the new vertexes will be:
\(\begin{gathered} (-2,4)\rightarrow(0,7) \\ (-4,-2)\rightarrow(-2,1) \\ (-5,3)\rightarrow(-3,6) \end{gathered}\)Then, the new triangle is:
Members of the science club voted for their favorite planet. The picture graph shows the total votes. How many more members voted for Mars than for Earth?

Answers
The members voted 2 more for mars than earth.
What are the number of planets in the solar system?The Solar System has at least eight planets: the terrestrial planets Mercury, Venus, Earth and Mars, and the giant planets Jupiter, Saturn, Uranus and Neptune.
Given here, Mars has 8 votes while earth has 6 votes
Thus difference in votes is =8-6
= 2
Hence, Mars got two more members than earth
Learn more about the solar system here:
https://brainly.com/question/12075871
#SPJ1
Triangle abc is a right triangle find x

Answers
Answer:
25Step-by-step explanation:
from the figure and the values we understand that it is an isosceles right triangle, therefore with the two sides and the 2 angles are congruent,
so x = 25

Determine whether the sequence -10, -7,-4, -1,... is an arithmetic sequence. Write yes or no. If so state the
common difference.
Answers
d = a(n) - a(n-1)
*a(n) is a term in the sequence and a(n-1) is the previous term in the sequence.
d = -1 - (-4) = -1 + 4 = 3
Answer:
Yes
Step-by-step explanation:
Yes because the distance between each of its terms is equal

Find the surface area of a regular pentagonal prism with a height of 3.5 inches and a base edge length of 2 inches. Round your answer to the nearest hundredth, if necessary.
Answers
Answer: 48.76 square inches.
Step-by-step explanation: A regular pentagonal prism has 7 faces: 2 pentagonal bases and 5 rectangular faces.
To find the surface area, we need to find the area of each face and add them up.
The area of one pentagonal base can be found using the formula:
A = (5/4) * (edge length)^2 * cot(π/5)
Substituting the given values, we get:
A = (5/4) * (2)^2 * cot(π/5)
≈ 6.8819 square inches (rounded to the nearest hundredth)
Since there are two pentagonal bases, their total area is:
2A ≈ 13.7638 square inches
The area of one rectangular face can be found using the formula:
A = (edge length) * (height)
Substituting the given values, we get:
A = 2 * 3.5
= 7 square inches
Since there are five rectangular faces, their total area is:
5A = 5 * 7 = 35 square inches
Therefore, the total surface area of the regular pentagonal prism is approximately:
13.7638 + 35 = 48.7638 square inches
Rounding to the nearest hundredth, we get:
Surface area ≈ 48.76 square inches.
The surface area of a regular pentagonal prism is 193.82 square inches.
What is a prism?A prism is a three-dimensional object.
There are triangular prism and rectangular prism.
We have,
The formula for the surface area of a regular pentagonal prism is:
SA = 5 × base area + 5 × lateral face area
where the base area is the area of one of the pentagonal bases, and the lateral face area is the area of one of the rectangular faces.
Since the base is a regular pentagon, we can use the formula for the area of a regular pentagon:
Area of pentagon = (1/4) × n × s² × tan(180°/n)
where n is the number of sides of the pentagon (which is 5 since it's a regular pentagon), and s is the length of one of its sides.
In this case,
s = 2 inches
Area of pentagon = (1/4) × 5 × 2² × tan(180°/5)
Area of the pentagon = 6.8819 square inches
This is the area of one of the pentagonal bases.
Since there are two bases,
The total base area is:
Base area = 2 × 6.8819
= 13.7638 square inches
Now,
Each lateral face is a rectangle with a width equal to the base edge length (2 inches) and a height equal to the height of the prism (3.5 inches).
Lateral face area.
= base edge length × height
= 2 inches × 3.5 inches
= 7 square inches
Since there are five lateral faces, the total lateral face area is:
Lateral face area
= 5 × 7
= 35 square inches
Now we can add up the base area and lateral face area to get the total surface area:
SA = 5 × base area + 5 × lateral face area
= 5(13.7638) + 5(35)
= 193.819 square inches
Rounding this to the nearest hundredth.
SA = 193.82 square inches
Thus,
The surface area of a regular pentagonal prism is 193.82 square inches.
Learn more about Prism here:
https://brainly.com/question/12649592
#SPJ2
I need a lot of help!
Is 54.03 + 4.04 greater than 54 + 4.4?
Answers
Answer:
54+4.4 is greater
Step-by-step explanation:
if u need explanation than please ask
Let (an) -1 be a sequence of real numbers and let f : [1,00) +R be a function that is integrable on [1, 6] for every b > 1. Prove or disprove each of the following statements: (a) If a f(x) dx is convergent, then § f(n) is convergent. (b) We have: Ž ith53 1+2 n=0 (c) If È an is convergent, then Î . is convergent. nal n=1 (d) If an converges absolutely, then am is convergent.
Answers
The statement (d) is true.
Given that (an) -1 is a sequence of real numbers and f: [1,00) +R is a function that is integrable on [1,6] for every b > 1. We have to prove or disprove the following statements:a) If a f(x) dx is convergent, then § f(n) is convergent.b) We have: Ž ith53 1+2 n=0c) If È an is convergent, then Î . is convergent.d) If an converges absolutely, then am is convergent.(a) If f(x)dx is convergent, then §f(n) is convergent.Statement a is true.Proof:If f(x)dx is convergent, then limm→∞ ∫1mf(x)dx exists.Using the summation by parts formula, we get:∫1mf(x)dx = (m − 1)∫1mf(x)·1m−1dx + ∫1mf′(x)·1−1mdxRearranging the above equation, we get:f(m) = 1m−1∫1mf(x)dx − 1m−1 ∫1mf′(x)·1−1mdxSince limm→∞ f′(x)·1−1m = 0 for every x ∈ [1, 6], it follows that limm→∞∫1mf′(x)·1−1mdx = 0Therefore, limm→∞f(m) = limm→∞1m−1∫1mf(x)dx exists. Therefore, the statement (a) is true.(b) We have: Ž ith53 1+2 n=0Statement b is false since the series diverges.(c) If Èan is convergent, then Î.an is convergent.Statement c is false.Proof:Since f(x) is integrable on [1, 6] for every b > 1, it follows that f(x) is bounded on [1, 6].Let M be such that f(x) ≤ M for every x ∈ [1, 6].Given that ∑n=1∞ an converges, it follows that limn→∞an = 0Since f(x) is integrable on [1, 6] for every b > 1, it follows that limx→∞f(x) = 0Therefore, we have:limn→∞∣∣∣∣∫n+1n(f(x)−an)dx∣∣∣∣≤Mlimn→∞∣∣∣∣∫n+1n(f(x)−an)dx∣∣∣∣=Mlimn→∞an=0Since the limit of the integral is zero, it follows that limn→∞∫∞1(f(x)−an)dx exists. But this limit is not equal to zero since it is equal to limn→∞f(n) which does not exist. Therefore, the statement (c) is false.(d) If ∑n=1∞ |an| converges, then ∑n=1∞ an converges. Statement d is true. Proof: Since ∑n=1∞ |an| converges, it follows that limn→∞|an| = 0 Therefore, there exists a number M such that |an| ≤ M for every n. By the comparison test, it follows that ∑n=1∞ an converges.
Know more about real numbers here:
https://brainly.com/question/31715634
#SPJ11
3.
Simplify the expression
10
O
0 4000
7
017
O
121121
O
20
100
49
(1 point) need to turn in by 12
Answers
The simplified expression is 10 + 4000 + 7 + 17 + 121121 + 20 + 100 + 49.
What is expression?In mathematics, an expression is a combination of numbers, variables, operators, and/or constants which evaluates to a single value. It can also be thought of as a combination of symbols that represent a value. Expressions are used to make calculations and solve equations. Examples of expressions include 3 + 5 = 8, x2 + 5x + 6 = 0, and 5/3. Expressions are fundamental to mathematics as they are used to represent mathematical concepts and operations.
Simplifying an expression means to reduce it to its most basic form. In this case, the expression contains a mix of numbers, which can all be reduced to their lowest form. 10 can be reduced to 10, 4000 can be reduced to 4000, 7 can be reduced to 7, 017 can be reduced to 17, 121121 can be reduced to 121121, 20 can be reduced to 20, 100 can be reduced to 100 and 49 can be reduced to 49.
Therefore, the simplified expression is 10 + 4000 + 7 + 17 + 121121 + 20 + 100 + 49.
To know more about expression click-
https://brainly.com/question/1859113
#SPJ1
What is your favorite marvel character?
OR
What is your favorite Percy Jackson character?
10 points!
Answers
Answer:
nico
Step-by-step explanation:
Please Answer ASAP!!!
2x+x-6/2=0
Solve for x
Answers
Answer:
The answer is x<2
-2x^5 + 14x^4 - 36x^3 + 52x^2 - 64x + 48=0
Step-by-step explanation:
How many different 7-place license plates are possible if the first 2 places are for letters and the other 5 for numbers probability?
Answers
The total number of possible 7-place license plates are 67600000.
Given: A 7-plate license plate. 2 places are for letters and 5 places are for numbers. To find how many different 7-plate license plates are possible
Let's solve the given problem:
The license plate has 7 places. 2 places are for letters and the remaining 5 places are for numbers.
Combination of letters: As there are no restrictions given in the question, so the first letter can be any alphabet out of the 26 alphabets (A, B, C, D, ......... Z). So the first place for the letter can be filled in ²⁶C₁ ways that are 26 ways. Also for the second place, as the letters can repeat so it can be filled in ²⁶C₁ ways too which are 26 ways. Therefore, the possible ways in which the place for two letters can be filled is 26 × 26 ways = 676 ways.Combination of numbers: As there are no restrictions given in the question so the first number can be any of the numbers out of the 10 numbers (10, 1, 2, 3, ....... 9). So the first number can be filled in ¹⁰C₁ = 10 ways. Similarly, as the numbers can repeat so the 2nd, 3rd, 4th and 5th numbers can be filled in ¹⁰C₁ ways that all the other places can be filled in 10 ways each. Therefore the total number of ways in which the place for 5 numbers can be filled is 10 × 10 × 10 × 10 × 10 ways = 100000 ways.Therefore, the total number of ways in which the 7-place license plate can fill are: Total possible ways in which the two letters can be filled × Total possible ways in which the 5 number places can be filled
= 676 × 100000
= 67600000 ways
Hence the total number of possible 7-place license plates are 67600000.
Know more about "permutations and combinations" here: https://brainly.com/question/13387529
#SPJ6
i need help asap please and thanks

Answers
Answer:
It will be 29.39
Step-by-step explanation:
Hope this Helped
\( \sin ^{ - 1} ( \frac{4}{9}) = 26.39\)
12years ago Justin Timberlake put money into an account paying 6.5% interest compounded biannually. If the account has $18,000 now, how much money did Justin Timberlake deposit?
Answers
Answer:
$8645.73
Step-by-step explanation:
Using the compound interest formula :
A = P(1 + r/n)^nt
A = final amount = 18000
r = rate = 6.5% = 0.065
n = number of compounding tines per period, biannually, once in 2 years = 0.5
18000 = P(1 + 0.065/0.5)^(0.5*12)
18000 = P(1 + 0.13)^6
18000 = P(2.081951752609)
18000 / 2.081951752609 = P
= 8645.7334
= $8645.73
Which statements correctly describe the graph of the function f(x) = x3 – 2x2 – 19x 20? Select three options.
Answers
The graph of the function f(x) = x³ – 2x² – 19x + 20 passes through the x-axis at –4, 1, and 5.
What is a factorization?It is the method to separate the polynomial into parts and the parts will be in multiplication. And the value of the polynomial at this point will be zero.
The function is given as
f(x) = x³ – 2x² – 19x + 20
By hit and trial. Let x = 1, we have
f(x) = 1³ – 2(1)² – 19(1) + 20 = 0
Then the equation will be
f(x) = (x – 1)(x² – x – 20)
f(x) = (x – 1)(x – 5)(x + 4)
Then the graph of the function passes through x-axis at –4, 1, and 5.
The graph is shown below.
More about the factorization link is given below.
https://brainly.com/question/6810544

I wanna make sure this is right pls help

Answers
Answer:
C
Step-by-step explanation:
Set it up like a formula
feet = inches/feet = cm/inches
PLEASE HELP!!!!!!!!!!!!

Answers
Answer:
the school block me
Step-by-step explanation:
from seinge this image
Match the equation to the correct description of the transformed function from the
parent function.

Answers
The correct translation for each function is given as follows:
2. Transformation 5 units right and 9 units down.4. Transformation 5 units right and 9 units up.3. Transformation 5 units left and 9 units up.1. Transformation 5 units left and 9 units down.What is a translation?A translation happens when either a figure or a function is moved horizontally or vertically on the coordinate plane.
The four translation rules for functions are defined as follows:
Translation left a units: f(x + a).Translation right a units: f(x - a).Translation up a units: f(x) + a.Translation down a units: f(x) - a.More can be learned about translations at brainly.com/question/28174785
#SPJ1
Math work...HELPPPP!

Answers
1/8 unit- 3
1/8 of 24 = 3
could someone please assist me

Answers
Answer:
See Below.
Step-by-step explanation:
We are given that:
\(\displaystyle \cos(A - B) = \cos A\cos B + \sin A \sin B\)
Part 5.2.1
Note that:
\(\displaystyle \cos ( A + B) = \cos (A - (-B))\)
Then from the given identity:
\(\displaystyle \cos(A - (-B)) = \cos A \cos( -B) + \sin A \sin (-B)\)
Cosine is an even function and sine is an odd function. That is:
\(\displaystyle \cos(- \theta) = \cos \theta \text{ and } \sin (-\theta) = -\sin\theta\)
Hence:
\(\displaystyle \cos(A + B) = \cos A \cos B - \sin A \sin B\)
Part 5.2.2
We want to verify that:
\(\displaystyle \cos^2 (A - B) = 4\cos^2 B \sin^2 B\)
From the identity:
\(\displaystyle \cos (A - B) = \cos A \cos B + \sin A \sin B\)
Since A + B = 90°, A = 90° - B. Hence:
\(\displaystyle \cos (A - B) = \cos (90^\circ - B) \cos B + \sin (90^\circ - B) \sin B\)
Sine and cosine are co-functions. That is:
\(\displaystyle \sin \theta = \cos (90^\circ - \theta) \text{ and } \cos\theta = \sin(90^\circ - \theta)\)
Hence:
\(\displaystyle \cos (A - B) = \sin B\cos B + \sin B \cos B = 2\sin B \cos B\)
And by squaring both sides:
\(\displaystyle \cos ^2 (A - B) = 4\sin^2 B\cos^2 B\)
Use the given scale factor and the side lengths of the scale drawing to determine the side lengths of the real object scale factor 3:1
Answers
To determine the side lengths of the real object using a scale factor of 3:1, we need the corresponding side lengths from the scale drawing.
To find the side lengths of the real object, we can multiply the corresponding side lengths of the scale drawing by the scale factor.
For example, if the scale drawing has a side length of 4 units, the corresponding side length of the real object would be 4 * 3 = 12 units.
Similarly, for any other side length in the scale drawing, we can multiply it by 3 to find the corresponding side length in the real object.
It's important to note that the scale factor must be applied consistently to all sides of the object. If one side length is multiplied by the scale factor, all other side lengths should also be multiplied by the same factor to maintain proportionality between the scale drawing and the real object.
By applying the scale factor of 3:1, we can determine the side lengths of the real object based on the given side lengths of the scale drawing.
Learn more about scale factor here:
https://brainly.com/question/29464385
#SPJ11
5 to the power of 2x=20
Answers
The approximate value of x in the equation 5 to the power of 2x=20 is 0.93
Calculating the value of xFrom the question, we have the following parameters that can be used in our computation:
5 to the power of 2x=20
As an expression, we have
5^(2x) = 20
Take the natural logarithm of both sides
so, we have the following representation
2x = ln(20)/ln(5)
Evaluate the quotients
This gives
2x = 1.86
So, we have
x = 0.93
Hence, the value of x is 0.93
Read more about expression at
https://brainly.com/question/15775046
#SPJ1
y=9x+9
what would be the slope of a parallel line?
Answers
Parallel lines have the same slope.
The slope of y = 9x + 9 is 9, because of the 9 in 9x.
The slope of a parallel line would also be 9.
Workers at a quarry are removing rock at a daily rate of 0.1%. There are 3.2 tons of rock when the work begins.
Which function can be used to find the number of tons of rock left at the end of d days?
A.m(d) = 3.2(0.999)^d
B.m(d) = 3.2(0.99)^d
C.m(d) = 3.2(1.01)^d
D.m(d) = 3.2(1.001)^d
Answers
Answer:c
Step-by-step explanation:
Answer:
The Answer is m(d) = 3.2(0.999)^d
Step-by-step explanation:
Help HelpHelp Help
Help Help
Help Help
Help Help
Help Help
Help Help

Answers
There are five possibilities for whole numbers such that radical expression 4 ≤ √3 + √x ≤ 5: {6, 7, 8, 9, 10}
How to derive an integer for a radical expression
In this problem we have the case of a radical expression, from which we need to determine at least a whole number such that following inequality is fulfilled:
4 ≤ √3 + √x ≤ 5
This can be done by algebra properties. First, determine the limits of the inequality:
4 ≤ √3 + √x
4 - √3 ≤ √x
(4 - √3)² ≤ x
x ≥ (4 - √3)²
x ≥ 16 - 8√3 + 3
x ≥ 19 - 8√3
√3 + √x ≤ 5
√x ≤ 5 - √3
x ≤ (5 - √3)²
x ≤ 25 - 10√3 + 3
x ≤ 28 - 10√3
There are five possibilities for five whole numbers: 6, 7, 8, 9, 10
To learn more on radical expressions: https://brainly.com/question/27847549
#SPJ1
Rita plans to make a call using a calling card. For each call, rita has two options: 1. Pay $0. 49, plus an additional $0. 019 per minute. 2. Pay $0. 059 per minute. She predicts that her call will be x minutes long. Which inequality represents the statement, "rita would save money using the second option"?.
Answers
The inequality that represents Rita saving money from the second option is 0.059x < 0.49 + 0.019x
"Information available from the question"
In the question:
1. Pay $0. 49, plus an additional $0. 019 per minute.
2. Pay $0. 059 per minute.
Now, According to the question:
How to determine the inequality that represents the statement?
We have the following parameters that can be used in our computation:
1. Pay $0.49, plus an additional $0.019 per minute.
2. Pay $0.059 per minute.
These statements can be represented as
Option 1: y = 0.49 + 0.019x
Option 2: y = 0.059x
Where y is the total amount and x is the number of minutes
When she saves money, we have
Option 2 < Option 1
Substitute the known values in the above equation, so, we have the following representation
0.059x < 0.49 + 0.019x.
Learn more about Inequality at:
https://brainly.com/question/27989101
#SPJ4
The mechanic averages 15 miles per hour for the round trip. How long is the mechanic away from the shop
Answers
The time the mechanic is away from the shop is 2 hours.
To find the time the mechanic is away from the shop, we need to know either the distance of the round trip or the speed of the mechanic on one of the legs of the trip.
For example, if we know that the distance of the round trip is 30 miles, we can use the formula:
time = distance / speed
where speed is the average speed for the round trip, which is given as 15 miles per hour.
So, the time the mechanic is away from the shop would be:
time = 30 miles / 15 miles per hour = 2 hours
But without knowing the distance of the round trip or the speed of the mechanic on one of the legs of the trip, we cannot calculate the time the mechanic is away from the shop.
for such more question on distance
https://brainly.com/question/12356021
#SPJ11