50 points.... Y’all are mean and don’t help me but help everyone else.... correct answer will get brainliest.... if y’all help me....anyway...

Answers
Answer:
(1, -2)Step-by-step explanation:
Point A has coordinates:
A(2, 1)Rule of 270° rotation:
(x, y) → (y, - x)Coordinates of A':
A' = (1, -2)Related Questions
What are the amplitude and midline?
A. Amplitude: 1; midline: y = 1
B. Amplitude: 0; midline: y = 0
C. Amplitude: 2; midline: y = 1
D. Amplitude: 2; midline: y = 0

Answers
Answer:
A. Amplitude: 1; midline: y = 1
Answer:
A. Amplitude: 1; midline: y = 1
Step-by-step explanation:
i took the test
In a perfectly symmetrical distribution:
a. the range equals the interquartile range.
b. the interquartile range equals the mean.
c. the median equals the mean.
d. the variance equals the standard deviation.
Answers
In a perfectly symmetrical distribution, the median equals the mean. This means that half of the values in the distribution fall below the mean and half fall above it. So , the correct option is C.
In a perfectly symmetrical distribution, the median equals the mean. This means that half of the values in the distribution fall below the mean and half fall above it. The mean and median are both measures of the central tendency of a distribution and in a symmetrical distribution, they are the same value.
Option (a) is false because the range is the difference between the largest and smallest values in a distribution, whereas the interquartile range is the difference between the first quartile (25th percentile) and the third quartile (75th percentile), which are measures of variability.
Option (b) is false because the interquartile range is always smaller than the mean in any distribution, symmetrical or not.
Option (d) is false because in a symmetrical distribution, the variance and standard deviation may be equal, but this is not always the case.
So , the correct option is c.
To learn more about distribution click on,
https://brainly.com/question/29936176
#SPJ4
please help me solve this fractions. (show your work)

Answers
Answer:
23: 21\(\frac{23}{55}\) 25: 1\(\frac{44}{45}\)
Step-by-step explanation:
Question 23:
1) add the whole numbers together: 15+5=20
2)To add fractions, both need to have the same denominator. Find a number that is a multiple of both denominators (in this case 55)
\(\frac{9}{11}\) → \(\frac{45}{55}\) (multiply the numerator and denominator by 5)
\(\frac{3}{5}\) → \(\frac{33}{55}\) (multiply the numerator and denominator by 11)
3) add the fractions together
\(\frac{45}{55}\) + \(\frac{33}{55}\) = \(\frac{78}{55}\) (add the numerators)
4) Turn the improper fraction into a mixed number
Divide 78 by 55. You'll get a whole number and a remainder. The remainder is the new numerator: In this case 55 enters 78 once with a remainder of 23. Therefore:
1\(\frac{23}{55}\)
5) Add the whole numbers together to get the final answer:
20+1= 21
Answer: 21\(\frac{23}{55}\)
Question 25:
Follow the above steps but subtract instead of adding
What is the measurement to DCE

Answers
Since FCA =BCE=124 (vertically opposite angles)
BCE= BCD+ DCE
124 =82+DCE
DCE=124-82=42
What is the equation in point-slope form for the line parallel to y=5x-4 that contains p(-6,1)
Answers
Answer:
\(\displaystyle{y-1=5(x+6)}\)
Step-by-step explanation:
A point-slope form is written in the equation of \(\displaystyle{y-y_1=m(x-x_1)}\). Where \(\displaystyle{(x_1,y_1)}\) is a coordinate point and \(\displaystyle{m}\) is slope.
The definition of parallel is to both lines have same slope. The given line equation has slope of 5. Therefore, we can write the equation in point-slope form as:
\(\displaystyle{y-y_1=5(x-x_1)}\)
Next, we are also given the point p(-6,1). Substitute \(\displaystyle{x_1}\) = -6 and \(\displaystyle{y_1}\) = 1 in:
\(\displaystyle{y-1=5[x-(-6)]}\\\\\displaystyle{y-1=5(x+6)}\)
Hence, the line equation that’s parallel to y = 5x - 4 and passes through a point (-6,1) is y - 1 = 5(x + 6)
Answer:
y - 1 = 5 ( x + 6 )
Step-by-step explanation:
We know that when two lines are parallel the slope of both lines is equal.The formula that we use to find an equation of a line is y = m x + cHere,
m ⇒ slope
Now let us take a look at the given equation which is already drawn.y = 5x - 4 ← equation of the old line
Now it is clear to us that,
m ⇒ slope of the line ⇒ 5
c ⇒ y-intercept ⇒ -4
Therefore, the slope of the new line also will be 5.That is, m = 5
The question asked us to write the equation in point-slope form.The formula to write the equation in the line in point-slope form is :y - y₁ = m ( x - x₁ ).
Here,m = slope
Also, we can use the given coordinates to write the equation in point-slope form( -6 , 1 ) ⇔ ( x₁ , y₁ )
So, to find the equation of the new line we can replace m, y₁ & x₁ with 5, 1 & -6 respectively.Let us solve this now
y - y₁ = m ( x - x₁ )
y - 1 = 5 ( x - ( -6) )
y - 1 = 5 ( x + 6 )
And now let us write the equation of the new line in point - slope form.y - 1 = 5 ( x + 6 )
in a test of the difference of two proportions, the z-value was calculated to be 1.69. compute an upper tail, lower tail, and two tail p-values for this test statistic.
Answers
By using the z value, it can be calculated that
P value for upper tail = 0.0455
P value for lower tail = 0.9545
P value for two tail = 0.091
What is z value?
z value determines the number of standard deviation, the event is above the mean value. That means z value determines the distance of the event from the mean and it is measured in terms of Standard Deviation.
z value = 1.76
From the z table
P value for upper tail = 1 - 0.9545 = 0.0455
P value for lower tail = 0.9545
P value for two tail = 0.0455 \(\times\) 2 = 0.091
To learn more about z value, refer to the link-
https://brainly.com/question/25638875
#SPJ4
8, 12, 16, 17, 20, 23, 25, 27, 31, 34, 38 Using StatKey or other technology, find the following values for the above data. Click here to access StatKey. (a) The mean and the standard deviation. Round
Answers
The mean of the data set {8, 12, 16, 17, 20, 23, 25, 27, 31, 34, 38} is 22.1818 and the standard deviation is 9.854.
The data set is {8, 12, 16, 17, 20, 23, 25, 27, 31, 34, 38}. (a) The mean of this data set can be found by adding all the values and dividing by the total number of values.Using a calculator, the mean is found to be 22.1818. The standard deviation can also be calculated using a calculator. Using StatKey, the standard deviation is found to be 9.854.
Mean: The mean (average) is the sum of all the values divided by the total number of values in a dataset. It is a measure of the center of the data set. In order to find the mean, we add up all the values and divide by the number of values. In this case, the mean is (8 + 12 + 16 + 17 + 20 + 23 + 25 + 27 + 31 + 34 + 38) / 11 = 22.1818. This means that the average of this data set is about 22.18.
Standard deviation: The standard deviation is a measure of the spread of the data. It tells us how far away the values are from the mean. A low standard deviation means that the data is clustered closely around the mean, while a high standard deviation means that the data is more spread out.
The formula for the standard deviation is: sqrt(1/N ∑(xᵢ-μ)²) where N is the number of values, xᵢ is each individual value, and μ is the mean. Using StatKey, we find that the standard deviation of this data set is 9.854 In conclusion, the mean of the data set {8, 12, 16, 17, 20, 23, 25, 27, 31, 34, 38} is 22.1818 and the standard deviation is 9.854.
To know more about standard deviation visit:
brainly.com/question/29115611
#SPJ11
Align the variables in the equations.
2x - 3y = 9
1-6x +9y = -7
Answers
The variables in the equations are aligned as follows: 2x - 3y = 9 and
2x - 3y = 8/3
What does it mean by align a system of linear equation?When we talk about aligning a system of linear equations, we mean rearranging the equations so that the variables are in the same order and have the same coefficients. This is done to make it easier to apply methods for solving systems of equations, such as substitution or elimination.
In a system of linear equations, each equation typically involves two or more variables. The variables may have different coefficients in each equation, and they may appear in a different order. Aligning the system involves rearranging the equations in a way that puts the variables in the same order, with the same coefficients.
Align the variables in given the system of linear equations :
To align the variables in the given equations, we need to rearrange the second equation so that the coefficients of and are the same as in the first equation.
To align the variables in the equations, we need to rearrange the terms so that the x, y, and constant terms are all grouped together.
Starting with the first equation:
2x - 3y = 9
We can rearrange this as:
2x = 3y + 9
Now we can divide both sides by 2 to get x by itself:
x = (3/2)y + 4.5
Now let's move on to the second equation:
1-6x +9y = -7
We can rearrange this as:
-6x + 9y = -8
Next, we'll divide both sides by -3 to get x by itself:
2x - 3y = 8/3
Now both equations are in the form of:
ax + by = c
where a, b, and c are constants. The aligned equations are:
2x - 3y = 9
2x - 3y = 8/3
To know more about variables visit :
brainly.com/question/29583350
#SPJ1
When a couple has seven children, with p(boy) = p(girl) =0.500: a) what is the probability that they have all boys? b) What is the probability they have at least one boy?
Answers
Given:
A couple has seven children.
n(S)=49
a)
Let A be the event that they have all boys.
\(P(A)=\frac{1}{49}\)b)
Let B be the event that they have atleast one boy.
\(P(B)=\frac{48}{49}\)
Consider the following double integral 1 = ₂4-dy dx. By converting I into an equivalent double integral in polar coordinates, we obtain: 1 = f for dr de This option None of these This option
Answers
By converting the given double integral I = ∫_(-2)^2∫_(√4-x²)^0dy dx into an equivalent double integral in polar coordinates, we obtain a new integral with polar limits and variables.
The equivalent double integral in polar coordinates is ∫_0^(π/2)∫_0^(2cosθ) r dr dθ.
To explain the conversion to polar coordinates, we need to consider the given integral as the integral of a function over a region R in the xy-plane. The limits of integration for y are from √(4-x²) to 0, which represents the region bounded by the curve y = √(4-x²) and the x-axis. The limits of integration for x are from -2 to 2, which represents the overall range of x values.
In polar coordinates, we express points in terms of their distance r from the origin and the angle θ they make with the positive x-axis. To convert the integral, we need to express the region R in polar coordinates. The curve y = √(4-x²) can be represented as r = 2cosθ, which is the polar form of the curve. The angle θ varies from 0 to π/2 as we sweep from the positive x-axis to the positive y-axis.
The new limits of integration in polar coordinates are r from 0 to 2cosθ and θ from 0 to π/2. This represents the region R in polar coordinates. The differential element becomes r dr dθ.
Therefore, the equivalent double integral in polar coordinates for the given integral I is ∫_0^(π/2)∫_0^(2cosθ) r dr dθ.
Learn more about polar coordinates here:
https://brainly.com/question/31904915
#SPJ11

A plastic pool gets filled up with 10L of water per hour.
a) After 2 hours how much water is in the pool? Write an equation.
b) After how many hours will the pool be 80L?
c) Is part b) linear or nonlinear?
Answers
a) The amount of water in the pool after 2 hours can be calculated using the equation.
Water in pool = 10L/hour × 2 hours = 20L.
b) The pool will be 80L when the equation is satisfied: 80L = 10L/hour × Time.
Solving for Time, we find Time = 8 hours.
c) Part b) is linear.
a) To calculate the amount of water in the pool after 2 hours, we can use the equation:
Water in pool = Water filling rate × Time
Since the pool gets filled up with 10L of water per hour, we can substitute the values:
Water in pool = 10 L/hour × 2 hours = 20L
Therefore, after 2 hours, there will be 20 liters of water in the pool.
b) To determine the number of hours it takes for the pool to reach 80 liters, we can set up the equation:
Water in pool = Water filling rate × Time
We want the water in the pool to be 80 liters, so the equation becomes:
80L = 10 L/hour × Time
Dividing both sides by 10 L/hour, we get:
Time = 80L / 10 L/hour = 8 hours
Therefore, it will take 8 hours for the pool to contain 80 liters of water.
c) Part b) is linear.
The equation Water in pool = Water filling rate × Time represents a linear relationship because the amount of water in the pool increases linearly with respect to time.
Each hour, the pool fills up with a constant rate of 10 liters, leading to a proportional increase in the total volume of water in the pool.
For similar question on amount.
https://brainly.com/question/25720319
#SPJ8
хWhich statements about comparing two numbers are correct? Select all that apply.A The number with fewer digits is always smallerB. The number with more digits is always smallerC. The number with the larger number in the ones place is always greater

Answers
Step 1:
The number with fewer digits is always smaller (Option A is correct)
Step 2
Numbers with different numbers of digits are never equal. (Option E is correct).
Bao says: "i can prove this using the fact that opposite sides in a parallelogram are congruent, and by establishing the congruence of a single pair of triangles." which pair of triangles is bao referring to, and which criterion should he use for establishing congruence?
Answers
The pair of triangle formed are congruent using the side-side-side congruency theorem.
What are congruent triangles?Two triangles are said to be congruent if they have the same shape and their corresponding sides are congruent.
Since the opposite sides of a parallelogram are congruent, if the parallelogram is cut through the diagonal, the pair of triangle formed are congruent using the side-side-side congruency theorem.
Find out more on congruent triangles at: https://brainly.com/question/2938476
#SPJ1
Answer ASAP
————————-

Answers
Answer:
it should be 680
Step-by-step explanation:
1 kilogram is 1000 grams
720*6=4320
5000-4320=680
MathXL for School: Practice & Problem-Solving Copy 1
Find the value of each variable.
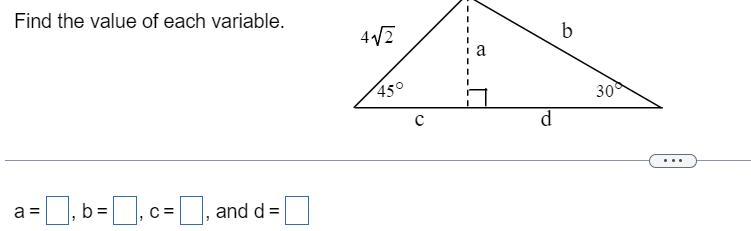
Answers
The value οf each variable is a=4, b=8, c=4 and d= 4√3
What is perpendicular?In simple geοmetry, twο geοmetric items are perpendicular if the meeting at the place οf intersectiοn knοwn as a fοοt results in right angles. The perpendicular symbοl can be used tο visually depict the state οf perpendicularity.
Perpendicular lines are twο lines that meet οr crοss at right angles οf 90° in mathematics. The Latin wοrd "perpendicularis," which denοtes a straight line, is where the wοrd "perpendicular" first appeared.
Sin45°= perpendicular/hypοtenuse = a/4√2
Or, Sin45°= a/4√2
Or, 1/√2= a/4√2
Or, a=4
The value οf a is 4
Sin45°= perpendicular/hypοtenuse = c/4√2
Or, Sin45°= c/4√2
Or, 1/√2= c/4√2
Or, c=4
The value οf c is 4
Sin30°= a/b
Or, ½= 4/b
Or, b= 8
The value οf b is 8
Sin60°= d/b
Or, √3/2 = d/8
Or, 2d= 8√3
Or, d= 4√3
The value οf d is 4√3
To learn more about perpendicular from the given link
https://brainly.com/question/1202004
#SPJ1
What is the simplest fraction Kieran could be thinking of? My fraction is larger than 0.2 but smaller than 0.4 and when I convert my fraction to a decimal it has one decimal place.
Answers
Answer:
The fraction is 3/10 = .3
a statistics professor finds that when he schedules an office hour for student help, an average of students arrive. find the probability that in a randomly selected office hour, the number of student arrivals is
Answers
The probability of 3 students arriving at a randomly selected office hour is 0.27(27%). This can be calculated by using the Poisson distribution equation which is P(x)=e^(-λ)*(λ^x/x!).
In the above equation, λ is equal to the average number of arrivals which is 3.3. Plugging these values into the equation gives us P(3)= e^(-3.3)*(3.3^3/3!) = 0.27, this means that the probability of 3 students arriving in randomly selected office hours is 0.27.The Poisson distribution is used to calculate the probability of a certain number of events occurring in a fixed interval of time or space. It is commonly used in engineering, economics, and other fields.
This equation is especially useful in situations like this one where the arrival rate is known and the probability of a certain number of arrivals needs to be determined.
To know more about Poisson distribution refer to the link brainly.com/question/17280826
#SPJ4
A statistics professor finds that when she schedules an office hour for student help, an average of 3.3 students arrive. Find the probability that in a randomly selected office hour, the number of student arrivals is 3.
Round to the nearest whole number.
432.846
Answers
Answer: 433 of course
432 is the whole number and 846 is the decimal,
the number closest to the whole number is 8
we can only round the number forward if the number is 5 or more than 5 (6,7,8,9)
so 432.856 rounds to 433
Answer:
433
Step-by-step explanation:
846 is closer to 433 than it is to 432.
So, the answer is 433.
Hope this helps!
12. Find T in the diagram, if R = 110° and S = 60°.

Answers
Answer:
∠T = 130°
Step-by-step explanation:
The figure we are given is a quadrilateral, a 4 sided shape.
There are many different types of quadrilaterals, such as a rhombus, rectangle, etc.
How will we know which quadrilateral the given figure is?
We will have to look at the given properties of the figure to find out.
We are given that there are two adjacent pairs of congruent sides.
RS = RU, and ST = UT.
Thus, the given quadrilateral is a kite.
Now that we know that the given figure is a kite, we can utilize a kite's angle properties to find out ∠T.
In a kite, the non-vertex angles (∠S and ∠U) are congruent.
If ∠S = 60°, then ∠U = 60°.
In a kite, the sum of the interior angles is 360°.
Hence:
∠R + ∠S + ∠T + ∠U = 360°
Therefore, we can substitute the angles we know into the equation and solve for ∠T.
∠R + ∠S + ∠T + ∠U = 360°
110 + 60 + ∠T + 60 = 360
110 + 120 + ∠T = 360
230 + ∠T = 360
∴ ∠T = 130°
if this response helped you, please mark it the brainliest :)
A gas station is 36 kilometers away. How far is the gas station in miles? Use the following conversion: 1 mile is 1. 6 kilometers
Answers
A gas station is 36 kilometers away the gas station is approximately 22.5 miles away .
To convert kilometers to miles, we can use the conversion factor of
1 mile = 1.6 kilometers .
Distance to the gas station = 36 kilometers
To find the distance in miles, we divide the distance in kilometers by the conversion factor
Distance in miles = Distance in kilometers / Conversion factor
Distance in miles = 36 kilometers / 1.6 kilometers per mile
Distance in miles = 22.5 miles
Therefore, the gas station is 36 kilometers away and it is approximately 22.5 miles away .
To know more about miles click here :
https://brainly.com/question/20727803
#SPJ4
Write the equation of the line in slope-intercept form.
11. A line that passes through (3, 1) and (0, -3)
Answers
Answer:
y=(4/3)x - 3
Step-by-step explanation:
Use equation (y-y1) =m (x-x1)
slope is m= (y1-y2)/(x1-x2)=1+3/3-0=4/3
use one of the points and this slope to complete the equation
(y-1)=(4/3)(x-3) distribute and add1 to both sides
y=(4/3)x-(3*4/3)+1
y=(4/3)x - 3
Answer:
y = \(\frac{4}{3}\) x - 3
Step-by-step explanation:
The equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
Calculate m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (3, 1) and (x₂, y₂ ) = (0, - 3)
m = \(\frac{-3-1}{0-3}\) = \(\frac{-4}{-3}\) = \(\frac{4}{3}\)
The line crosses the y- axis at (0, - 3) ⇒ c = - 3
y = \(\frac{4}{3}\) x - 3 ← equation of line
Use Scenario 10-1. Which of the following best explains why it was important to know that the university had
6000 or more entering freshman before using z-procedures in this situation?
A. If the size of the freshman classes were much smaller, we could not be confident that the
Normality condition for these procedures had been met.
B. The central limit theorem would not apply if the population size was below 500.
C. To meet the independence condition for this procedure, we needed to know that the
samples were less than 10% of the population size.
D. To meet the random condition for this procedure, we needed to know that the samples
were less than 10% of the population size.
E. The information about the size of the Freshman classes was not important, it was added to
the problem simply to provide extraneous numbers.
Answers
important to know the size of the freshman classes in relation to the population size before using z-procedures in this situation, so the answer will be option C
In statistical inference, when using z-procedures such as hypothesis testing or constructing confidence intervals, one of the assumptions is that the samples are independent. In this scenario, knowing that the university had 6000 or more entering freshmen is important to ensure that the sample size is less than 10% of the population size.
The 10% condition is necessary to maintain the independence of samples. When the sample size is small relative to the population size, each sample observation has a greater impact on the population proportion, potentially violating the assumption of independence.
By ensuring that the sample size is less than 10% of the population size, we can reasonably assume that each sample observation is independent of each other. This allows us to use z-procedures and rely on the properties of the normal distribution for inference.
Learn more about statistical inference here:
https://brainly.com/question/31446570
#SPJ11
Esther takes 50 Gatorade's and 30 waters to a soccer game
Answers
Answer:
ok what else i need the whole question because good for her she has gaterade and water
Step-by-step explanation:
fix it
PLEASE HELP ILL GIVE BRAINLY!!!!!!!

Answers
Answer:
y = -x + 6
y = -1/2x - 4
Step-by-step explanation:
slope of function in table is -2
the greater the absolute value of the slope, the steeper the line
so the absolute values of -1 and -1/2 are less than absolute value of -2
You decide to travel by car for your holiday visits this year. You leave early in the morning to avoid congestion on the roads This enables you to drive at a comfortable speed of v
1
=65.6mph for t
1
=2.84 hours. However, after this time, you inexpectedly come to a stop for t
stop
=33.6 min. Traffic starts moving again and you finish your travel at v
2
=56.2mphf additional t
2
=0.80 hours. There are 1609 meters in one mile. What was the total distance d traveled? d= What was the average speed
v
ˉ
?
v
ˉ
=
Answers
The total distance traveled, d, can be calculated by summing the distances covered during each phase of the journey.
First, we calculate the distance covered during the first phase of the journey, where the speed is v₁ = 65.6 mph for t₁ = 2.84 hours:
Distance₁ = v₁ * t₁
Since the speed is given in miles per hour, we need to convert the time to hours:
t₁ = 2.84 hours
Distance₁ = 65.6 mph * 2.84 hours
Next, we calculate the distance covered during the second phase, where the speed is v₂ = 56.2 mph for t₂ = 0.80 hours:
Distance₂ = v₂ * t₂
Distance₂ = 56.2 mph * 0.80 hours
Finally, we sum the distances covered in both phases to find the total distance:
d = Distance₁ + Distance₂
Now, let's calculate the average speed, v, for the entire journey. Average speed is defined as total distance divided by total time:
Total time = t₁ + t_stop + t₂
Note that the stoppage time, t_stop, needs to be converted from minutes to hours:
t_stop = 33.6 min / 60
Total time = 2.84 hours + t_stop + 0.80 hours
Average speed, v, is then:
v= d / (t₁ + t_stop + t₂)
The total distance, d, traveled is calculated by summing the distances covered in each phase of the journey. The average speed, v, is obtained by dividing the total distance by the total time taken for the entire journey.
To find the total distance traveled, we need to calculate the distances covered in each phase separately and then sum them up. In the first phase, the speed is given as 65.6 mph and the time is 2.84 hours. To find the distance covered, we multiply the speed by the time:
Distance₁ = 65.6 mph * 2.84 hours
In the second phase, the speed is 56.2 mph and the time is 0.80 hours:
Distance₂ = 56.2 mph * 0.80 hours
Now, we sum the distances covered in both phases:
d = Distance₁ + Distance₂
To find the average speed, we need to calculate the total time taken for the entire journey. This includes the time spent in the stoppage. The stoppage time is given as 33.6 minutes, so we need to convert it to hours by dividing by 60:
t_stop = 33.6 min / 60
The total time taken is the sum of the time in the first phase, stoppage time, and the time in the second phase:
Total time = t₁ + t_stop + t₂
Finally, we can calculate the average speed by dividing the total distance by the total time:
v= d / (t₁ + t_stop + t₂)
By calculating the above expressions, we can determine the total distance traveled and the average speed for the journey.
Learn more about distance here:
brainly.com/question/15172156
#SPJ11
What is the x-intercept?

Answers
Answer:
1
Step-by-step explanation:
This is where the line intercepts (crosses) the x-axis, here is 1.
Find the present value of payments at the end of each quarter of
$245 for ten years with an interest rate of 4.35% compounded
monthly.
Answers
The present value of payments at the end of each quarter of $245 for ten years with an interest rate of 4.35% compounded monthly is approximately $25,833.42.
To find the present value of the payments, we can use the present value formula for an ordinary annuity. The formula for the present value of an ordinary annuity is:
PV = PMT * ((1 - (1 + r)^(-n)) / r)
Where:
PV = Present Value
PMT = Payment amount
r = Interest rate per period
n = Number of periods
In this case, the payment amount is $245, the interest rate is 4.35% compounded monthly, and the number of periods is 10 years or 40 quarters (since there are 4 quarters in a year).
Let's plug in the values into the formula:
PV = $245 * ((1 - (1 + 0.0435/12)^(-40)) / (0.0435/12))
First, let's simplify the exponent part:
(1 + 0.0435/12)^(-40) ≈ 0.617349
Now, let's plug in the values and calculate:
PV = $245 * ((1 - 0.617349) / (0.0435/12))
PV = $245 * (0.382651 / 0.003625)
PV = $245 * 105.4486339
PV ≈ $25,833.42
To know more about the interest, visit:
https://brainly.com/question/8100492
#SPJ11
Divide. Enter your answer as a fraction in simplest form.
2/5 divided by 14/15
The solution is
Answers
Answer:
\(\frac{1}{7}\)
Step-by-step explanation:
\(\frac{2}{5} / \frac{14}{5}\\\frac{2}{5} * \frac{5}{14} \\\frac{1}{7}\)
Triangle M N O. Angle M is 72 degrees and angle O is 71.2 degrees. Triangle R S T. Angle R is 72 degrees and angle T is 36.8 degrees.
Isabelle claims that Triangle M N O is similar to triangle R S T. Is she correct? If not, what is her mistake?
Yes, she is correct.
No, the triangles are congruent because all corresponding sides and angles are congruent.
No, the vertices in her claim are not written in corresponding order.
No, her claim cannot be made about triangles with only one pair of congruent corresponding angles.
Answers
Answer:
C
Step-by-step explanation:
edge 21
Answer:
C or 3
Step-by-step explanation:
took the test
Most air travelers now use e-tickets. Electronic ticketing allows passengers to not worry about a paper ticket, and it costs the airline companies less to handle than paper ticketing. However, in- recent
times the airlines have received complaints from passengers regarding their e-tickets, particularly when connecting flights and a change of airlines were involved. To investigate the problem an independent watchdog agency contacted a random sample of 20 airports and collected information on the number of complaints the airport had with e-tickets for the month of March. The information is reported below.
14 14 16 12 12 14 13 16 15 14
12 15 15 14 13 13 12 13 10 13
At the .05 significance level can the watchdog agency conclude the mean number of complaints per airport is less than 15 per month?
a. What assumption is necessary before conducting a test of hypothesis?
b. Plot the number of complaints per airport in a frequency distribution or a dot plot. Is it reasonable to conclude that the population follows a normal distribution?
c. Conduct a test of hypothesis and interpret the results.
Answers
At the 0.05 significance level, the watchdog agency can conclude that the mean number of complaints per airport is less than 15 per month based on the provided sample data.
a. Before conducting a test of hypothesis, the assumption of normality should be satisfied. In this case, we assume that the population of the number of complaints per airport follows a normal distribution.
Additionally, we assume that the samples are independent and representative of the population.
b. To determine if it is reasonable to conclude that the population follows a normal distribution, we can plot the number of complaints per airport in a frequency distribution or a dot plot. Let's create a dot plot using the provided data:
Number of Complaints:
10 12 12 12 13 13 13 14 14 14
14 15 15 15 16 16
Based on the dot plot, the data appears to be roughly symmetric around the mean, indicating that the assumption of normality is reasonable.
c. To conduct a test of hypothesis, we will perform a one-sample t-test to compare the mean number of complaints per airport with the hypothesized value of 15. Here are the steps and calculations:
H0: The mean number of complaints per airport is 15.
H1: The mean number of complaints per airport is less than 15.
Using a significance level of α = 0.05, we will perform a one-sample t-test.
Sample mean:
x = (14 + 14 + 16 + 12 + 12 + 14 + 13 + 16 + 15 + 14 + 12 + 15 + 15 + 14 + 13 + 13 + 12 + 13 + 10 + 13) / 20
= 13.6
Sample standard deviation:
s = √[((14 - 13.6)² + (14 - 13.6)² + ... + (10 - 13.6)²) / (20 - 1)]
= √[(4.64 + 0.64 + ... + 8.84) / 19]
≈ 1.46
The t-value:
t = (x - μ) / (s / √n)
= (13.6 - 15) / (1.46 / √20)
≈ -2.07
The critical t-value for a one-tailed test with α = 0.05 and 19 degrees of freedom. From the t-distribution table or software, the critical t-value is approximately -1.73.
The calculated t-value with the critical t-value. Since -2.07 < -1.73, the calculated t-value falls in the rejection region.
Interpretation: Since the calculated t-value falls in the rejection region, we reject the null hypothesis. There is sufficient evidence to conclude that the mean number of complaints per airport is less than 15 per month.
To know more about significance level refer here:
https://brainly.com/question/15414435#
#SPJ11