A self-serve frozen yogurt store sells servings up to 12 ounces. It charges $0.50 per ounce for a serving between 0 and 8 ounces, and $4 for any serving greater than 8 ounces and up to 12 ounces.
Build the function that models the relationship between the price p(w) and of the weight w of a serving of yogurt.
Answers
Answer:
p(w) - - - - - - p = 0.5w ; for 0 < w ≤ 8
p(w) - - - - - - p = 4 ; for w < 8 and w ≤ 12
Step-by-step explanation:
Price, p of weight of a toughurt serving :
p(w) - - - - - - p = 0.5w ; for 0 < w ≤ 8
p(w) - - - - - - p = 4 ; for w < 8 and w ≤ 12
The function that models the relationship is:
p(w) = 0.50w for 0 < w ≤ 8
p(w) = 4w for 8 < w ≤ 12
The self-serve frozen yoghurt store charges:
$0.50 per serving for a serving between 0 and 8 ounces
$4 per serving for any serving greater than 8 ounces and up to 12 ounces.
Let the weight of servings be represented by w
Let the price as a function of the weight be p(w)
For a charge of $0.50 per serving:
p(w) = 0.50w, where 0 < w ≤ 8
For a charge of $4 per serving:
p(w) = 4w, where 8 < w ≤ 12
Therefore, the function that models the relationship is:
p(w) = 0.50w for 0 < w ≤ 8
p(w) = 4w for 8 < w ≤ 12
Learn more on functions here: https://brainly.com/question/10439235
Related Questions
Item 4
What is the equation of this line written in slope-intercept form?
y−52=−2(x+1)
1.) y=−2x−92
2.) y=−2x+12
3.) y=−2x+72
4.) I don't know.
Answers
Answer: Option 2 is correct Y= -2x+1/2
Step-by-step explanation:

The solution is Option 2.
The equation of line in the slope intercept form is y = -2x + 1/2
where m is the slope m = -2 and the y intercept is 1/2
What is an Equation of a line?
The equation of a line is expressed as y = mx + b where m is the slope and b is the y-intercept
And y - y₁ = m ( x - x₁ )
y = y-coordinate of second point
y₁ = y-coordinate of point one
m = slope
x = x-coordinate of second point
x₁ = x-coordinate of point one
The slope m = ( y₂ - y₁ ) / ( x₂ - x₁ )
Given data ,
Let the equation of line be represented as A
The value of A is given by
y - 5/2 = -2 ( x + 1 )
Now , the slope intercept of the equation of line is given by
Equation of a line is expressed as y = mx + b where m is the slope and b is the y-intercept
So , on simplifying the equation , we get
y - 5/2 = -2 ( x + 1 )
y - 5/2 = -2x - 2
Adding 5/2 on both sides of the equation , we get
y = -2x - 2 + 5/2
y = -2x + ( -4 + 5 ) / 2
y = -2x + 1/2
The slope of the equation A is m = -2
The y intercept of the equation A is 1/2
Hence , The equation of line in the slope intercept form is y = -2x + 1/2
To learn more about equation of line click :
https://brainly.com/question/14200719
#SPJ2
ill mark brainlist plsshelp

Answers
30(15/18) = y
we then multiply 30*15 = 450
450/18 = y
we then get
y = 25
María y Carlos quieren vender galletas en la feria de la colonia. El costo del material para hacer las galletas es de $530. Ellos pidieron la cooperación de sus vecinos. ¿Cuál es la expresión algebraica que permite conocer la aportación (y) de acuerdo al número de vecinos (x)?
Answers
Answer:
it will be 100
Step-by-step explanation:
8 x 1/2=4 simplified
Answers
Answer:
Step-by-step explanation:
8➗2=4
since 1/2 is divinding
please help im begging you, brainliest to correct answer!!!
(zoomed in equation on slide 2)
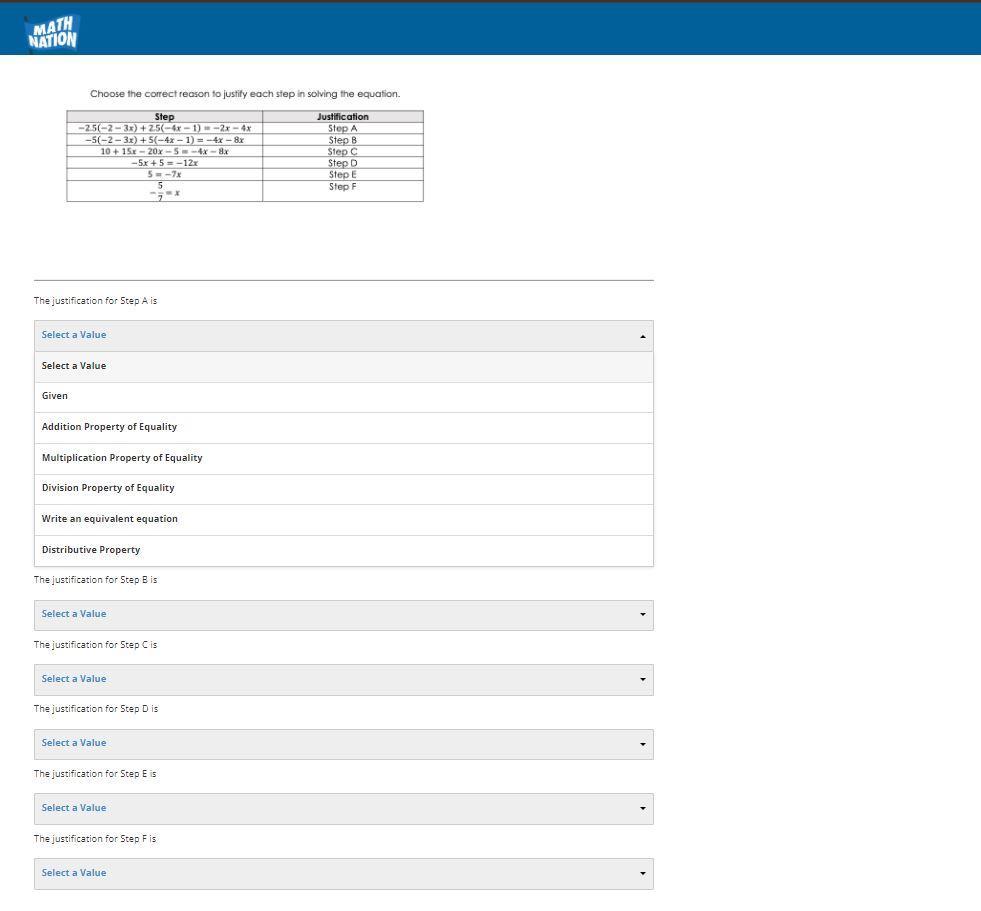

Answers
Step b is multiplication property
Step c is distributive property
Step d is substitution/ write and equivalent expression
Step e is addition property
Step f is division
Find the solution of xay! + 5xy + (4 + 1x)y = 0, x > 0 of the form = oo yı = x" c,x", = n=0 where co 1. Enter r = Cn = , n= 1,2,3,...
Answers
The solution of the equation x^ay! + 5xy + (4 + x)y = 0, where x > 0, can be represented as a power series of the form y = ΣCnx^n, where C0 = 1 and Cn = 0 for n = 1, 2, 3, ...
To find the solution of the equation x^ay! + 5xy + (4 + x)y = 0, we can represent the solution as a power series expansion of the form y = ΣCnx^n, where Cn is the coefficient of x^n and n ranges from 0 to infinity. Plugging the power series into the equation, we get:
x^a*(ΣCnx^nn!) + 5x*(ΣCnx^n) + (4 + x)(ΣCn*x^n) = 0
We can then collect the terms with the same powers of x:
ΣCnx^(n+a)n! + Σ5Cnx^(n+1) + Σ(4 + x)Cnx^n = 0
For the equation to hold true for all powers of x, each term with the same power of x must be zero. Therefore, we can determine the coefficients Cn for each power of x. For n = 0, the term ΣCnx^a0! simplifies to C0x^a0! = C0*x^a. Since the equation must hold for all x > 0, the coefficient C0 must be non-zero. Therefore, C0 = 1. For n = 1, the term Σ5Cnx^2 simplifies to 5C1x^2 = 0. Therefore, C1 = 0. Similarly, for n = 2, 3, 4, ... , the terms involving Cn will also be zero, as they are multiplied by powers of x. Hence, the solution of the equation x^ay! + 5xy + (4 + x)y = 0 can be represented as y = C0x^a = x^a, where a is a positive real number, and the coefficients Cn are zero for n = 1, 2, 3, ....
Learn more about coefficient here: brainly.com/question/1594145
#SPJ11
Chris has scored the following points in his last five basketball games: 13,10,14,9,6
How many points must he have score in the next game to average 14 points per game?
PLEASE HELP! NO FILES THO! THEY DON'T WORK!
Answers
Answer:
he must score 32 points in the next game in order to average 14 points per game.
Step-by-step explanation:
14 x 6 = 84
84-(13+10+14+9+6)=32
checking:
32 + 13 + 10 + 14 + 9 + 6 = 84
84 divided by 6 = 14
Two lines intersect. Find the value of b.
bo
42°
cº

Answers
Step-by-step explanation:
b = 42
c = 138
plz mark my answer as brainlist plzzzz if you find it useful .
the circumference of the base of a 5 -foot-high cylinder is 46 inches. what is the volume of the cylinder? (use the formula v
Answers
The volume of the cylinder is 10,099.09 inches³.
Here we have to find the volume of the cylinder.
Given data:
Circumference = 46 inches
height(h) = 5 foot = 12 × 5 = 60 inches
Circumference = 2πr
46 = 2 ×π × r
r = 23/π
The formula for the volume of the cylinder = πr²h
Volume = π( 23/π)² 60
= 529× 60 / π
= 10,099.09 inches³
Therefore the volume is 10,099.09 inches³.
To know more about the cylinder refer to the link given below:
https://brainly.com/question/23935577
#SPJ4
Help me with this!?!
In order to solve a system by substitution, you want to...
get opposite coefficients for each variable in each equation.
get opposite coefficients for one set of variables in each equation.
isolate a variable in an equation and then substitute into the other equation.
put the corresponding augmented matrix into RREF (row reduced echelon form).
Answers
Answer:
Step-by-step explanation:
1. Solve one of the equations for one of the variables. For example, if the system is:
x + 2y = 7
3x - 4y = -2
2. You could solve the first equation for x by subtracting 2y from both sides:
x = 7 - 2y
3. Substitute the expression for the variable you solved for in step 1 into the other equation. For example, substituting x = 7 - 2y into the second equation gives:
3(7 - 2y) - 4y = -2
4. Simplify and solve for the remaining variable. Using the example from step 2, you would simplify the equation as follows:
21 - 6y - 4y = -2
21 - 10y = -2
-10y = -23
y = 23/10
5. Substitute the value of the variable you found in step 3 back into one of the original equations to solve for the other variable. Using the example from step 1, you would substitute y = 23/10 into x = 7 - 2y:
x = 7 - 2(23/10)
x = -9/5
6. Check your solution by substituting the values you found for x and y into both of the original equations. If the values satisfy both equations, then you have found the correct solution.
x + 2y = 7 becomes (-9/5) + 2(23/10) = 7, which is true
3x - 4y = -2 becomes 3(-9/5) - 4(23/10) = -2, which is also true
Therefore, the solution to the system of equations is x = -9/5 and y = 23/10.
The graphed line can be expressed by which equation?

Answers
Answer:
y = 3/2x + 3/2
Step-by-step explanation:
Slope is rise over run, or 3/2
The y intercept is at 3/2.
y = 3/2x + 3/2
4-101. Copy and simplify each expression. Homework Help ✎ a. −2 + 5 _____________________________________
b. (−4) · (6) _____________________________________
c. 4 − (−3) _____________________________________
d. 10 + (−2) − 7 _____________________________________
e. −5 − 3(2 − 6) _____________________________________
f. (3 − 3)(102 − 11) _____________________________________
Answers
The simplified form of each of the given expressions as required is as follows;
a. -2 + 5 = 3
b. (−4) • (6) = -24
c. 4 − (−3) = 4 + 3 = 7
d. 10 + (−2) − 7 = 10 - 2 - 7 = 1.
e. −5 − 3(2 − 6) = -5 -3(-4) = -5 + 12 = 7.
f. (3 − 3)(102 − 11) = (0) (91) = 0.
What are the simplified forms of the given arithmetic operations?It follows from the task content that the given arithmetic operations are to be simplified.
The PEMDAS guidelines which prescribe the order of mathematical operations is used for the computation.
where;
P = Parentheses
E = Exponents
M = Multiplication
D = Division
A = Addition
S = Subtraction
Read more on PEMDAS guidelines;
https://brainly.com/question/24882677
#SPJ1
Help? Very confused math is not my subject.

Answers
Rewrite the root expressions as fractional exponents:
\(\dfrac{\sqrt[3]{7}}{\sqrt[5]{7}} = \dfrac{7^{1/3}}{7^{1/5}}\)
Recall that \(\frac{a^m}{a^n} = a^{m-n}\), so that
\(\dfrac{7^{1/3}}{7^{1/5}} = 7^{1/3 - 1/5}\)
Simplify the exponent:
\(\dfrac13 - \dfrac15 = \dfrac5{15} - \dfrac3{15} = \dfrac{5-3}{15} = \dfrac2{15}\)
Then you end up with
\(\dfrac{\sqrt[3]{7}}{\sqrt[5]{7}} = 7^{2/15}\)
18x = ~36?
What is the value of x in the equation 4x
O A. - 13 1 /
O B. - 11 /
O c. 2
O D. 24
Which one is it ?

Answers
Answer:
from the picture the answer is D.
Step-by-step explanation:
\(2 \ti4mes \f7rac{?}{?} \)
to find x subtract 18x from 4x which will give you -14x=-36
divide both sides by -14
9/3 = x/7 what is x and where did you find your answer
Answers
Answer:
x = 21
Step-by-step explanation:
9/3 = 3
7 x 3 = 21
Answer:
x = 21
9/3 = 3
(3)(7) = 21
And I got the answer from my brain
Step-by-step explanation:
if 8 is the mean of x+x+3+x-5+2x+3 find the value of x
Answers
The value of x is equal to 6.2.
How to calculate the mean for the set of data?In Mathematics and Geometry, the mean for this set of data can be calculated by using the following formula:
Mean = [F(x)]/n
For the total number of data based on the information provided above, we have the following;
Total data, F(x) = x + x + 3 + x - 5 + 2x + 3
Total data, F(x) = 5x + 1
Now, we can calculate the value of x as follows;
8 = (5x + 1)/4
32 = 5x + 1
5x = 32 - 1
x = 31/5
x = 6.2
Read more on mean here: brainly.com/question/9550536
#SPJ1
For each of the following transfer functions, write the corresponding differential equation. (a) X(s)/F(s) = 7 / (s^2 + 5s + 10) (b) X(s)/F(s) = 15 / (s + 10)(s + 11)
Answers
X(s)/F(s) = 7 / (s^2 + 5s + 10) => x''(t) + 5x'(t) + 10x(t) = 7f''(t) + 35f'(t) + 70f(t) (3)X(s)/F(s) = 15 / (s + 10)(s + 11) => x''(t) + 21x'(t) + 110x(t) = 15f(t) - 150f'(t) + 1650f''(t) (4)
The given transfer functions are as follows:X(s)/F(s) = 7 / (s^2 + 5s + 10) (1)X(s)/F(s) = 15 / (s + 10)(s + 11) (2)Differential equation is required for each transfer function. The given functions represent the response of the system X(s) to the input F(s).The corresponding differential equation for the first transfer function is given by the inverse Laplace transform of (1),X(s)/F(s) = 7 / (s^2 + 5s + 10) => L^-1 [X(s)/F(s)] = L^-1 [7 / (s^2 + 5s + 10)]=> x''(t) + 5x'(t) + 10x(t) = 7f''(t) + 35f'(t) + 70f(t)The differential equation of the second transfer function is given by the inverse Laplace transform of (2),X(s)/F(s) = 15 / (s + 10)(s + 11) => L^-1 [X(s)/F(s)] = L^-1 [15 / (s + 10)(s + 11)]=> x''(t) + 21x'(t) + 110x(t) = 15f(t) - 150f'(t) + 1650f''(t)The final answers are:X(s)/F(s) = 7 / (s^2 + 5s + 10) => x''(t) + 5x'(t) + 10x(t) = 7f''(t) + 35f'(t) + 70f(t) (3)X(s)/F(s) = 15 / (s + 10)(s + 11) => x''(t) + 21x'(t) + 110x(t) = 15f(t) - 150f'(t) + 1650f''(t) (4)
Learn more about Differential equation
brainly.com/question/14620493
#SPJ11
What is formula in cell B3?
a)=0.2*EXP(C3) b)=C3-0.2*EXP(C3) c) -0.2 C3 d) =abs(C3-B3)
Answers
The formula in cell B3 is "=C3-0.2*EXP(C3)". It calculates the value by subtracting 0.2 times the exponential value of C3 from C3.
The formula "=C3-0.2*EXP(C3)" is present in cell B3. This formula calculates a value based on the value in cell C3. It starts by computing the exponential value of C3 using the "EXP" function, which raises the mathematical constant "e" to the power of C3. Then, it multiplies the exponential value by 0.2. This product is subtracted from the value in cell C3. Therefore, the resulting value in cell B3 is obtained by subtracting 0.2 times the exponential value of C3 from C3 itself.
The purpose of this formula could vary depending on the context of the spreadsheet. However, based on the given information, it seems to be calculating the difference between the value in cell C3 and a modified version of C3. The modification involves subtracting a fraction of the exponential value of C3 from C3. This could be used, for example, to analyze the impact of an exponential decay factor (0.2 in this case) on the original value in C3. The resulting value in cell B3 represents the difference between the original value and the modified value.
Learn more about exponential here:
https://brainly.com/question/29160814
#SPJ11
determine the domain and range for this relationship. Drag the tiles to the correct locations. Not all tiles will be used.
domain:
range:
{4,4.5,5,5.5,6,6.5,7}
(i cant do the less than or equal to sign but its supposed to have the line under it.)
4
0
4
{4,5,6,7}
0
0
{0,1,2,3,4,5,6}
pleaseee help me thanks
Answers
Answer in the picture below!

What adds to be the bottom numbers but also multiplies to be the top?
39 at the bottom and 25 on the right above 39
Answers
Answer:
well i cant answr it if i oont have more inforomartion
Step-by-step explanation:
Answer:
Step-by-step explanation:
if we add 11,36,61,... to the bottom we get bottom as a multiple of numerator.
Can someone pleaseeee help and if you’re correct i’ll give brainliest

Answers
Answer:i think is the fourth one or the fifth one.
Step-by-step explanation:
Answer:
california
Step-by-step explanation:
how many of these figures have atleast one vertex
Answers
The graph shows the responses of 80 students who were asked whether they spend too much or too little time watching television. How many thought they watched too little television?

Answers
Step-by-step explanation:
\(80 \div 100 \times 30 = \\ = 0.8 \times 30 = \\ = 24\)
F(-1)=-6 f(4)=-6
write a linear function f with the given values
(The top one please)
Answers
Answer:
y=-6.
Step-by-step explanation:
1) the condition f(-1)=-6 and f(4)=-6 mean points A and B have coordinates (-1;-6) and (4;-6);
2) the points A and B has the same y-coordinate, it means, the required line is parallel to the x-axis and the required function is: y=-6.
P.S. the suggested way is not the only one.
The linear function f is f(x) = -6.
What is a function?A function is a relationship between inputs where each input is related to exactly one output.
Example:
f(x) = 2x + 1
f(1) = 2 + 1 = 3
f(2) = 2 x 2 + 1 = 4 + 1 = 5
The outputs of the functions are 3 and 5
The inputs of the function are 1 and 2.
We have,
f(-1) = -6
f(4) = -6
The linear function f can be in the form of f(x) = mx + c.
Now,
m = (-6 + 6) / (4 - 1)
m = 0/3
m = 0
Now,
f(-1) = -6
x = -1
f(x) = mx + c
f(-1) = 0 x -1 + c
-6 = 0 + c
c = -6
Now,
f(x) = -6
Thus,
The function f is a constant function.
f(x) = -6
Learn more about functions here:
https://brainly.com/question/28533782
#SPJ5
if the curve yf(x) on the interval [a,b] is revolved about the y-axis, the area of the surface generated is .
Answers
The surface area generated by revolving the curve y=f(x) on the interval [a,b] about the y-axis is given by the formula:
2π∫[a,b] f(x)√(1+(f'(x))^2) dx
To find the surface area generated by revolving the curve y=f(x) about the y-axis on the interval [a,b], we need to use the formula for the surface area of a surface of revolution.
This formula is derived by dividing the curve into small segments of length dx and approximating the surface area of each segment as a frustum of a cone. The formula for the surface area of a frustum of a cone is:
dA = 2πr √(dr^2 + dz^2)
where r is the radius of the circular cross-section of the frustum, and dz is the height of the frustum.
Using calculus, we can express r and dz in terms of x and dx. The radius of the circular cross-section of the frustum is equal to f(x), and the height of the frustum is equal to dx. Therefore, we have:
r = f(x)
dz = dx
Substituting these values into the formula for the surface area of a frustum of a cone, we get:
dA = 2πf(x) √(1 + (f'(x))^2) dx
To find the total surface area generated by revolving the curve y=f(x) on the interval [a,b] about the y-axis, we need to integrate dA from a to b:
A = ∫[a,b] dA
= ∫[a,b] 2πf(x) √(1 + (f'(x))^2) dx
This is the formula for the surface area generated by revolving the curve y=f(x) on the interval [a,b] about the y-axis.
For more questions like Area click the link below:
https://brainly.com/question/27683633
#SPJ11
The local theater sells tickets to its plays at a rate of $38 each. Patrons who attend multiple shows are encouraged to buy an annual membership for $80. Members are able to buy tickets at a discounted rate of $20 each. Roger expects to attend 6 shows this year. Grace expects to attend 4 shows. Who would benefit from buying a membership this year?
neither Grace nor Roger
only Grace
only Roger
both Grace and Roger
Worth 40 points plz help fast!
Answers
Answer:
only Rodger
Step-by-step explanation:
Roger:
38*6=228
80+(20*6)=200
He will save 28 dollars with the membership
Grace:
38*4=152
80+(20*4)=160
Grace will pay 8 dollars more with the membership
What are the zeros of the quadratic function?
y = x^2 - 2x - 15
Answers
Answer:
\(x=\frac{1+\sqrt{31}}{2},\:x=\frac{1-\sqrt{31}}{2}\)
Step-by-step explanation:
\(-> 0=2x^2-2x-15\)
-zeros means the solution aka x intercept, meaning y=0
\(x_{1,\:2}=\frac{-\left(-2\right)\pm \sqrt{\left(-2\right)^2-4\cdot \:2\left(-15\right)}}{2\cdot \:2}\)
=> according to quadratic formula
\(x_{1,\:2}=\frac{-b\pm \sqrt{b^2-4ac}}{2a}\)
where \(ax^2+bx+c=0\)
=> \(x_{1,\:2}=\frac{-\left(-2\right)\pm \:2\sqrt{31}}{2\cdot \:2}\)
simplify
\(x_1=\frac{-\left(-2\right)+2\sqrt{31}}{2\cdot \:2},\:x_2=\frac{-\left(-2\right)-2\sqrt{31}}{2\cdot \:2}\)
\(x=\frac{1+\sqrt{31}}{2},\:x=\frac{1-\sqrt{31}}{2}\)
Cannot simplify farther
Evaluate L −1
{ s 2
+2s+10
3s+2
} by the First Translation Theorem. L{e at
f(t)}=F(s−a)=L{f(t)} s→s−a
for any a. (First Translation Theorem) L{sinkt}= s 2
+k 2
k
,L{coskt}= s 2
+k 2
s
Answers
The inverse Laplace transform of s² + 2s + 10 / (3s + 2) using the First Translation Theorem is (1/3) * \(e^{-2/3t\) * δ(t) + (2/3) * \(e^{-2/3t}\) + (10/3) * \(e^{-2/3t}\).
To evaluate L⁻¹{s² + 2s + 10 / (3s + 2)}, we can use the First Translation Theorem along with the known Laplace transforms for certain functions.
First, let's rewrite the expression in terms of a shifted variable:
L⁻¹{s² + 2s + 10 / (3s + 2)} = L⁻¹{(s² + 2s + 10) / (3(s + 2/3))}
According to the First Translation Theorem, for a function f(t) with Laplace transform F(s), we have:
L⁻¹{F(s - a)} = e^(at) * L⁻¹{F(s)}.
Now, let's apply the First Translation Theorem to the terms in the expression:
L⁻¹{s² / (3(s + 2/3))} = \(e^{-2/3t}\) * L⁻¹{(s²) / (3s)} = \(e^{-2/3t}\) * (1/3) * L⁻¹{s} = \(e^{-2/3t}\) * (1/3) * δ(t).
Here, δ(t) represents the Dirac delta function.
L⁻¹{2s / (3(s + 2/3))} = \(e^{-2/3t}\) * L⁻¹{(2s) / (3s)} = \(e^{-2/3t}\) * (2/3) * L⁻¹{1} = \(e^{-2/3t}\) * (2/3) * 1 = (2/3) * \(e^{-2/3t}\).
L⁻¹{10 / (3(s + 2/3))} = \(e^{-2/3t}\) * L⁻¹{10 / (3s)} = \(e^{-2/3t}\) * (10/3) * L⁻¹{1} = \(e^{-2/3t}\) * (10/3) * 1 = (10/3) * \(e^{-2/3t}\).
Finally, combining the results:
L⁻¹{s² + 2s + 10 / (3s + 2)} = (1/3) * \(e^{-2/3t}\) * δ(t) + (2/3) * \(e^{-2/3t}\) + (10/3) * \(e^{-2/3t}\).
Therefore, using the First Translation Theorem, the inverse Laplace transform of s² + 2s + 10 / (3s + 2) is (1/3) * \(e^{-2/3t}\) * δ(t) + (2/3) * \(e^{-2/3t}\) + (10/3) * \(e^{-2/3t}\).
To know more about inverse Laplace transform, refer to the link below:
https://brainly.com/question/31322563#
#SPJ11
a new surgical procedure has promising results to alleviate hip pain, but a portion of patients who receive the procedure report an audible squeaking noise coming from the hip within six months. data from a follow-up study of 143 patients revealed that 10 of them could hear squeaking. if you were to construct a 99% confidence interval around the follow-up study point estimate, what would be e, the margin of error, or half-width of the confidence interval? since the sample size is so large, you can reference the z distribution to determine a critical
Answers
The margin of error of the 99% confidence interval is given as follows:
0.0549 = 5.49%.
What is a confidence interval of proportions?A confidence interval of proportions has the bounds given by the rule presented as follows:
\(\pi \pm z\sqrt{\frac{\pi(1-\pi)}{n}}\)
In which the variables used to calculated these bounds are listed as follows:
\(\pi\) is the sample proportion, which is also the estimate of the parameter.z is the critical value.n is the sample size.The confidence level is of 99%, hence the critical value z is the value of Z that has a p-value of \(\frac{1+0.99}{2} = 0.995\), so the critical value is z = 2.575.
The margin of error is given as follows:
\(M = z\sqrt{\frac{\pi(1-\pi)}{n}}\)
The parameters for this problem are given as follows:
\(n = 143, \pi = \frac{10}{143} = 0.0699\)
Hence the margin of error is of:
M = 2.575 x sqrt(0.0699 x 0.9301/143)
M = 0.0549 = 5.49%.
More can be learned about the z-distribution at https://brainly.com/question/25890103
#SPJ1
The budget for the valentine's day dance is $500. linghts and decorations cost $125. The Dj charges $100 per hour plus a one time business fee of $75. What is the maximum number of hours the school can have the DJ play at the dance?
Answers
ok
Budget =< lights + DJ + business fee
$500 =< $125 + $100x + $75
solve for x
500 =< 200 + 100x
500 - 200 =< 100x
300 =< 100x
x =< 300/100
x =< 3
The maximum number of hours is 3