Multiply, if possible. Then simplify.
√8 . √32
Answers
The simplified result of √8 * √32 is 16.
To multiply and simplify the expression √8 * √32, we can simplify the square roots individually and then multiply them together.
First, we simplify the square roots:
√8 can be simplified as √(4 * 2). Since the square root of 4 is 2, we can write it as 2√2.
Similarly, √32 can be simplified as √(16 * 2), which is 4√2.
Now, we can multiply the simplified square roots:
(2√2) * (4√2) = 2 * 4 * √2 * √2 = 8 * 2 = 16.
Therefore, the simplified result of √8 * √32 is 16.
Learn more about expression here:
https://brainly.com/question/28170201
#SPJ11
Related Questions
Question 2 (1 point)
According to Hooke's Law, the force needed to stretch a spring varies directly to the amount the spring is stretched 150 pounds of force
stretches a spring five inches, how much will the spring be stretched by a force of 180 pounds?
inches
Blank 1:
Answers
===========================================
Explanation:
x = amount (in inches) the spring is stretched
y = force applied (in pounds)
y = kx for some constant k, since x and y vary directly
---------------
Plug in the given info that x = 5 and y = 150 pair up together
Solve for k
150 = k*5
150/5 = k
k = 30
The equation goes from y = kx to y = 30x. Whatever the stretching distance (x) is, you multiply by 30 to get the force needed (y) to pull the spring.
---------------
Plug in y = 180 to solve for x
y = 30x
180 = 30x
30x = 180
x = 180/30
x = 6
The spring will be stretched 6 inches if you apply a force of 180 pounds.
PLEASE HELP ME WITH THIS TEST the LAST QATION AND I STUCK ON IT

Answers
Answer:
x = 36
∠M = 36
∠P = 71
∠N = 73
Step-by-step explanation:
A triangular prism has a height of 9 m. The area of a triangular base measures 16 m². What is the volume of a triangular prism?
Possible answers are 15,60,72,144 cubic meters. What one is correct?
Answers
Answer:
144m^3
Step-by-step explanation:
A line with a slope of -3 passes through the points (9, r) and (10, 3). What is the value of r? r
Answers
Answer: The value of R is 6.
Explanation: I recommend you go to these two links for an easier understanding:
https://www.wikihow.com/Find-the-Y-Intercept
https://www.wikihow.com/Find-the-X-Intercept
-13x(-13) plz help and i put the brainliest
Answers
Answer:
=169x
Step-by-step explanation:
I hope this helps you
The Augello family is driving from Columbus to St. Louis at a constant rate of 65 miles per hour. The distance between the two cities is 420 miles. Write an equation in slope-intercept form to represent the distance y in miles remaining after driving x hours.
Answers
Answer:
\( y = -65x + 420 \)
Step-by-step explanation:
Slope-intercept form equation is given as \( y = mx + b \)
Where,
y = distance remaining
x = hours driven
m = slope/constant rate. In this case, the value of m would be -65. This means the distance will reduce at a constant rate of 65 miles per hour.
b = y-intercept, which is the initial value or the distance between the cities = 420
Plug in the values into the slope-intercept equation, to represent the distance y in miles remaining after driving x hours. You would have:
\( y = -65x + 420 \)
The equation in slope intercept form is \(y =-65x + 420\)
Linear functionA linear function is a function whose value changes at a constant rate.
The given parameters are:
Distance = 420 milesRate = -65 miles per hourA linear function is represented as:
\(y = mx + c\)
Where
m represents the ratec represents the initial value i.e. the distanceSo, the function becomes
\(y =-65x + 420\)
Hence, the equation in slope intercept form is \(y =-65x + 420\)
Read more about linear functions at:
https://brainly.com/question/14323743
pls help its the last question

Answers
Answer:
Step-by-step explanation:
9.2 to 9.3 the top right one
Will make brain list

Answers
For h(x), to find out the maximum, you would need to use this formula: \(c-(\frac{b^{2} }{4a} )\)
We are given the equation: \(h(x)=-x^{2}+4x-2\).
So, a = -1, b = 4, and c = -2. Then, substitute the numbers into the formula:
h(x)'s maximum = \(c-(\frac{b^{2} }{4a} )\)
= \((-2)-(\frac{(4)^{2} }{4(-1)} )\)
= \((-2)-(\frac{16 }{-4} )\)
= \((-2)-(-4)\)
= \(2\)
For g(x), to find out the maximum, just look at the highest point of y on the graph of the function, which is 2.
Thus, the answer you have chosen is the correct answer, which is choice 3: functions g and h have the same maximum of 2. Hope this helps :)
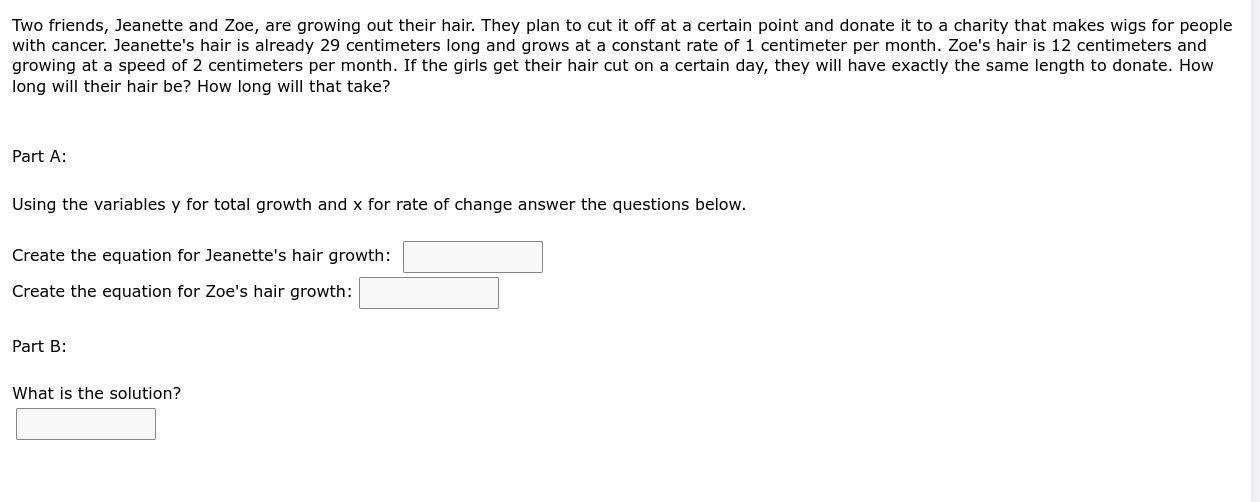
Two friends, Jeanette and Zoe, are growing out their hair. They plan to cut it off at a certain point and donate it to a charity that makes wigs for people with cancer. Jeanette's hair is already 29 centimeters long and grows at a constant rate of 1 centimeter per month. Zoe's hair is 12 centimeters and growing at a speed of 2 centimeters per month. If the girls get their hair cut on a certain day, they will have exactly the same length to donate. How long will their hair be? How long will that take?
Answer the blanks in the picture provided.
Answers
The if they will have exactly the same length to donate. The long will their hair be is 8.5
What is linear equation ?
Linear equation can be defined as the equation in which highest degree is one.
Given ,
Two friends, Jeanette and Zoe, are growing out their hair. They plan to cut it off at a certain point and donate it to a charity that makes wigs for people with cancer.
Jeanette's hair is already 29 centimeters long and grows at a constant rate of 1 centimeter per month.
29+n = 12+2n
2n-n = 29-12
n = 17/2
n = 8.5
Hence, The if they will have exactly the same length to donate. The long will their hair be is 8.5
To learn more about Linear equation from the given link.
https://brainly.com/question/29739212
#SPJ1
Joe uses online banking. He pays the basic charge and pays 7 bills and has an international wire transfer. He also has ATM transactions that include 4 regional network and 4 National network transactions. He also receives a cash advance of $450. What are the total fees for the month?
Answers
The total fees for the month are $71.50.
To calculate the total fees for the month, we need to determine the charges for each transaction and then add them up.
Let's start by breaking down the fees for each transaction:
- Basic charge: Let's assume the basic charge is $10.
- Bill payments: Let's assume each bill payment incurs a $1 fee, so 7 bill payments would be $7.
- International wire transfer: Let's assume the fee for an international wire transfer is $25.
- ATM transactions: Let's assume the fee for a regional network ATM transaction is $1.50 and the fee for a national network ATM transaction is $2.50, so 4 regional network transactions would be $6 and 4 national network transactions would be $10.
- Cash advance: Let's assume the fee for a cash advance is 3% of the amount, so $450 cash advance would be $13.50.
Now we can add up all the fees:
$10 (basic charge) + $7 (bill payments) + $25 (wire transfer) + $6 (regional ATM transactions) + $10 (national ATM transactions) + $13.50 (cash advance fee) = $71.50
Therefore, the total fees for the month are $71.50.
Learn more about :
ATM transactions : brainly.com/question/30487396
#SPJ11
If I get a haircut every two weeks for a year and my haircuts is 40 dollars how much money did I spend in all together?
Answers
Answer: $1040
Step-by-step explanation:
There are 52 weeks in a year so they will get 26 haircuts in the year at every 2 weeks.
26x$40 =$1040
A simple random sample of size 22 is drawn from a population that has a normal distribution. The sample has a mean of 129 and a standard deviation of 3. Compute the standard error of the mean.
Answers
For a simple random sample with size 22 and mean of 129, the computed value the standard error of the mean is equals to the 0.6396.
The standard error is a statistical term that measures the accuracy for which a sample distribution represents a population by using standard deviation. The deviation of sample mean from population mean is called the standard error of mean. SEM is calculated by the standard deviation divided by the square root of the sample size, \(SEM = \frac{ \sigma}{\sqrt{n}}\)
We have a simple random sample from a population that has a normal distribution. Also, Mean of sample = 129
Sample size, n = 22
Standard deviations of sample, σ = 3
Using the formula of standard error of mean is, SEM \(= \frac{ 3}{\sqrt{22}}\)
= 0.63960
Hence, required value is 0.6396.
For more information about standard error of mean, visit :
https://brainly.com/question/29037921
#SPJ4
(2.1 x 104) + (3.5 x 106)
Answers
Answer:
the answer is 589.4
Step-by-step explanation:
In doing this equation you do the order of operations:
(2.1 x 104)= 218.4
(3.5 x 106)= 371
you then add the answers together to get:
218.4 + 371 = 589.4
son las partes de las que se compone el reporte de la encuesta:
A)lnicio, desarrollo y fin.
B) lntroduccion, desarrollo y conclusiones.
C ) Entrada,cuerpo y cierre
D )Ninguno de los anteriores
Answers
Answer:
Na dimio reso
Ta pidi yo
Era olvida yo contigo
Step-by-step explanation:
there's no explanation
if z is inversely proportional to x and z = 5 when x = 7, find the value of x when z = 70
Answers
Answer:
x = \(\frac{1}{2}\)
Step-by-step explanation:
Given that z is inversely proportional to x then the equation relating them is
z = \(\frac{k}{x}\) ← k is the constant of proportion
To find k use the condition z = 5 when x = 7 , then
5 = \(\frac{k}{7}\) ( multiply both sides by 7 )
35 = k
z = \(\frac{35}{x}\) ← equation of proportion
When z = 70 , then
70 = \(\frac{35}{x}\) ( multiply both sides by x )
70x = 35 ( divide both sides by 70 )
x = \(\frac{35}{70}\) = \(\frac{1}{2}\)
Megan wants to find the minimum value of the function y = x^2 - 10x + 3. She begins to convert the equation into vertex form by completing the square. Megan stops at the step below.
y = (x + a)^2 + 3 + b
what number should Megan use to replace the a in this equation?
A. 25
B. -10
C. 100
D. -5

Answers
The equation y = x^2 - 10x + 3 is in standard form
Megan should replace the a in y = (x + a)^2 + 3 + b with -5
How to write the function in vertex form?The function is given as:
y = x^2 - 10x + 3
Rewrite as:
y = (x^2 - 10x) + 3
Take the coefficient (k) of x
k = -10
Divide by 2
k/2 = -5
Square both sides
(k/2)^2 = 25
So, we have:
y = (x^2 - 10x + 25 - 25) + 3
Rewrite as:
y = (x^2 - 10x + 25) - 25 + 3
Express as a perfect square
y = (x - 5)^2 - 25 + 3
From the question, we have:
y = (x + a)^2 + 3 + b
By comparing both equations, we have:
a = -5
Hence, Megan should replace the a in y = (x + a)^2 + 3 + b with -5
Read more about vertex forms at:
https://brainly.com/question/15914313
which expression is equivalent to (3x+4)(7x-2)
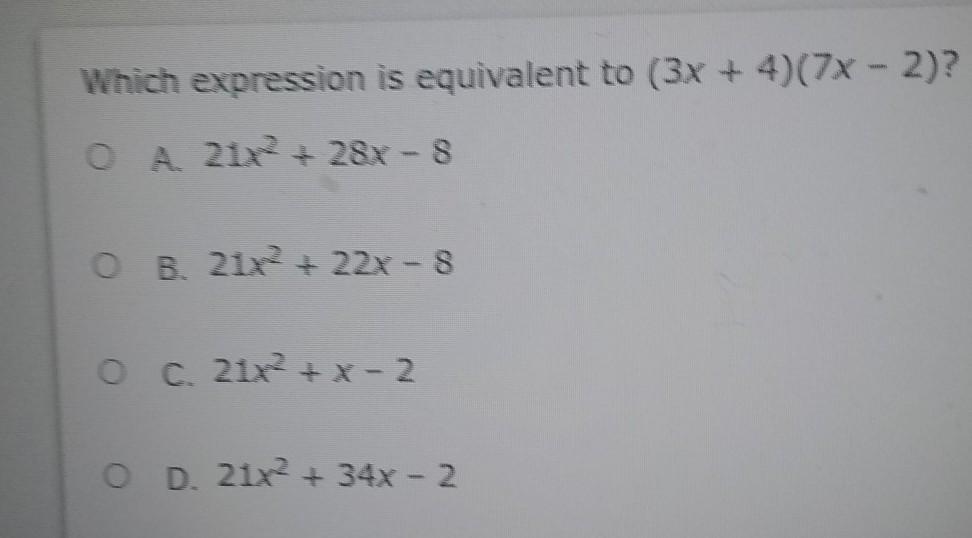
Answers
Answer:
21x² + 22x - 8
Step-by-step explanation:
(3x+4)(7x-2) = 21x² - 6x + 28x - 8 = 21x² + 22x - 8
Answer:
B.
Step-by-step explanation:
Let's use the FOIL (First, Outside, Inner, Last) method.
We need to multiply the first terms in each pair of parentheses, which are
3x and 7x.
Multiplying, we get \(21x^2\).
We multiply the outside terms in each pair, which are 3x and -2.
Multiplying, we get \(-6x\). Remember that since -2 is negative, then the whole product is negative.
We need to multiply the inner terms now. They are 4 and 7x.
Multiplying, we get \(28x\).
Finally, we multiply the last terms in each pair of parentheses. they are 4 and -2.
Multiplying, we get \(-8\).
Now, we put the terms together.
\(21x^2-6x+28x-8\)
We can combine -6x, and 28x. Doing this, we get
\(21x^2+22x-8\). Which gives us B.
I hope this helped.
How would you interpret the statement that b (14)=170
Answers
Answer:
B= 12.14
14 multiplied by B= 170
170/14 = 12.14
Step-by-step explanation:
What is 6% equivalent to

Answers
Answer: 0.06, 6/100, 3/50
Step-by-step explanation:
consider f(x), g(x) and a(x), for x where h(x) = (fo g)(x).given that 2(3)=7, g'(3)=4 and f'(7) =—5, find the gradient of the normal to the curve of h at x =3.
Answers
The gradient of the normal to the curve of h at x =3 is 1/20.
The provided is that h(x) = f(g(x))
Also provided,
g(3) = 7
g'(3) = 4
And f'(7) = -5
Taking,
h(x) = f(g(x))
Differentiating with respect to x,
h'(x) = f('g(x)).g'(x)
h'(x) is the gradient of the curve at x,
Now, putting the value of x = 3,
h'(x) = f('g(3)).g'(3)
We know, g(3) = 7 and g'(x) = 4,
h'(x) = f'(7).4
We know, f'(x) = -5
h'(x) = -5(4)
h'(x) = -20
The slope to the curve at x = 3 is -20.
We know the slope of the normal M1 and the slope at the curve M2 at the same point has their product value as -1,
So,
M1.M2 = -1
M1(-20) = -1
M1 = 1/20
So, the slope of the normal at the curve at x = 3 is 1/20.
To know more about slope, visit,
https://brainly.com/question/16949303
#SPJ4
0.60792 liters x ________ = 607.92 mL
Answers
0.60792 liters x 1000 = 607.92 mL
Multiplying the volume in liters by 1000 converts it to milliliters.
3x^2+bx+24=0 if one of its root is 3
Answers
Answer:
Other root of equation, \(\frac{8}{3}\)
Value of (\(b\)), \(-17\)
Step-by-step explanation:
The given quadratic equation is in standard form. Standard form is the basic format of representing a quadratic equation, a quadratic equation in standard form would use the following format,
\(y=ax^2+bx+c\)
To solve this problem, one could use Vieta's theorems. Vieta's theorems state the following, let (\(x_1\)) and (\(x_2\)) represent the roots of the equation,
\((x_1)+(x_2)=-\frac{b}{a}\)
\((x_1)(x_2)=\frac{c}{a}\)
Substitute the given values into the equation,
\(3+(x_2)=-\frac{b}{3}\)
\((3)(x_2)=\frac{24}{3}\)
Simplify,
\((3)+(x_2)=-\frac{b}{3}\)
\((3)(x_2)=8\)
Inverse operations,
\((3)+(x_2)=-\frac{b}{3}\)
\(x_2=\frac{8}{3}\)
Substitute in the value of (\(x_2\)),
\((3)+(\frac{8}{3})=-\frac{b}{3}\)
Simplify, convert to improper fractions and combine like terms,
\(\frac{9}{3}+\frac{8}{3}=-\frac{b}{3}\)
\(\frac{17}{3}=-\frac{b}{3}\)
Multiply both sides of the equation by (\(3\)) to remove the denominator,
\(17=-b\)
Solving equations with like terms -8 -3y + 2y =32
Answers
Answer:
y= -40
Step-by-step explanation:
Factor 16x2 - 48x + 36 completely.
OA) (8x - 12)2
OB6)2
B) (4x - 6)2
C) 2(2x - 3)2
D) 4(2x - 3)2
-
pls help
Answers
We then applied the formula for factoring perfect square trinomials to obtain the fully factored form, which is 4(2x - 3)2.
To factor 16x2 - 48x + 36 completely, we can first factor out the greatest common factor of 4:
16x2 - 48x + 36 = 4(4x2 - 12x + 9)
Now,
we can see that the expression inside the parentheses is a perfect square trinomial, which can be factored as:
4x2 - 12x + 9 = (2x - 3)2
Therefore, putting the pieces together, we have:
16x2 - 48x + 36 = 4(4x2 - 12x + 9) = 4(2x - 3)2
Hence, the fully factored form of the given expression is 4(2x - 3)2, which is option D).
The reason why we can recognize 4x2 - 12x + 9 as a perfect square trinomial is because it has the form a2 - 2ab + b2, where a = 2x and b = 3. This form can always be factored as (a - b)2, which in this case gives (2x - 3)2. The factor of 4 in the final answer comes from the fact that we initially factored out a 4 from the given expression.
Learn more about Trinomial:
brainly.com/question/11379135
#SPJ11
If \(\frac{1}{a}:\frac{1}{b}:\frac{1}{c}=3:4:5\), then \(a:b:c\)
Answers
The calculated solution for the ratio given as a : b : c is 1/3 : 1/4 : 1/5
How to evaluate the ratioFrom the question, we have the following parameters that can be used in our computation:
1/a : 1/b : 1/c = 3 : 4 : 5
Take the inverse of both sides
So, we have
a : b : c = 1/3 : 1/4 : 1/5
The above means that the solution for a : b : c is 1/3 : 1/4 : 1/5
Read more about ratio at
https://brainly.com/question/12024093
#SPJ1
Put the following equation of a line into slope-intercept form, simplifying all
fractions.
4x + 4y = 8
Answers
Answer:
y = -x +2
Step-by-step explanation:
4x + 4y = 8 to its slope-intercept form, y = mx + b:
Subtract 4x from both sides of the equation:
4x + 4y - 4x = 8 - 4x
4y = -4x + 8
Divide both sides of the equation by 4 to isolate y:
\(\frac{4y}{4} = \frac{-4x}{4} + \frac{8}{4}\)
y = -x + 2
Find the volume of the cylinder to the nearest whole number. Use 3.14
for It.
Radius
Seeds 2 in.
Volume of Cylinders
Cylinder
Height
4 in.
DONE
Volume
in.3
SEEDS
V = πr²h
Answers
Answer:
The formula to find the volume of a cylinder is V = πr²h, where V is the volume, r is the radius, and h is the height of the cylinder.
Given that the radius of the cylinder is 2 inches and the height is 4 inches, we can substitute these values into the formula to find the volume:
V = πr²h
V = 3.14 x 2² x 4
V = 3.14 x 4 x 4
V = 50.24
Rounding this value to the nearest whole number, the volume of the cylinder is 50 in³.
Find the surface area of the composite figure. Round your answer to the nearest tenth if necessary.

Answers
If the length,breadth, height of cuboid are 16 m, 5 m,4 m , slant height of pyramid is 10m, height be 6 m then the surface area of composite figure be 444 \(m^{2}\).
Given that the length,breadth,height of cuboid are 16m,5 m, 4m and slant height be 10 m and height be 6 m.
Surface area is basically sum of area of all the sides of a figure.
Surface area of composite figure = area of cuboid less area of common side + area of 2 slant sides of pyramid+area of two triangles.
=2(lb+bh+hl)-(lb)+2(slant height*breadth of cuboid)+2[1/2* length of cuboid* height of triangle)
=2(16*5+5*4+4*16)-16*5+2(10*5)+2[1/2*16*6]
=2(80+20+64)-80+2*50+2(48)
=2(164)-80+100+96
=328-80+196
=248+196
=444\(m^{2}\)
Hence the surface area of composite figure having a cuboid and pyramid on top is 444\(m^{2}\).
Learn more about surface area at https://brainly.com/question/1297098
#SPJ1
Evaluate the expression when x = 6 and y = 2:

Answers
Answer:
\( \frac{3x + 4}{y} = \frac{3(6) + 4}{(2)} \\ = \frac{18 + 4}{2} = \frac{22}{2} \\ = 11\)
Write the equation of a circle with its center at the origin and passes through the
point (5,0)
AND ALSO!!
Write the equation of a circle of with the center (2,3) and containing the point (3,2)
please and thank you ! :)
Answers
The equation of a circle with its center at the origin and passes through the point (5,0): x2+y2=25
the equation of a circle of with the center (2,3) and containing the point (3,2): x2+y2=2
What is Origin ?Origin is referred to as the first point or the starting point from which we begin our calculations or measurements in mathematics, particularly in the subject of coordinate geometry. The 0 on a ruler is the point at which we begin taking measurements; as a result, it is referred regarded as the scale's origin.
(x-h)^2+(y-k)^2=r2
is the equation for a circle with center (h,k) and radius (r).
This yields the more well-known equation
x2+y2=r2
for a circle centered at the origin.
Since we are aware that the circle's center is at its origin, i.e.
This fact, together with the circle's intersection point (5,0), can be used to determine the radius. The distance formula is used for this.
r=√(x2−x1)^2+(y2−y1)^2
In light of (0,0) and (5,0):
r=√(5−0)^2+(0−0)^2
=√25+0
√25=5
⇒ r=5
Therefore, x^2+y^2=(5)^2
x2+y2=25 is the equation for the circle.
(x-h)^2+(y-k)^2=r2 is the equation for a circle with center (h,k) and radius (r).
This yields the more well-known equation x2+y2=r2 for a circle centered at the (2,3).
Since we are aware that the circle's center is at (2,3), i.e.
This fact, together with the circle's intersection point (3,2), can be used to determine the radius. The distance formula is used for this.
r=√(x^2−x1)^2+(y2−y1)^2
In light of (2,3) and (3,2):
r=√(3−2)^2+(2−3)^2
r = √(1)^2+(-1)^2
= √1+1
=√2
⇒ r=√2
Therefore, x2+y2=(√2)^2
x2+y2=2 is the equation for the circle.
To learn more about Origin click on the link
https://brainly.com/question/396450
#SPJ13