Find the equation of the line passing through the points (2, -1) and (-6, -5)

y = 2x - 5

y = -1/2 x

y = 1/2 x - 2

y = 1/2 x

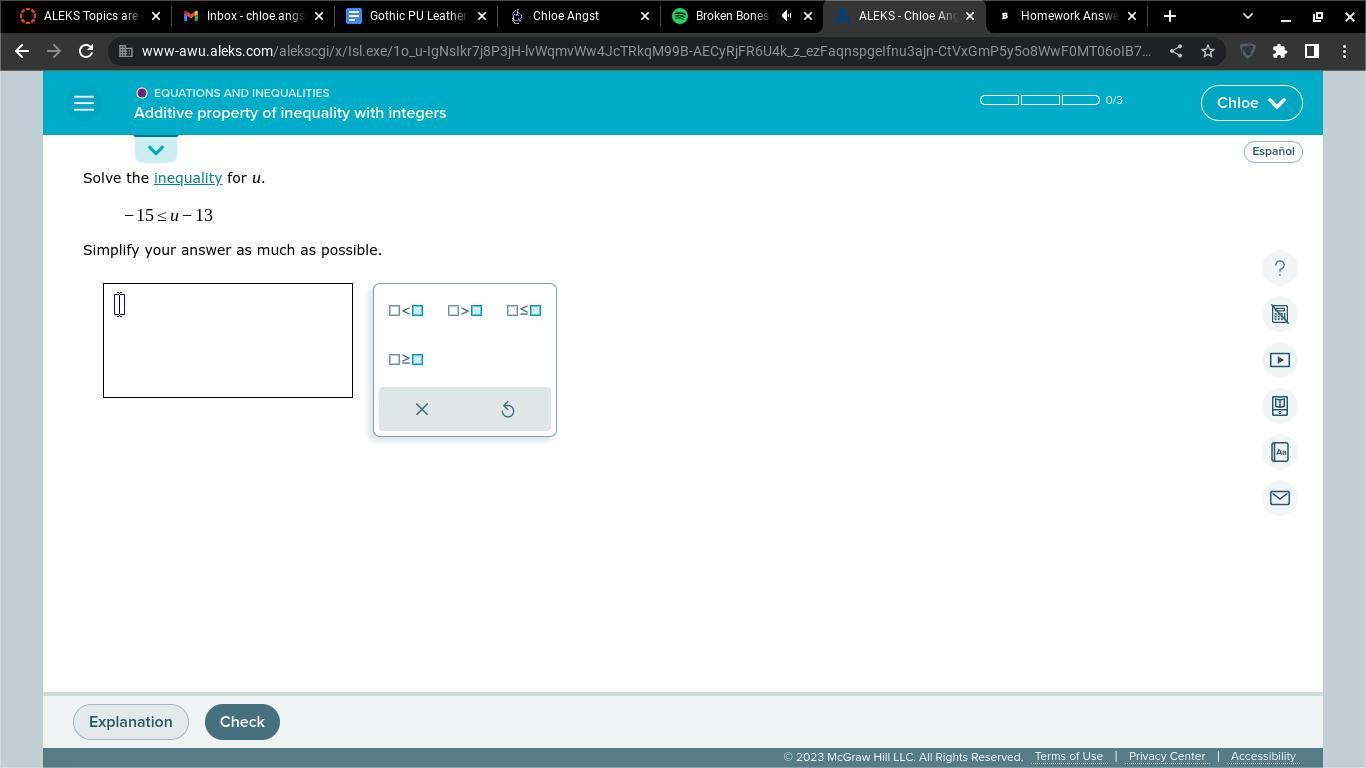
By responding to the query, we can therefore deduce that the answer to u is: \(u\geq -2\)

In mathematics, an equation is a claim that two expressions are equivalent. Two parts that are separated by the algebraic symbol (=) make up an equation. As an illustration, the claim "\(2x+3 = 9\)" makes the statement

Finding the value or values of the variable(s) necessary for the equation to be correct is the goal of equation solving. Equations can have one or more components and be straightforward or complex, regular or nonlinear.

The formula "\(x^{2} +2x-3=0\)" raises the variable x to the second degree. In many various branches of mathematics, including algebra, calculus, and geometry, lines are used.

\(-15\leq u-13\\-15+13\leq 13+13\\-2\leq u\)

Therefore the solution of \(u\geq -2\)

Learn more about equation

https://brainly.com/question/649785

#SPJ1

The gallons of gas that Aubrey would need to travel 111.54 miles is 7.8 gallons.

In Mathematics, a direct proportion simply refers to a mathematical equation which is typically used to represent the equality of two (2) ratios.

By applying direct proportion to the given information, we have the following mathematical equation:

15 gallons of gasoline = 214.5 miles

X gallons of gasoline = 111.54 miles

By cross-multiplying, we have the following:

214.5x = 15 × 111.54

x = 1,673.1/214.5

x = 7.8 gallons of gasoline.

Read more on direct proportion here: https://brainly.com/question/29672991

#SPJ1

In an arithmetic series where the first term is 1 and the nth term is 3, the task is to determine the value of n. By using the formula for the nth term of an arithmetic sequence and solving the equation, it is found that n equals 2 in this case.

The value of n in the arithmetic series, we can use the formula for the nth term of an arithmetic sequence, which is given by a_n = a_1 + (n - 1)d, where a_n represents the nth term, a_1 is the first term, n is the number of terms, and d is the common difference.

In this case, a_1 = 1 and a_n = 3. We need to find the value of n.

Using the formula, we can rewrite it as 3 = 1 + (n - 1)d.

Since we know that the common difference is constant in an arithmetic series, we can subtract 1 from both sides to get 2 = (n - 1)d.

Now, we have a_1 = 1 and a_n = 3. By substituting these values into the formula, we can write it as 3 = 1 + (n - 1)(a_n - a_1).

Substituting the known values, we get 3 = 1 + (n - 1)(3 - 1).

Simplifying further, we have 3 = 1 + 2(n - 1).

Expanding the expression, we get 3 = 1 + 2n - 2.

Combining like terms, we have 3 = 2n - 1.

Adding 1 to both sides, we get 4 = 2n.

Dividing both sides by 2, we find n = 2.

Therefore, the value of n in the given arithmetic series is 2.

To know more about arithmetic series refer here:

https://brainly.com/question/16415816#

#SPJ11

a. The probability that a failure is due to an induced substance is 0.013487 or 1.35% approximately.

b. Probability of a failure due to disease or infection= 44%.

a. Determine the probability that a failure is due to an induced substance.

The total percentage of heart failures that are caused by outside factors is 19%, of which 73% are due to induced substances.

Therefore, the probability that a failure is due to an induced substance = 73/100*19/100= 0.013487 or 1.35% approximately.

b. Determine the probability that a failure is due to disease or infection.

The total percentage of heart failures that are caused by natural occurrences is 81%, of which disease accounts for 27% and infection (e.g., staph infection) accounts for 17%.

Therefore, the probability that a failure is due to disease or infection = 27/100 + 17/100= 0.44 or 44% approximately.

Probability of a failure due to induced substance= 1.35% (approx.)Probability of a failure due to disease or infection= 44% (approx.)

To learn about probability here:

https://brainly.com/question/24756209

#SPJ11

After giving Jimmy one-third of the remaining candy pieces and Selena one-fourth of the remaining candy pieces, Bev is now down to having two-thirds as many as three-quarters as many as twenty-four pieces of candy.

Calculating how much candy is still available after each distribution is necessary if we want to establish how many pieces of candy Bev still possesses. At the beginning, Bev has twenty-four individual bits of candy. After giving Jimmy a third of the candy pieces, the number of pieces that are still remaining may be computed as (2/3) times 24, which is equal to two-thirds of the total amount.

The next thing that happens is that Bev gives Selena a quarter of the remaining candy pieces. We need to multiply the total amount that is still available by one quarter since Selena is entitled to a portion of what is left over after Jimmy has received his part. As a result, the remaining candy pieces can be approximated using the formula (3/4 * (2/3) * 24 after Selena has been given her portion.

The solution to the equation is found to be (3/4) * (2/3) * 24, which is 4 * 8, which equals 32. Therefore, after giving Jimmy one third of the remaining candy pieces and Selena one quarter of the remaining candy pieces, Bev still has 32 pieces of candy left.

Learn more about equation here:

https://brainly.com/question/10724260

#SPJ11

Answer:

This is the distance formula

(x₂ - x₁)² + (y₂ - y₁)² = d²

complete the equation to derive the distance.

(2 - -3)² + (-4 - 3)² = d²

5² + (-7)² = d²

25 + 49 = d²

64 = d²

d = 8

\(AC= d = (x_{2} -x_{1}) ^{2} + (y_{2} -y_{1}) ^{2}\)

\(BC = (y_{2} -y_{1} )\)

\(AB = (x_{2} -x_{1} )\)

The distance between two points is called the length of the line segment.

\(\sqrt{(x_{2}-x_{1}) ^{2}+(y_{2} -y_{1} )^{2} } =d\)

Where,

d is the distance between the two points

\((x_{1},x_{2}) and (y_{1},y_{2})\) are the two points

It states that "the sum of the squares on the legs of a right triangle is equal to the square on the hypotenuse (the side opposite the right angle)".

According to the given question

We have a graph of a triangle ABC

Length of AC  is d.

Coordinates of A is \((x_{1},y_{1})\)

Coordinates of B  is \((2, 3)\)

and coordinates of C is \((x_{2}, y_{2} )\)

Now, according to the distance formula, length of AC is given by

\(AC = d =\sqrt{(x_{2}-x_{1} ) ^{2}+(y_{2}-y_{1}) ^{2} }\)

⇒ \(d = (x_{2} -x_{1}) ^{2} + (y_{2} -y_{1}) ^{2}\)

By Pythagoras theorem and distance formula

\(BC = (y_{2} -y_{1} )\)

And, \(AB = (x_{2} -x_{1} )\)

Learn more about the distance formula and Pythagoras theorem here:

https://brainly.com/question/27801945

#SPJ2

p(x < c) is best described as the area under the cumulative distribution function (cdf), f(x), to the left of c.

In probability theory, the cumulative distribution function (cdf) is a function that provides the probability of a random variable taking on a value less than or equal to a given value. In this case, p(x < c) represents the probability that the random variable x is less than the value c. To find this probability, we look at the area under the cdf, f(x), to the left of c.

The cdf, f(x), is defined as the integral of the probability density function (pdf) of the random variable. The pdf gives the relative likelihood of the random variable taking on a specific value. By integrating the pdf from negative infinity to c, we obtain the area under the curve of the pdf up to the value c, which corresponds to the probability p(x < c).

Therefore, p(x < c) can be interpreted as the area under the cumulative distribution function, f(x), to the left of the value c. It represents the probability that the random variable x is less than c.

Learn more about:Cumulative distribution

brainly.com/question/30402457

#SPJ11

JKL with vertices J(1, 3), K(5, 0), and L(7, 4) and its image after the glide reflection with a translation along <0,-3> and areflection in the y-axis is J'(-1, 0), K'(-5, -3), L'(-7, 1).

In the given question we have to graph JKL with vertices J(1, 3), K(5, 0), and L(7, 4) and its image after the glide reflection with a translation along <0,-3> and areflection in the y-axis.

The given vertices are J(1, 3), K(5, 0), and L(7, 4).

Firstly translation along (0,-3).

So translation is (x,y)→(x,y-3)

J'=(1, 3-3)=(1, 0)

K'=(5, 0-3)=(5, -3)

L'=(7, 4-3)=(7, 1)

We have to find the reflection in the y-axis so (x,y)→(-x,y).

Now the points are J'(-1, 0), K'(-5, -3), L'(-7, 1).

The graph of the glide reflection with a translation along <0,-3> and a reflection in the y-axis is given below.

To learn more about reflection with a translation link is here

brainly.com/question/15577335

#SPJ1

Answer:

Its c

Step-by-step explanation:

Answer:

118.7 inches squared.

Step-by-step explanation:

The area is the total space taken up by a flat (2-D) surface or shape. The area is always measured in square units.

Diameter is the length across the entire circle, the line splitting the circle into two identical semicircles.

The expression for solving the area of a circle is A = π × \(r^{2}\).

To solve for the semicircle above, we can divide the diameter into 2 to get the radius.

So, the radius of the upper semicircle is 6 inches.

If the radius of a circle is 6 inches, then you can substitute r for 6 into the formula.

This simplifies to A = 36π. If a semicircle if half the size of a normal circle, then it will be A = 18π, because 36 ÷ 2 = 18.

To solve for the lower semicircle, we can do the same this as we did above.

But wait, we don't know the radius or diameter!

No worries! To solve for the diameter of the circle, we can take the line that is parallel to the semicircle (the one that has a length of 12in) and subtract 6 from it. We subtract 6 from it because the semicircle takes up the remaining length of the line, not including the 6in.

To solve for the lower semicircle, we can divide the diameter by 2 to get the radius.

So, the radius of the circle is 3.

Now we can insert 3 into the expression.

This simplifies to A = 9π. If a semicircle if half the size of a normal circle, then it will be A = 4.5π because like above, 9 ÷ 2 = 4.5.

Adding the two semicircles together:

So, the area of both semicircles is approximately 70.6858 square inches.

To solve for the area of a rectangle we use the expression:

Inserting the dimensions of the rectangle:

So, the area of the rectangle is 48 square inches.

Adding the two areas together:

Therefore, the area of the entire figure, rounded to the nearest tenth is \(118.7\) \(in^{2}\).

Answer:

x = 3

Step-by-step explanation:

\((8x-8)^{3/2}=64\)

Multiply both sides by the exponent 2/3.

\(8x - 8 = 64^{2/3}\)

Solve for the exponent.

\(8x-8=16\)

Add 8 to both sides.

\(8x = 16+8\)

\(8x=24\)

Divide 8 into both sides.

\(x=24/8\)

\(x=3\)

Answer:

the answer is A

Step-by-step explanation:

In the congruent triangles both triangle are same . In this triangle 2.3.3 congruent triangle image given below to understand better.

According to the question, given that

2.3.3 congruent triangle under congruence triangle are explain:

Both triangles are said to be congruent if the three angles and three sides of one triangle match the corresponding angles and sides of the other triangle. We can see from the examples PQR and XYZ that PQ = XY, PR = XZ, and QR = YZ, and thus P = X, Q = Y, and R = Z. Then, we can state that XYZ and PQR.

To be congruent, the two triangles must be the same size and shape. It is necessary for both triangles to be superimposed on one another. A triangle appears to be in a different place or have a different look as we rotate, reflect, and/or translate it.

Two triangles must meet five requirements in order to be congruent. The congruence properties are SSS, SAS, ASA, AAS, and RHS.

SSS Congruence Criteria

Side-Side-Side criterion is referred to as the SSS criterion. According to this standard, two triangles are congruent if their respective triangles' three sides are equal to one another.

The three angles of BAC must be identical to the corresponding angles of XYZ if, according to the SSS condition, BAC XYZ.

SAS Congruence Criteria

Side-Angle-Side criterion is referred to as the SAS criterion. According to this standard, two triangles are said to be congruent if their matching sides and included angles are the same on each side of each other.

The third side (AB) and the other two angles of ABC must be identical to the equivalent side (XY) and the angles of XYZ if ABC XYZ under the SAS condition.

ASA Congruence Criteria

Angle-Side-Angle criterion is also known as ASA criterion. According to the ASA criterion, two triangles are congruent if any two of their included sides and related angles are equivalent in size to those of the other triangle.

AAS Congruence Criteria

Angle-Angle-Side criterion is also known as the AAS criterion. Two triangles are said to be congruent according to the AAS criterion if any two angles and the non-included side of one triangle match the corresponding angles and side of the second triangle.

RHS Congruence Criteria

Right angle-hypotenuse-side congruence is referred to as the RHS criterion. If the hypotenuse and side of one right-angled triangle are equal to the hypotenuse and the corresponding side of another right-angled triangle, then two triangles are said to be congruent according to this criterion.

To learn more about congruent triangle visit here : brainly.com/question/22062407

#SPJ1

The equivalent statement involving an exponent of the given logarithmic statements are :

(a)  a^5 = 4

(b) 2^4 = 16

a.) loga4 = 5
To change this logarithmic statement into an equivalent statement involving an exponent, we use the following format:

base^(exponent) = value.
In this case, the base is "a", the exponent is 5, and the value is 4.

So the equivalent statement can be written as:
a^5 = 4

b.) log216 = 4
Similarly, for this logarithmic statement, the base is 2, the exponent is 4, and the value is 16.

Thus we can use the following format :

base^(exponent) = value.

So the equivalent statement can be written as:
2^4 = 16

To learn more about logarithmic statements visit : https://brainly.com/question/28041634

#SPJ11

when multiplying fractions , multiply across the numerator and denominator

you dont need common denominators

example

\(\frac{1}{2}*\frac{4}{5}=\frac{1*4}{2*5}\)

Hope this helps : -)

- Jeron

Answer:

3/

10

= 0.3

Step-by-step explanation:

Multiple: 2/5

* 3/4

= 2 · 3/5· 4

= 6/20

= 3 · 2/10 ·  2

= 3/10

Multiply both numerators and denominators. Result fraction keep to lowest possible denominator GCD(6, 20) = 2. In the following intermediate step, cancel by a common factor of 2 gives 3/

10

.

In other words - two fifths multiplied by three quarters = three tenths.

The required 2/5 x 3/4 in its most reduced form is 3/10.

In mathematics, reducing an expression to its simplest or most reduced form involves simplifying or canceling common factors or terms. This process is often applied to fractions, radicals, or algebraic expressions.

To multiply fractions, multiply the numerators and multiply the denominators:

(2/5) x (3/4) = (2 x 3) / (5 x 4) = 6/20

To simplify or reduce the fraction, find the greatest common divisor (GCD) of the numerator and denominator, which is 2:

6/20 = (6 ÷ 2) / (20 ÷ 2) = 3/10

Therefore, 2/5 x 3/4 in its most reduced form is 3/10.

Learn more about reduced form here:

https://brainly.com/question/5054385

#SPJ6

Answer:

The other angle is 100

Step-by-step explanation:

A straight line is 180, and if one angle is 80, then 180-80=100, giving you your answer. ^^

Answer:

i think the answer will be 180-80?

the general solution of the given differential equation is: y = C7 + C8/x.

The differential equation 7xy'' + 7y' = 0 can be solved as follows: 7xy'' + 7y' = 0

The given differential equation is a second-order linear differential equation of the form y'' + f(x) y' + g(x) y = 0, where f(x) = 7/x and g(x) = 0.

On dividing both sides by 7x, we get:y''/x + y'/x = 0

Now, substituting p = y'/x, we get:y''/x = dp/dx + p/x

Hence, the given differential equation can be written as:dp/dx + p/x = 0

This is a first-order linear differential equation of the form dy/dx + P(x) y = Q(x), where P(x) = 1/x and Q(x) = 0.

Now, multiplying both sides by the integrating factor I(x) = e^∫P(x)dx = e^∫(1/x)dx = e^lnx = x, we get:x dp/dx + p = 0

Multiplying both sides by dx and integrating, we get:∫x dp/p = -∫dxln |p| = -ln |p| + C1x ln |p| = C2

Taking antilogarithm on both sides, we get:|p| = e^(C2/x)Multiplying both sides by a constant of integration C3, we get:

p = ± C3 e^(C2/x)But p = y'/xHence, y'/x = ± C3 e^(C2/x)

Integrating both sides with respect to x, we get:y = C4 + C5 ∫e^(C2/x) dxwhere C4 and C5 are constants of integration.

Using the substitution t = C2/x, we get:dy/dt = - C5/t²Hence, y = C4 - C5 ∫dt/t²= C4 + C5/t + C6 where C6 is a constant of integration.

Substituting t = C2/x, we get:y = C4 + C5 C2/x + C6x= C7 + C8/x where C7 and C8 are constants of integration.

Hence, the general solution of the given differential equation is: y = C7 + C8/x.

To learn more about Differential equation visit: brainly.com/question/16663279

#SPJ11

Answer:

y = 53

Step-by-step explanation:

To get the answer, first put 19 in place of the x, and then times that iwth two, getting 38, you add that to 15. The answer is 53.

Answer:The Value of the coin after 19 years is $53.

Step-by-step explanation:If x=age, then simply substitute the x in “2x+15” for 19.After you do that you should get y=2(19)+15.Do the math to get y=38+15. Y=53.

a)  The equation log3(x) + 2log3(x) = log3(1) + 2log3(8) needs to be solved for x.b) The equation 50000 = 3600(1 + 0.18 * 12x) needs to be solved for x.

a)To solve the equation log3(x) + 2log3(x) = log3(1) + 2log3(8), we can simplify it using logarithmic properties.

First, let's simplify the right side of the equation. We know that log3(1) is equal to 0, as any number raised to the power of 0 is always 1. Additionally, we can use the property loga(b^c) = cloga(b) to simplify 2log3(8). Since 8 can be expressed as 2^3, we have 2log3(8) = 2log3(2^3) = 2 * 3log3(2) = 6log3(2).

Substituting these values back into the equation, we have log3(x) + 2log3(x) = 0 + 6log3(2). Combining like terms, we get 3log3(x) = 6log3(2).

To solve for x, we can use the property loga(b) = logc(b) / logc(a). Applying this property, we have log3(x) = (6log3(2)) / 3. Simplifying further, we get log3(x) = 2log3(2).

Now, we can use the property loga(b) = c to rewrite the equation as x = 3^2log3(2). Evaluating the logarithm, we have x = 3^2(1) = 3^2 = 9.

Therefore, the solution to the equation log3(x) + 2log3(x) = log3(1) + 2log3(8) is x = 9.

b) To solve the equation 50000 = 3600(1 + 0.18 * 12x), we can follow the steps below.

First, let's simplify the expression inside the parentheses. Multiplying 0.18 by 12 gives us 2.16, so we have 1 + 2.16x.

Next, we can distribute the 3600 to the terms within the parentheses, resulting in 3600 + 3600(2.16x). Our equation becomes 50000 = 3600 + 3600(2.16x).

To isolate the variable x, we need to move the constant term 3600 to the right side of the equation. Subtracting 3600 from both sides, we have 50000 - 3600 = 3600(2.16x).

Simplifying further, we get 46400 = 3600(2.16x).

To solve for x, we divide both sides by 3600(2.16). This gives us 46400 / (3600 * 2.16) = x.

Evaluating the right side of the equation, we have x ≈ 6.29.

Therefore, the solution to the equation 50000 = 3600(1 + 0.18 * 12x) is approximately x = 6.29.

To learn more about equation  Click Here: brainly.com/question/29657983

#SPJ11

Answer:

y =11/3x+7 point intercept form

Step-by-step explanation:

y-7=11/3 *(x + 0) point slope form

Area of the scale drawing is 10 square inches. Area of the actual deck would be 160 square feet.

Area of the actual deck would be 16 times the area of the scale drawing.

Area of a two dimensional shape is the total region which is bounded by the object's shape.

Given scale drawing can be made into two squares.

One with a side length of 1 inch and other with side length of 3 inches.

Area of a square with side length 'a' = a²

Area of the given scale drawing = (1 inch)² + (3 inches)²

                                                     = 1 inch² + 9 inches²

                                                     = 10 inches²

If the scale is 1 inch = 4 feet,

Area of the actual deck = (1 × 4 feet)² + (3 × 4 feet)²

                                       = 4² [(1 feet)² + (3 feet)²]

                                       = 4² (10)

                                       = 160 feet²

Area of the actual deck = 160 feet²

                                        = 16 (Area of the scale drawing)

If the scale is 1 inch = k feet,

Area of the actual deck = k² ( Area of scale drawing)

                                        = 10 k² square feet

Hence the area of the actual deck would be 10k² if the scale is 1 inch = k feet.

Learn more about Area here :

https://brainly.com/question/12916755

#SPJ9

So, Isaac should pour 81 cubic inches of mixture into the pan.

Volume is a physical quantity that refers to the amount of space occupied by an object or a substance. It is typically measured in cubic units, such as cubic meters, cubic centimeters, or cubic feet.

The volume of an object or substance can be determined by measuring its length, width, and height (in the case of a solid object), or by measuring the amount of space it occupies (in the case of a liquid or gas).

In mathematical terms, the volume of an object is given by the formula V = l x w x h, where l is the length, w is the width, and h is the height. For irregularly shaped objects, the volume may be determined by displacement - that is, by measuring the amount of water or other fluid displaced by the object when it is immersed in the fluid.

To find the volume of the mixture that Isaac should pour into the pan, we need to find the volume of the pan itself and subtract the volume of the space that needs to be left empty.

The pan is in the shape of a rectangular prism, so its volume is:

V_pan \(= l *w * h = 9 in. * 9 in. * 2 in. = 162 in^{3}\)

To leave room for the casserole to rise, Isaac should only fill the pan to a height of 1 inch. So, the volume of the space that needs to be left empty is:

V_empty \(= l *w * h = 9 in. * 9 in. * 2 in. = 81 in^{3}\)

Therefore, the volume of the mixture that Isaac should pour into the pan is:

V = V_pan - V_empty\(= 162 in^{3} - 81 in^{3} = 81 in^{3}\)

To know more about the volume, visit:

https://brainly.com/question/13338592

#SPJ1

The constant G is a fundamental constant in physics and plays a crucial role in understanding the gravitational interactions between celestial bodies.\(A.d is N.m^2/kg^2.\)

Conversions:

10 meters to feet: 10 meters * 3.281 feet/meter = 32.81 feet

100 kilograms to pounds: 100 kilograms * 2.2046 pounds/kilogram = 220.46 pounds

50 Kelvin to Rankine: 50 Kelvin * (9/5) Rankine/Kelvin = 90 Rankine

6 micrometers to centimeters: 6 micrometers * 0.0001 centimeters/micrometer = 0.0006 centimeters

The unit of the constant G in the formula F.L.G = \(A.d is N.m^2/kg^2.\)

In the given formula, F represents force in Newtons (N), L represents length in meters (m), G represents the constant with the unit of \(N.m^2/kg^2\), A represents area in square meters (\(m^2\)), and d represents distance in meters (m).

The unit \(N.m^2/kg^2\) represents the gravitational constant in physics, commonly denoted as G. It is used in the calculation of gravitational forces between objects. The unit can be broken down as follows: N represents the unit of force, \(m^2\)represents the unit of area, and\(kg^2\)represents the square of the unit of mass. The constant G is a fundamental constant in physics and plays a crucial role in understanding the gravitational interactions between celestial bodies.

Learn more about kilograms here:

https://brainly.com/question/29761698

#SPJ11

The correct option is C: 2*2/3 yards, 8 feet is expressed as the 2 ²/₃ yards.

Numbers that make up a portion of the whole are referred to as fractions.

Divide the length either by conversion ratio to change a foot measure to a yard measurement.

Given that one yard is equals to three feet, you can use the following straightforward formula to convert:

yards = feet / 3

The length in question is eight feet.

The corresponding yard measurement is so expressed as follows:

yards = 8/3

As a result of converting it to mixed fractions,

2 ²/₃ yards.

Thus, 8 feet is expressed as the 2 ²/₃ yards.

To know more about the mixed fractions, here

https://brainly.com/question/29507294

#SPJ4

The correct question is-

A clothesline rope is 8 feet long. Which of these is another way to express 8 feet? answer choices

A; 2*1/3 yards

B: 2*1/2 yards

C: 2*2/3 yards

D: 2*3/4 yards

Answer:

x = 13

Step-by-step explanation:

NM + ML + LK = x - 6 + 9 + 2x - 19

NK = 23

x - 6 + 9 + 2x - 19 = 23

3x - 16 = 23

3x = 39

x = 13

answer: -13a + 1b

-5a - 8 = -13

2b - b = 1b

Answer:

Combine like terms

−5a -8a+2b-b

13a+2b-b

2

Combine like terms

again

3

Multiply by 1

Solution

-13a+b

Step-by-step explanation:

Answer:

see explanation

Step-by-step explanation:

the inscribed angle ADB is half the arc that subtends it , AB , then

AB = 2 × ∠ ADB = 2 × 46° = 92°

the central angle BOC is equal to the arc that subtends it, BC , so

BC = 22°

Then

ABC = AB + BC = 92° + 22° = 114°

-----------------------------------------------------

BD is a diameter of the circle , so BAD = 180° THEN

AD = 180° - AB = 180° - 92° = 88°

-----------------------------------------------------

the sum of the arcs on a circle = 360° , so

ADC = 360° - ABC = 360° - 114° = 246°