Find the roots of the equation below.
x² - 6x + 12 = 0
O-3± √3
O 3 ± √3
O-3± N3
3 ± N√3
Answers
x = (-b ± √(b² - 4ac)) / 2a
where a, b, and c are the coefficients of the quadratic equation.
In this case, a = 1, b = -6, and c = 12. Substituting these values into the formula, we get:
x = (6 ± √(6² - 4(1)(12))) / 2(1)
x = (6 ± √(36 - 48)) / 2
x = (6 ± √(-12)) / 2
Since the square root of a negative number is not a real number, the roots of the equation are complex numbers. We can simplify the expression for the roots by factoring out -1 from the square root:
x = (6 ± √(4 × -3)) / 2
x = (6 ± 2√(-3)) / 2
x = 3 ± √(-3)
Using the imaginary unit i, which is defined as i² = -1, we can write the roots in the form a + bi:
x = 3 ± √3i
Therefore, the roots of the equation x² - 6x + 12 = 0 are 3 + √3i and 3 - √3i.
The quadratic formula states that for an equation of the form ax² + bx + c = 0, the roots are given by:
x = (-b ± sqrt(b² - 4ac)) / 2a
Comparing the given equation to the standard form ax² + bx + c = 0, we have a = 1, b = -6, and c = 12. Substituting these values into the quadratic formula, we get:
x = (-(-6) ± sqrt((-6)² - 4(1)(12))) / 2(1)
Simplifying:
x = (6 ± sqrt(6² - 48)) / 2
x = (6 ± sqrt(-12)) / 2
The expression sqrt(-12) involves an imaginary number, so the roots of the equation are complex conjugates:
x = 3 + sqrt(3)i and x = 3 - sqrt(3)i
Therefore, the roots of the equation x² - 6x + 12 = 0 are x = 3 + sqrt(3)i and x = 3 - sqrt(3)i.
Related Questions
____ : A straight line extending from the center of a circle or sphere to the circumference or surface
Answers
radius : A straight line extending from the center of a circle or sphere to the circumference or surface
In geometry, circles and spheres are two-dimensional and three-dimensional shapes, respectively, that possess unique characteristics. One important concept associated with circles and spheres is the notion of a line extending from their centers to their circumferences or surfaces. This line has a specific name and serves as a fundamental element in understanding these geometric shapes. In this detailed explanation, we will explore the term used to describe this line and delve into its significance.
The line extending from the center of a circle to its circumference, or from the center of a sphere to its surface, is called a "radius." The term "radius" refers to a straight line segment that connects the center of the circle or sphere to any point on its circumference or surface. It is important to note that the term "radius" is used exclusively when referring to circles and spheres and not to other shapes or objects.
Let's examine the properties and significance of the radius in both circles and spheres:
1. Circle:
In a circle, the radius is a fundamental element that defines the size and shape of the circle. It is the distance between the center of the circle and any point on its circumference. Notably, all radii of a circle are equal in length. In mathematical formulas and calculations involving circles, the radius is often denoted by the letter "r."
The radius plays a crucial role in determining various properties of the circle, including its area, circumference, and relationships with other geometric elements, such as chords and tangents.
2. Sphere:
In a sphere, which is a three-dimensional object analogous to a circle, the radius serves a similar purpose. It extends from the center of the sphere to any point on its surface. Like in a circle, all radii of a sphere are equal in length. In mathematical formulas and calculations involving spheres, the radius is typically denoted by the letter "r."
The radius of a sphere is essential in determining properties such as its volume, surface area, and relationships with other geometric elements like great circles or diameters.
The radius is a foundational concept in the study of circles and spheres. It provides a measure of distance from the center to the outer edge and is used in various mathematical calculations and geometric analyses. Moreover, the radius plays a crucial role in determining the symmetry, proportions, and relationships between different parts of circles and spheres.
It is worth mentioning that the concept of the radius is closely related to other geometric elements in circles and spheres. For instance, diameters are lines that pass through the center of a circle or sphere and have endpoints on the circumference or surface, respectively. The diameter is always twice the length of the radius. Additionally, chords are segments within a circle or sphere that connect any two points on the circumference or surface.
The radius bisects any chord that passes through the center, dividing it into two equal segments.
In conclusion, the line extending from the center of a circle to its circumference or from the center of a sphere to its surface is called a "radius." The radius is a fundamental element that defines the size, shape, and proportions of circles and spheres. It plays a crucial role in calculating various properties, such as area, circumference, volume, and surface area.
Understanding the concept of the radius is essential in geometry and provides insights into the symmetrical and proportional relationships within circles and spheres.
To learn more about Sphere:
https://brainly.com/question/28228180
#SPJ11
Please help me! Thank you if you do!

Answers
Answer:
it is C
Step-by-step explanation:
why because look at the numbers and youll understand
step by step explanation :
PLEASE HELP
The slope of the line that represents the data Nicholas collected is -63, and the y-intercept is 825. Explain what these represent in the context of the situation. Remember that x is the number of days, and y is the number of canned goods. explain the answer
Answers
Answer:
sorry i coldent find the anser
Step-by-step explanation:
ello
+v+
Answer:
The slope indicates that the soup kitchen uses 63 cans per day. The y-intercept shows that there were initially 825 cans at the soup kitchen.
Step-by-step explanation:

The fundamental question addressed by the correlational method is
a. "Does variable A cause variable B?"
b. "How is a control group influenced by the absence of an independent variable?"
c. "What impact does random assignment have on psychological behavior?"
d. "Are two or more variables related in some systematic way?"
Answers
The fundamental question addressed by the correlational method is whether two or more variables are related in some systematic way. So the correct option is D.
Correlational method is a research technique used to explore the relationship between two or more variables. In this method, researchers collect data on the variables of interest and analyze their patterns of association. The fundamental question addressed by the correlational method is whether two or more variables are related in some systematic way. This means that researchers are interested in exploring whether changes in one variable are associated with changes in another variable.
For instance, a researcher may be interested in exploring the relationship between stress and job performance. The researcher may collect data on the levels of stress and job performance in a sample of employees and then use statistical analysis to determine if there is a systematic relationship between the two variables. If the results show that higher levels of stress are associated with lower levels of job performance, then the researcher can conclude that there is a negative correlation between the two variables.
It is important to note that correlation does not imply causation. While a correlation between two variables indicates that they are related, it does not necessarily mean that changes in one variable are causing changes in the other variable. Therefore, researchers must be cautious when interpreting correlational data and should consider other factors that may be influencing the relationship between variables.
Therefore, the fundamental question addressed by the correlational method is whether two or more variables are related in some systematic way, and researchers must be cautious when interpreting correlational data and should consider other factors that may be influencing the relationship between variables.
To learn more about correlational method here:
brainly.com/question/28476621#
#SPJ11
Five years ago, someone used her $40,000 saving to make a down payment for a townhouse in RTP. The house is a three-bedroom townhouse and sold for $200,000 when she bought it. After paying down payment, she financed the house by borrowing a 30-year mortgage. Mortgage interest rate is 4.25%. Right after closing, she rent out the house for $1,800 per month. In addition to mortgage payment and rent revenue, she listed the following information so as to figure out investment return: 1. HOA fee is $75 per month and due at end of each year 2. Property tax and insurance together are 3% of house value 3. She has to pay 10% of rent revenue for an agent who manages her renting regularly 4. Her personal income tax rate is 20%. While rent revenue is taxable, the mortgage interest is tax deductible. She has to make the mortgage amortization table to figure out how much interest she paid each year 5. In last five years, the market value of the house has increased by 4.8% per year 6. If she wants to sell the house today, the total transaction cost will be 5% of selling price Given the above information, please calculate the internal rate of return (IRR) of this investment in house
Can you show the math as far as formulas go?
Answers
Given the following information: Five years ago, someone used her $40,000 saving to make a down payment for a townhouse in RTP. The house is a three-bedroom townhouse and sold for $200,000 when she bought it. After paying down payment, she financed the house by borrowing a 30-year mortgage.
Mortgage interest rate is 4.25%. Right after closing, she rent out the house for $1,800 per month. In addition to mortgage payment and rent revenue, she listed the following information so as to figure out investment return: 1. HOA fee is $75 per month and due at end of each year 2. Property tax and insurance together are 3% of house value 3. She has to pay 10% of rent revenue for an agent who manages her renting regularly 4. Her personal income tax rate is 20%. While rent revenue is taxable, the mortgage interest is tax deductible. She has to make the mortgage amortization table to figure out how much interest she paid each year 5. In the last five years, the market value of the house has increased by 4.8% per year 6.
To know more about interest visit:
https://brainly.com/question/30393144
#SPJ11
Trigonometry Match the following triangles with their missing values for variable x. ROUND TO THE NEAREST TENTH.

Answers
We have the following:
We can solve the triangles by means of the trigonometric ratios, which relate the hypotenuse with the legs.
The main trigonometric ratios are:
\(\begin{gathered} \sin \theta=\frac{\text{opposite}}{\text{hypotenuse}} \\ \cos \theta=\frac{\text{adjacent}}{\text{hypotenuse}} \\ \tan \theta=\frac{\text{opposite}}{\text{adjacent}} \end{gathered}\)1.
To calculate the first triangle we must use the trigonometric cosine ratio, which relates the hypotenuse with the adjacent leg:
\(\begin{gathered} \cos x=\frac{8}{11} \\ x=\cos ^{-1}(\frac{8}{11}) \\ x=43.3 \end{gathered}\)2.
To calculate the first triangle we must use the trigonometric ratio sine, which relates the hypotenuse with the opposite leg
\(\begin{gathered} \sin x=\frac{15}{24} \\ x=\sin ^{-1}(\frac{15}{24}) \\ x=38.7 \end{gathered}\)3.
To calculate the first triangle we must use the tangent trigonometric ratio, which relates the adjacent leg with the opposite leg
\(\begin{gathered} \tan x=\frac{20}{37} \\ x=\tan ^{-1}(\frac{20}{37}) \\ x=28.4 \end{gathered}\)Rip van Winkle fell asleep for a very long time. When he fell asleep, his beard was 888 millimeters long, and each passing week it grew 222 additional millimeters.
Graph the relationship between the length of Rip van Winkle's beard (in millimeters) and time (in weeks).
Answers
If his beard was 8 millimeter long and each passing week it grew 2 additional millimeters, the graph that represents the relationship between the length of his beard in millimeter and time in week has been plotted
The initial length of the beard = 8 millimeter
The length of beard grow in each week = 2 millimeter
Consider the number of week as x
Therefore the linear relationship will be
The length of the beard y = 2x + 8
Plot the graph using the equation
Hence, If his beard was 8 millimeter long and each passing week it grew 2 additional millimeters, the graph that represents the relationship between the length of his beard in millimeter and time in week has been plotted
The complete question is
Rip van Winkle fell asleep for a very long time. When he fell asleep, his beard was 8 millimeters long, and each passing week it grew 2additional millimeters.
Graph the relationship between the length of Rip van Winkle's beard (in millimeters) and time (in weeks).
Learn more about graph here
brainly.com/question/17267403
#SPJ1

Identify the proof to show that △WZY≅△YXW , where WZ⊥ZY , WX⊥XY , ∠ZYW≅∠XWY , WX≅ZY , and WZ≅XY
Answers
Answer: To show that △WZY ≅ △YXW, we need to show that they are congruent by proving that all corresponding sides and angles are equal.
Given:
WZ⊥ZY and WX⊥XY, which implies ∠WZY = ∠YXW = 90°
∠ZYW ≅ ∠XWY (given)
WX ≅ ZY and WZ ≅ XY (given)
To prove:
△WZY ≅ △YXW
Proof:
By the given information, ∠WZY = ∠YXW = 90°.
By the given information, ∠ZYW ≅ ∠XWY.
Therefore, ∠WZY + ∠ZYW ≅ ∠YXW + ∠XWY. Combining these angles gives us ∠WZY + ∠ZYW = ∠YXW + ∠XWY.
Since the sum of interior angles in a triangle is 180°, we have:
∠WZY + ∠ZYW + ∠YZW = 180° for △WZY
∠YXW + ∠XWY + ∠YWX = 180° for △YXW
Substituting in the angle congruence (∠ZYW ≅ ∠XWY), we have:
∠WZY + ∠XWY + ∠YZW = 180° for △WZY
∠YXW + ∠ZYW + ∠YWX = 180° for △YXW
By the given information, WX ≅ ZY and WZ ≅ XY.
Therefore, by the Side-Angle-Side (SAS) congruence postulate, △WZY ≅ △YXW.
Hence, we have proved that △WZY ≅ △YXW.
Step-by-step explanation:
rate x time = distance how far will a boat travel moving 20 km per hour for 3.5 hours?
Answers
the boat will travel 70 kilometers in 3.5 hours when moving at a rate of 20 kilometers per hour.
To calculate the distance traveled by a boat, we can use the formula: Rate x Time = Distance.
In this case, the rate of the boat is 20 km per hour, and the time is 3.5 hours.
Using the formula, we have:
Distance = Rate x Time
Distance = 20 km/h x 3.5 hours
Calculating the result:
Distance = 70 km
To know more about Distance visit:
brainly.com/question/15256256
#SPJ11
PLEASE HELP< I WILL GIVE YOU BRAINIEST
What are the real and imaginary parts of the complex number?
−6−i
Answers
Imaginary part: -i
The net of a triangular prism is shown. The areas of the individual sides aregiven in the table.SideАВCDEArea10 cm235 cm228 cm235 cm210 cm2AB00DWhat is the lateral surface area?
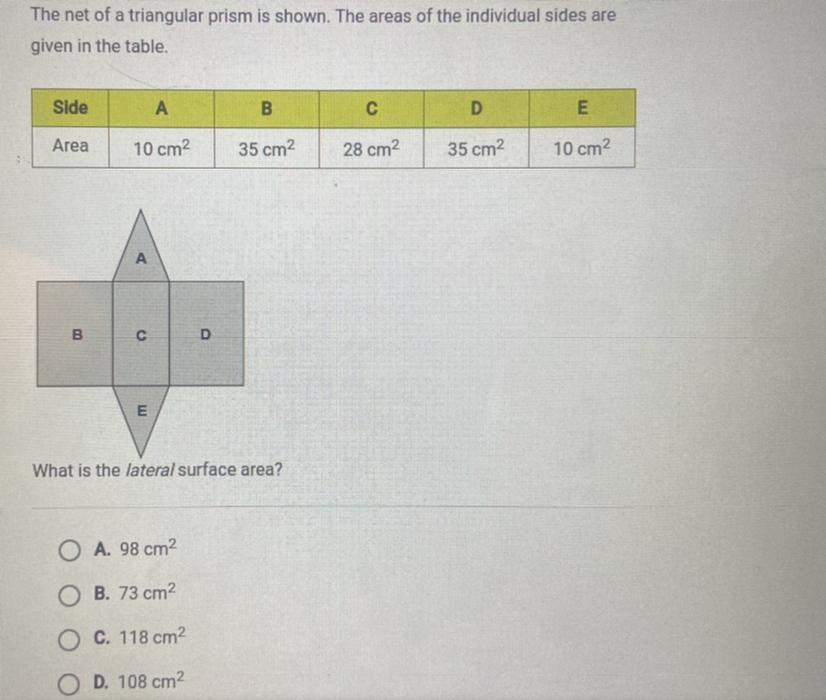
Answers
Answer:
A. 98 cm^2
Explanation:
Given the net of a triangular prism with the area of each face, note that the lateral surface area of the given triangular prism can be determined by adding only the area of the lateral surfaces of the prism, we won't include the area of the bases of the prism.
So the lateral surface area will be the sum of the areas of the rectangles as seen below;
\(\begin{gathered} \text{LSA }=Area_B+Area_C+Area_D \\ =35+28+35 \\ =98cm^2 \end{gathered}\)Therefore, the lateral surface area of the given net of a triangular prism is 98 cm^2
If you take the opposite of the product of 8 and -2, will the answer be less than -5, between -5 and 5 and 10, or greater than 10?
Answers
Answer: Greater than 10.
8x-2=-16 the inverse (opposite) of -16 is 16. 16 is greater than 10.
HELICORRETO BIMESTRAL N° 1 - 2° GRADO - GEOMETRÍA - TM
Answers
According to the angle, the length of the zip wire is approximately 27.27 meters.
In this case, we have a right-angled triangle, where one of the angles is 90 degrees, and the other angle is 10 degrees. The side opposite the 10-degree angle is the length of the zip wire, and the side adjacent to the 10-degree angle is half of the distance between the two posts.
To find the length of the zip wire, we can use the trigonometric function called the tangent. The tangent of an angle is the ratio of the length of the side opposite the angle to the length of the side adjacent to the angle.
So, we can write:
tan(10 degrees) = opposite/adjacent
where the opposite side is the length of the zip wire, and the adjacent side is half of the distance between the two posts, which is 12.5 meters.
Now, we can solve for the length of the zip wire:
opposite = tan(10 degrees) x adjacent
= tan(10 degrees) x 12.5
= 2.1818 (rounded to 4 decimal places) x 12.5
= 27.2727 (rounded to 4 decimal places) meters
To know more about angle here
https://brainly.com/question/4316040
#SPJ4
Complete Question:
A zip wire runs between two posts, 25 m apart. The zip wire is at an angle of 10° to the horizontal. Calculate the length of the zip wire.
please help this is trigonometry

Answers
49 to the nearest integer
Answers
Answer:
The Answer would be 49.
Step-by-step explanation:
Mattie Evans drove 210 miles in the same amount of time that it took a turbopropeller plane to travel 660 miles. The speed of the plane was 150 mph faster than the speed of the car. Find the speed of the plane.
Answers
The speed of the plane is 220 mph.
Let's assume the speed of the car is "x" mph.
Since Mattie Evans drove 210 miles in the same amount of time that it took the plane to travel 660 miles, we can set up the equation:
210 / x = 660 / (x + 150)
To solve for "x," we can cross-multiply:
210(x + 150) = 660x
Distribute and simplify:
210x + 31,500 = 660x
Subtract 210x from both sides:
31,500 = 450x
Divide both sides by 450:
x = 31,500 / 450
x ≈ 70
Therefore, the speed of the car is approximately 70 mph.
To find the speed of the plane, we can substitute this value back into the equation:
Speed of plane = speed of car + 150
Speed of plane = 70 + 150
Speed of plane = 220 mph.
For similar question on speed.
https://brainly.com/question/29723026
#SPJ11
(4x^2-1)*(x+3)-(2x^2-1)(2x+1)=x^2
Answers
Answer: " x = 0.4191113191843072889 ; 0.4191113191843072889 " .
_________________________________________________
Step-by-step explanation:
_________________________________________________
Given:
___________
" (4x² − 1)(x+3) − (2x² − 1)(2x+1) = x² " ; Solve for "x" ;
___________
First, factor:
"(4x² − 1)" ;
= 4 x² − 2x − 2x − 1 ;
→ 2 x(2x +1) − 1(2x +1) ;
= (2x − 1)(2x + 1) .
___________
Now, rewrite:
___________
" (4x² − 1)(x+3) − (2x² − 1)(2x+1) = x² " ;
by substituting for: "(4x² − 1)" ; as follows:
____________________
" (2x − 1)(2x + 1)(x+3) − (2x² − 1)(2x + 1) " ;
= " [(2x − 1)(x + 3) − (2x² − 1)] * (2x +1) = x² ;
___________
Start with:
"(2x − 1)(x + 3)" ;
___________
Note: "(a + b)(c+d) = ac + ad + bc + bd " ;
___________
→ (2x*x) + (2x*3) + (-1*x) + (-1*3) ;
= 2x² + 6x + (-1x) + (-3) ;
= 2x² + 6x − 1x − 3 ;
{Note: "Adding a negative is the same as "subtracting a positive."}.
→ Combine the "like terms":
+ 6x − 1x = + 5x ;
and rewrite:
= 2x² + 5x − 3 ;
Now, rewrite the entire equation:
→ [2x² + 5x − 3 − (2x² − 1)] * (2x +1) = x² ;
Now, consider this equation:
___________
→ [2x² + 5x − 3 − 1(2x² − 1)] * (2x +1) = x² ;
Now, let us consider the:
" − 1(2x² − 1)] " ;
Take note of the "distributive property of multiplication" :
___________
→ a(b + c) = ab + ac ;
___________
→ -1 [2x² + (-1) ] = (-1*2x²) + (-1* -1) ;
= - 2x² + 1 ;
Now, we can rewrite the entire equation:
___________
→ " (2x² + 5x − 3 − 2x² + 1) * (2x +1) = x² " ;
Now, consider the following part:
→ " (2x² + 5x − 3 − 2x² + 1) " ;
→ Combine the "like terms" :
+ 2x² − 2x² = 0 ;
− 3 + 1 = - 2 ;
→ and rewrite this part:
→ " ( 5x − 2) " ;
Now, we can rewrite the entire equation:
___________
→ " (5x − 2) (2x + 1) = x² " ;
___________
Now, for the "left-hand side" of the equation:
___________
Note: "(a + b)(c+d) = ac + ad + bc + bd " ;
___________
→ " (5x − 2) (2x + 1) = (5x*2x) + (5x*1) +(-2*2x) + (-2*1) ;
= 10x² + 5x + (-4x) + (-2) ;
= 10x² + 5x − 4x − 2 ;
→ "Combine the "like terms" :
+ 5x − 4x = + 1x '
→ and rewrite the expression:
= 10x² + 1x − 2 ;
Now, we can rewrite the entire equation:
___________
→ 10x² + 1x − 2 = x² ;
___________
Subtract: "x² " ; from Each Side of the equation:
___________
→ 10x² + 1x − 2 − x² = x² − x² ;
to get:
→ 9x² + 1x − 2 = 0 ; Solve for "x" ;
___________
This equation is written in "quadratic format":
→ " ax² + bx + c = 0 " ; {a ≠ 0} ;
→ in which: " a = 9 ; b = 1 ; c = -2 " ;
To solve for "x" using the "quadratic equation formula"
→ x = {-b ± √(b² − 4ac) } / {2a} ; Plug in our known values:
x = { -1 ± √(1² − 4(9)(-2) } / {2*9} ;
x = { -1 ± √[1 − (-72)} / {18} ;
x = { -1 ± √[1 +72} / {18} ;
x = (-1 ± √73) / 18 ;
___________
" x = (-1 +√73)/18 ; (-1 −√73) / 18 " .
___________
Using calculator:
___________
" x = 0.4191113191843072889 ; 0.4191113191843072889 " .
___________
Hope this helps!
Good luck to you!
___________
9u^2-11u+7=0
Please help
Answers
Answer:
= − 1 3 1
Step-by-step explanation:
No real solutions because the discriminant is negative
The square root of a negative number is not a real number
The coefficient of 4(3)(2)Q is

Answers
Answer:
24
Step-by-step explanation:
4 times 3 is 12
12 times 2 is 24
So the coefficient is 24
Hope this helps!
What is the greatest common factor of the terms in the polynomial
12x4 - 6x2 + 9x2
Answers
Answer:
\(3x^2\)
Step-by-step explanation:
\(12x^4-6x^2+9x^2\)
Let's break down these terms into three parts; our number, our base (variable), and our power (exponent).
First, let's think of a number that could be factored out of the numbers 12, 6, and 9. If you're thinking of the number 3, you'd be correct.
Second, look at the bases. We can see that the variables in these terms are all \(x\), so we can combine that with our number 3.
Third, look at the powers. We can see that the exponent of 2 can be factored out, so let's combine that with our 3x:
\(3x^2\)
To put that in perspective:
\(3x^2(4x^2-2+3)\)
_
To check your work, you can distribute the greatest common factor into the parentheses:
\(3x^2(4x^2-2+3)\)
\(12x^4-6x^2+9x^2\)
among the following, a perfect number a) 6 b)28 c) 625 d) 100
this question comes under more than one correct answer
Answers
Answer:
28 and 6
Step-by-step explanation:
List the factors (excluding itself):
6: 1, 2, 3,
28: 1, 2, 4, 7, 14
Add them up!
1+2+3=6
1+2+4+7+14=28
if they add up to the original number then the number is perfect.
========================================
Explanation:
By definition, a perfect number is one where the factors smaller than itself add to the number in question.
The factors of 6, smaller than 6, are {1,2,3}. We can see that 1+2+3 = 6. Therefore, 6 is a perfect number.
Similarly, 1+2+4+7+14 = 28 showing that 28 is also a perfect number.
------
The factors of 625, that are smaller than 625, are 1,5,25,125. They add to 1+5+25+125 = 156. This isn't equal to 625, so 625 isn't a perfect number.
A similar issue happens with 100 as well. The smaller factors are 1,2,4,5,10,20,25,50, meaning we get a sum of 1+2+4+5+10+20+25+50 = 117, which is too large.
------
The first four perfect numbers are: {6, 28, 496, 8128}
thomas was interested in learning more about the salary of a teacher. he believed as a teacher increases in age, the annual earnings also increases. the age (in years) is plotted against the earnings (in dollars) as shown below. using the best-fit line, approximately how much money would a 45-year-old teacher make?
Answers
The salary of the teacher who is 45 year old according to the best fit line is $50000.
What is best fit line?A straight line that minimizes the gap between it and some data is called a line of best fit.
In a scatter plot containing various data points, a relationship is expressed using the line of best fit.
It is a result of regression analysis and a tool for forecasting indicators and price changes.
The line of best fit is a tool used in finance to find patterns or correlations in market returns across assets or across time.
From the graph the two coordinates of the best fit line are:
(30, 40000) and (70, 70000)
The equation of the line is given as:
y = mx + c
The slope of the equation is given as:
m = (y2 - y1) / (x2 - x1)
m = (70000 - 40000)/ 70 - 30
m = 30000/4
m = 750
Substituting the value of slope and coordinate in the point slope form we have:
y - y1 = m (x - x1)
y - 40000 = 750 (x- 30)
y = 750x + 17500
Here, x is the age and y is the salary.
Substituting x = 45 we have:
y = 750(45) + 17500
y = 50000
Hence, the salary of the teacher who is 45 year old according to the best fit line is $50000.
Learn more about equations here:
https://brainly.com/question/29657992
#SPJ1
The correct question is:

Solve the exponential equation: 4x – 2 = 2x
Answers
Answer:
x=1
Step-by-step explanation:
There is a sale on produce at the local supermarket. Strawberries are ½ price if you buy more than 45 ounces. The scale only measures in pounds. How many pounds would you have to buy to receive the discount
Answers
Answer:
3 pounds
Step-by-step explanation:
a pound is 16oz so 3x16=48
Which postulate can be used to prove the two triangles are congruent?

Answers
Answer:
SAS
Step-by-step explanation:
If two sides and the included and the included angle of one triangle are congruent to two sides and the included angle of another triangle , then the triangles are congruent. The included angle is the angle formed by the two sides.
EF = BC
∠ F = ∠ C
DF = AC
then the two triangles are congruent by the SAS postulate.
Answer:
SAS
Step-by-step explanation:
On triangle one and two, they both start by having a congruent side, then a congruent angle, then another congruent side, thus making these triangles congruent by Side Angle Side.
It can't be AAS because there is only one angle, we know is congruent.
It can't be SSS because there are only two sides, we know are congruent.
It can't be HL because there is no right angle, meaning these aren't right triangles.
Given a circle in the complex plane with a diameter that has endpoints at:
-12 − i and 18 + 15i
Find the center of the circle.
Answers
Answer:
the second part to the question is 17
Step-by-step explanation:
edge 2021 :)
have a lovely day!
The lengths of two sides of a triangle are shown.
Side 1: 8x2 − 5x − 2
Side 2: 7x − x2 + 3
The perimeter of the triangle is 4x3 − 3x2 + 2x − 6.
Part A: What is the total length of the two sides, 1 and 2, of the triangle? Show your work. (4 points)
Part B: What is the length of the third side of the triangle? Show your work. (4 points)
Part C: Do the answers for Part A and Part B show that the polynomials are closed under addition and subtraction? Justify your answer. (2 points)
Answers
Answer:
To find the total length of the two sides, we simply add them together:
Total length = Side 1 + Side 2
Total length = (8x^2 - 5x - 2) + (7x - x^2 + 3)
Total length = -x^2 + 8x^2 - 5x + 7x - 2 + 3
Total length = 7x^2 + 2x + 1
Therefore, the total length of the two sides of the triangle is 7x^2 + 2x + 1.
Step-by-step explanation:
To find the length of the third side of the triangle, we need to use the formula for the perimeter of a triangle:
Perimeter = Side 1 + Side 2 + Side 3
We are given the perimeter of the triangle as 4x^3 - 3x^2 + 2x - 6 and we know the lengths of Side 1 and Side 2. Therefore, we can rewrite the formula as:
4x^3 - 3x^2 + 2x - 6 = (8x^2 - 5x - 2) + (7x - x^2 + 3) + Side 3
Simplifying the right-hand side:
4x^3 - 3x^2 + 2x - 6 = 7x^2 + 2x + 1 + Side 3
Side 3 = 4x^3 - 3x^2 + 2x - 6 - 7x^2 - 2x - 1
Simplifying further:
Side 3 = 4x^3 - 7x^2 - x - 7
Therefore, the length of the third side of the triangle is 4x^3 - 7x^2 - x - 7.
Yes, the answers for Part A and Part B show that the polynomials are closed under addition and subtraction.
Closure under addition means that when two polynomials are added, the result is also a polynomial. In Part A, we added the two polynomials 8x^2 - 5x - 2 and 7x - x^2 + 3 to get the total length of the two sides of the triangle, which is 7x^2 + 2x + 1. Since the total length is also a polynomial, this shows that the polynomials are closed under addition.
Closure under subtraction means that when one polynomial is subtracted from another polynomial, the result is also a polynomial. In Part B, we subtracted the two polynomials 8x^2 - 5x - 2 and 7x - x^2 + 3 from the given perimeter of the triangle, 4x^3 - 3x^2 + 2x - 6, to get the length of the third side of the triangle, which is 4x^3 - 7x^2 - x - 7. Since the length of the third side is also a polynomial, this shows that the polynomials are closed under subtraction.
Therefore, the answers for Part A and Part B demonstrate that the polynomials are closed under addition and subtraction.
(X+4)/2x +(x+20)/3x=3
Answers

a man has 32 coins in his pocket, all of which are dimes and quarters. if the total value of his change is 515 cents, how many dimes and how many quarters does he have?your answer is
Answers
Answer:
19 dimes and 13 quarters
Step-by-step explanation:
Let's start by defining some variables:Let's call the number of dimes the man has "d".
Let's call the number of quarters the man has "q".We know that the man has 32 coins in total, so we can write:
d + q = 32We also know that the total value of the change is 515 cents. Since dimes are worth 10 cents and quarters are worth 25 cents, we can write an equation for the total value in cents:
10d + 25q = 515
We now have two equations with two variables, which we can solve simultaneously. Let's rearrange the first equation to solve for one of the variables:
d = 32 - q
We can substitute this expression for "d" into the second equation:
10(32 - q) + 25q = 515Simplifying and solving for "q", we get:
320 - 10q + 25q = 515
15q = 195
q = 13
So the man has 13 quarters. We can substitute this value into the first equation to find the number of dimes:
d + 13 = 32
d = 19
Therefore, the man has 19 dimes and 13 quarters.
There are 19 dimes and 13 quarters.
Let x represent the number of dimes and y represent the number of quarters.
There are a total of 32 coins, so:
x + y = 32
We know that the total value of the change is 515 cents
. The value of x dimes is 10x cents and the value of y quarters is 25y cents. So:
10x + 25y = 515
We can simplify the first equation by solving for one variable in terms of the other:
x + y = 32x = 32 - y
Now we can substitute this expression for x into the second equation:
10(32 - y) + 25y = 515
Simplify and solve for y:
320 - 10y + 25y = 515
15y = 195y
y = 13
So there are 13 quarters. We can find the number of dimes by substituting y = 13 into x + y = 32:x + 13 = 32x = 19So there are 19 dimes.
So, There are 19 dimes and 13 quarters.
To know more about quarters visit:
https://brainly.com/question/391885?
#SPJ11
Order|2.25|| –1,5), 2, 2, and -2 from least to greatest.
.
Answers
Answer:
-2, -1 ,2 ,2 ,2.22.25 that's the answer