Answers
Answer:
The area of the shaded parts is 160 cm².
Step-by-step explanation:
To find the area of the shaded parts, subtract the area of the unshaded triangle from the area of the outer rectangle.
The formula for the area of a rectangle is:
\(\boxed{A= w \cdot l}\)
where:
w = widthl = lengthTherefore, the area of the outer rectangle is:
\(\begin{aligned}\implies A_{\sf rectangle}&=14 \cdot 16\\&= 224\; \sf cm^2\end{aligned}\)
The formula for the area of a triangle is:
\(\boxed{A=\dfrac{1}{2} bh}\)
where:
b = baseh = heightFrom inspection of the given diagram, the base of the unshaded triangle is 16 cm, and the height is (14 - 6) cm.
Therefore, the area of the unshaded triangle is:
\(\begin{aligned}\implies A_{\sf triangle}&=\dfrac{1}{2} \cdot 16 \cdot (14-6)\\\\&=\dfrac{1}{2} \cdot 16 \cdot 8\\\\&=8 \cdot 8\\\\&=64\; \sf cm^2\end{aligned}\)
Finally, to calculate the area of the shaded parts, subtract the area of the unshaded triangle from the area of the outer rectangle:
\(\begin{aligned} \implies A_{\sf shaded}&=A_{\sf rectangle}-A_{\sf triangle}\\&=224-64\\&=160\; \sf cm^2\end{aligned}\)
Therefore, the area of the shaded parts is 160 cm².
Related Questions
PLEASE HELP ME ASAP!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!

Answers
Answer:
94
Step-by-step explanation:
the sample size of the first survey is 15+26+7 = 48
so, for 48 surveyed teachers we get 15 SUV drivers.
our expectations is to get the same ratio also for other sample sizes (like 300).
15/48 = x/300
x = 15×300 / 48 = 15×75 / 12 = 93.75 ≈ 94
what is the probability that a 5-card straight will be drawn (a straight is 5 cards in a row of any suit) from a standard deck of 52 cards if the first three cards drawn are the 2 of spades, the 4 of diamonds, and the 6 of diamonds?
Answers
The probability that a 5-card straight will be drawn from a standard deck of 52 cards if the first three cards drawn are the 2 of spades, the 4 of diamonds, and the 6 of diamonds is 0.005.
A standard deck has 52 cards.
In this case, three cards are already drawn from the deck, which are the 2 of spades, the 4 of diamonds, and the 6 of diamonds. Thus, remaining number of cards= 49.
Probability of getting first card: 1/4
Probability of getting the second card from same suit= 1/13+ 1/13+ 1/12+ 1/11= 563/1716
Probability of getting the third card from same suit= 1/12+1/12+ 1/11+ 1/10= 472/ 1320
Probability of getting the fourth card from same suit= 1/11+ 1/11+ 1/10+ 1/9= 389/ 990
Probability of getting the fifth card from same suit= 1/10+ 1/10+ 1/9+ 1/8= 314/ 720
Therefore, total probability of the event that a 5-card straight will be drawn,
= (1/4)* (563/1716)* (472/ 1320)* (389/990)* (314/720)
= 0.005
Read more about Deck of Card Questions:
https://brainly.com/question/19202591
#SPJ4
Chen is a lorry driver.
He earns a bonus if he drives at least 2.8 kilometres per litre of fuel.
The figures show some information about Chen's last journey.
Journey time 4.5 hours
Average speed 61 km/h
Amount of fuel used 96 litres
Work out whether Chen earned a bonus for this journey.
Answers
Answer:
Yes, Chen earned a bonus for the journey.
Step-by-step explanation:
1 hour = 61 km
4.5 hrs = 61 * 4.5 = 274.5 km
Now,
96 litres = 274.5 km
1 litre = 274.5/96 km
1 litre = 2.859 = 2.9 km (approximately)
So, he drived more than 2.8 kilometers per litre of fuel.
Answer:
Yes
Step-by-step explanation:
Calculating distance travelled by Chen
Journey time x Average speed4.5 hours x 61 km/h274.5 kmCalculating distance covered per litre of fuel
Distance covered / Amount of fuel used274.5 / 962.86 km/litreThe requirement was 2.8 km/litreTherefore, Chen has earned a bonus for this journeyExpress the following ratio as a fraction then reduce the fraction to the lowest terms
4:6
Answers
Step-by-step explanation:
4:6 as a fraction is 4/6
4/6 in its lowest terms is 2/3
hope this helps
Let R be the region in the first quadrant under the graph of y=1√x=1 for 4≤x≤94≤≤9.
Find the area of R. If the line x=K= divides the region Rinto two regions of equal area, what is the value of K?
Answers
The area of the region R can be determined by calculating the definite integral of 1/√x from 4 to 9. The area is equal to 8/3.
To determine the value of K, we need to divide the area of region R into two regions of equal area. Let R1 be the region in the first quadrant under the graph of y=1/√x from 4 to K and let R2 be the region in the first quadrant under the graph of y=1/√x from K to 9.
We can determine the area of R1 by calculating the definite integral of 1/√x from 4 to K. This is equal to (2√K-2√4). Similarly, we can determine the area of R2 by calculating the definite integral of 1/√x from K to 9. This is equal to (2√9-2√K).
Since we want to divide the region R into two regions of equal area, the two definite integrals must be equal. This means that 2√K-2√4 = 2√9-2√K. Solving for K gives us K = 6.
To know more about area refer here:
https://brainly.com/question/27683633
#SPJ11
which of the following is equivalent to [ (x^ 2 y^ 3 )^ -2/ (x^ 6 y^ 3 z)^3]? worth 60 points!
![which of the following is equivalent to [ (x^ 2 y^ 3 )^ -2/ (x^ 6 y^ 3 z)^3]? worth 60 points!](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/28280hJR7aB4p5RFsoxFSBqVvBJ1UYKP.png)
Answers
Answer:
\(\dfrac{1}{x^{48}y^{36}z^{6}}\)
Step-by-step explanation:
\( (\dfrac{(x^2y^3)^{-2}}{(x^6y^3z)^{2}})^3 = \)
\( = (\dfrac{1}{(x^6y^3z)^{2}(x^2y^3)^{2}})^3 \)
\( = (\dfrac{1}{x^{12}y^6z^{2}x^4y^6})^3 \)
\(= (\dfrac{1}{x^{16}y^{12}z^{2}})^3\)
\(= \dfrac{1}{x^{48}y^{36}z^{6}}\)
Answer:
\(\displaystyle \frac{1}{x^{48}y^{36}z^6}\)
Step-by-step explanation:
\(\displaystyle[\frac{(x^2 y^3)^{-2}}{(x^6 y^3 z)^2 } ]^3\)
\(\displaystyle \frac{(x^2 y^3)^{-6}}{(x^6 y^3 z)^6 }\)
\(\displaystyle \frac{(x^{-12} y^{-18})}{(x^{36} y^{18}z^6 ) }\)
\(\displaystyle \frac{x^{-48} y^{-36}}{z^6 }\)
\(\displaystyle \frac{1}{x^{48}y^{36}z^6}\)
ILL GIVE BRAINLIEST!!
Find the length of AB.
6 in
130°
AB = [ 2 ]in
![ILL GIVE BRAINLIEST!!Find the length of AB.6 in130AB = [ 2 ]in](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/IOfByegHD6UkxQxmzzLUiDgq2UIa9DYD.png)
Answers
Answer:
Length arc AB = 3.14 in
Step-by-step explanation:
Length of arc AB = central angle/360 * 2πr
Central angle = 30°
Radius (r) = 6 in.
Substitute
Length of arc AB = 30/360 * 2*π*6
Length arc AB = 3.14 in. (Nearest tenth)
Answer:
3.14
Step-by-step explanation:
its just right
after working for 25 hours the mermaid $375 after working 40 hours of the mermaid $600 predict how much he would make after 10 hours
Answers
Answer:
after 10 hours she made 150$
Step-by-step explanation:
:)
Answer:
The total amount made after working 10 hours would be $150
Explaining it thoroughly and you will be mark as Brainiest…

Answers
Answer: It says it right there
Step-by-step explanation:
create a video explaning
if we are given a right-tail probability (or area) a and want a measurement m, which function in excel should we use? assume that the mean of the distribution is mu and the standard deviation is sigma.
Answers
The function in Excel that we should use to find a measurement M given a right-tail probability (or area) A is = NORM.INV(1-A, mu, sigma)
In order to find a measurement M given a right-tail probability A in Excel, we can use the NORM.INV function. The NORM.INV function in Excel returns the inverse of the normal cumulative distribution for a given probability.
The formula for NORM.INV function is:
= NORM.INV(1-A, mu, sigma)
where:
A is the right-tail probability (or area)
mu is the mean of the distribution
sigma is the standard deviation
The function returns the measurement M such that the right-tail area from M to infinity under the normal curve is equal to A.
Note - In some versions of Excel, the function may be called NORM.INV.RT instead of NORM.INV.
Read more about standard deviation:
brainly.com/question/24298037
#SPJ4
i need answer please
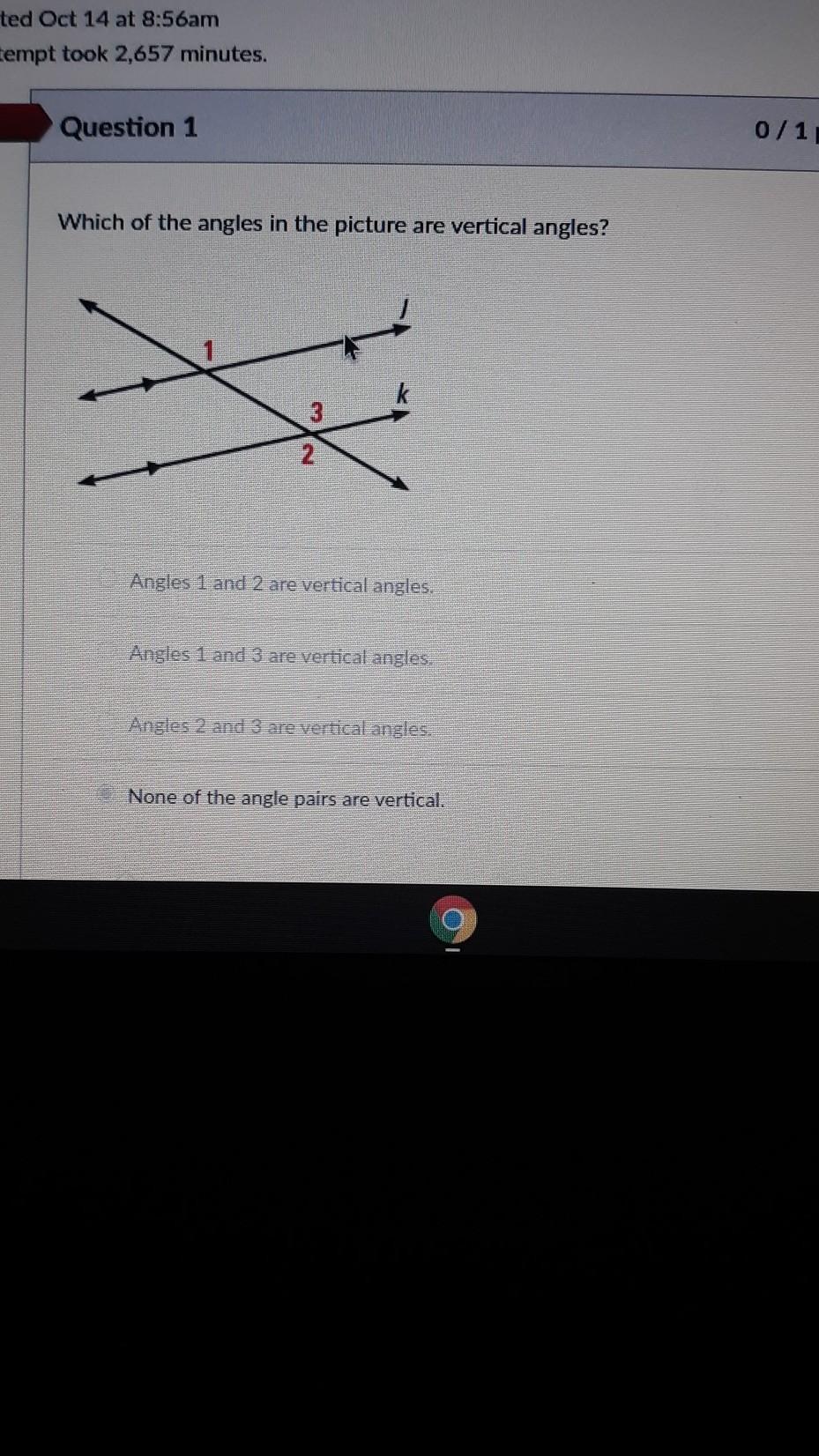
Answers
Answer: Angles 2 and 3 are vertical angles
Step-by-step explanation:
Vertical angles are angles that are not adjacent, and are made by the same 2 lines.
Find the sum of the interior angles for a hexagon. 540° 720° 900° 1,080°
Answers
The sum of interior Angles of Hexagon is always 720°
Any hexagon's internal angles add up to 720° in all cases. By dividing 720° by 6, we may get the size of each interior angle of a regular hexagon. As a result, we have:
720°÷6 = 120°
In a regular hexagon, each inside angle is 120°.
An example of a regular hexagon with equal-length sides and angles is shown in the diagram below. By multiplying the six 120° angles together, we can demonstrate that the result is 720°.
Therefore, the interior angles of a hexagon are always added up to 720°.
Learn more about hexagon :
brainly.com/question/3295271
#SPJ4

Let f(x) = 2x² - 3x and g(x) = 5x - 1.
Find g[f(2)].
g[f(2)] =
Answers
Answer:
Step-by-step explanation:
![Let f(x) = 2x - 3x and g(x) = 5x - 1. Find g[f(2)]. g[f(2)] =](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/Lo55e9uXjNaaQpC1cMKzbxMKNP1qBEAF.png)
To find g[f(2)], we need to evaluate the composite function g[f(2)] by first finding f(2) and then substituting the result into g(x).
Let's start by finding f(2):
f(x) = 2x² - 3x
f(2) = 2(2)² - 3(2)
= 2(4) - 6
= 8 - 6
= 2
Now that we have the value of f(2) as 2, we can substitute it into g(x):
g(x) = 5x - 1
g[f(2)] = g(2)
= 5(2) - 1
= 10 - 1
= 9
Therefore, g[f(2)] is equal to 9.
Learn more about composite function here: brainly.com/question/30660139
#SPJ11
HELP IM DOING THIS RN!

Answers
Answer:
10
Step-by-step explanation:
2. 2. 2
6 +8 = C
36+64=100
Square root 100=10
Answer:
-10
Step-by-step explanation:
here is my explanation i count the distance from store to home for B
and that give me 8^2
for A i count the distance home to school give me 6^2
I add it 6^2 + 8^2 = -10
so C= -10
let me know if is correct
Parallelogram ABCD is rotated to create image A’B’C’D’
Which rule describes the transformation?
O (x, y) > (y, -x)
O (x,y) > (-y,x)
O(x, y) > (-x, -Y)
O (x,y) > (x,-y)
Answers
the awnser to your question is
(x,y)>(y,-x)
Answer:The answer is (x,y) -(y,-x)
Step-by-step explanation:it's
f(r)=3.14r2 what is f(7)?
Answers
Hi :)
f(r) = 3.14r2
f(7) = 3.14(7)(2)
f(7) = 43.96
Use a calculator to find the approximate value of the expression. round the answer to two decimal places. the sine of the complementary angle to 75°.a. 0.65 b. 0.34 c. 0.57 d. 0.26
Answers
Answer: Option d. 0.26
Step-by-step explanation:
Since the addition of complementary angles = 90°
75° + x = 90°
x = 90° - 75 °
x = 15° ( the complementary angle )
sine 15° = 0.25882
= 0.26 correct to two decimal places
84
12
+(5-7²)-72+6-2
Answers
Answer:
8300
Step-by-step explanation:
First, do parenthesis
Then, do the rest of the PEMDAS
Last, you're done!
A cyclist rode 3.75 miles in 0.5 hours. How fast was she going in miles per hour?
1.875 miles per hour
3.75 miles per hour
7.5 miles per hour
14 miles per hour
Answers
Answer:
7.5 miles per hour
Step-by-step explanation:
3.75/.5 = 7.5 miles per hour
Gru acquired 80% of the share capital of Agnes on 1 January 2021 . At that date Agnes had reserves of £30,000.NCl is valued using the proportion of net assets method. At the 31 December 2021 reporting date, the reserves of Gru and Agnes were £400,000 and £50,000 respectively. An impairment of £35,000 to goodwill was identified at the reporting date. What is the group's reserves to present in the consolidated statement of financial position at 31 December 2021? a. £381,000 b. £388,000 c. £405,000 d. £412,000 e. £416,000 f. £450,000 g. None of the above
Answers
f. £450,000 To calculate the group's reserves for the consolidated statement of financial position, we need to consider the equity of both Gru and Agnes, including the impact of the impairment of goodwill.
Gru acquired 80% of Agnes, which implies that Gru's share in Agnes' reserves is 80%. Therefore, Gru's share of Agnes' reserves is £30,000 * 80% = £24,000. The consolidated reserves at the reporting date would be the sum of Gru's reserves (£400,000) and Agnes' reserves (£50,000), excluding the impairment of goodwill (£35,000). Thus, the consolidated reserves are £400,000 + £50,000 - £35,000 = £415,000. However, we need to subtract Gru's share of Agnes' reserves to avoid double counting. Therefore, the group's reserves to present in the consolidated statement of financial position at 31 December 2021 are £415,000 - £24,000 = £391,000.
The group's reserves to present in the consolidated statement of financial position at 31 December 2021 are £450,000.The group's reserves to present in the consolidated statement of financial position at 31 December 2021 is £388,000.
To know more about financial visit:
https://brainly.com/question/32292990
#SPJ11
someone please help
i will give brainliest

Answers
Answer:
its -18 going to the right closed circle
Step-by-step explanation:
Answer: r >_ (greater than or equal to) -18
Step-by-step explanation:
You would place it on -18 :)
You’re welcome :)
Square the binomial (r+s). what is the result?
a) r^2+s^2
b) r^2+2rs+s^2
c) 2r+2s
d) r^2+rs+s^2
Answers
Answer:
answer is b)
\( {(r + s)}^{2} = {r}^{2} + 2rs + {s}^{2} \)
What is the prime factorization of 14.Using exponents when appropriate and order the factors from least to greatest
Answers
Answer:
The Prime Factorization is:
2 x 7
In Exponential Form:
21 x 71
CSV Format:
2, 7
Prime Factors Tree
14
/ \
2 7
Prime[1] = 2, Prime[4] = 7
Step-by-step explanation:
compare this level of uniformity to that of the surface of a table that is 1 meter square. how big would the largest bumps on that table be if its surface were smooth to one part in 100000? express your answer to one significant figure and include the appropriate units.
Answers
If a 1-meter square table were smooth to one part in 100000, the largest bumps on the table would be approximately 10 micrometers tall.
Uniformity is an important concept in various fields of science, including physics and mathematics. When we talk about uniformity, we refer to how much variation or deviation there is in a particular parameter or property. In the context of a surface, uniformity refers to how flat or even the surface is, and how much variation there is in its height or depth.
Now, let's compare the level of uniformity of a surface to that of a table that is 1-meter square. If the surface of the table were smooth to one part in 100000, this means that there would be a maximum deviation of 1/100000 or 0.00001 meters in the height of the surface. In other words, the largest bumps on the table would be 0.00001 meters tall.
To put this in perspective, imagine taking a strand of your hair and dividing it into 100 parts. One of these parts would be approximately the size of the largest bumps on the table. That's how small they would be!
To express this answer to one significant figure, we can round the height of the largest bumps on the table to 0.00001 meters or 10 micrometers (μm), which is a common unit of measurement for surface roughness.
To know more about surface here
https://brainly.com/question/28267043
#SPJ4
Y=5x
A proportional relationship
Answers
Yes, the equation y = 5x represents a proportional relationship
What is Equation?Two or more expressions with an Equal sign is called as Equation.
The given equation is y=5x.
In a proportional relationship, the ratio of y to x is constant.
In the given equation the variable x and y has a proportional relationship.
The equation is in the form of y=mx+b
the ratio of y to x is 5, meaning that for every unit increase in x, y increases by 5 units.
Hence, Yes, the equation y = 5x represents a proportional relationship
To learn more on Equation:
https://brainly.com/question/10413253
#SPJ1
mary just bought a 20-year bond with an 8oupon rate (paid semi-annually) and $1000 par value for $1050. she is expecting an effective annual yield (eay) of: (round to two decimal places.)
Answers
Mary's expected effective annual yield (EAY) is approximately 1.06%.
To calculate the effective annual yield (EAY) of a bond, we need to consider the coupon rate, the purchase price, and the remaining years until maturity.
In this case, Mary bought a 20-year bond with an 8% coupon rate (paid semi-annually) and a $1000 par value for $1050. To calculate the EAY, we can follow these steps:
Calculate the semi-annual coupon payment: 8% of $1000 is $80. Since it is paid semi-annually, the coupon payment for each period is $80/2 = $40.
Calculate the total coupon payments over the 20-year period: There are 20 years, which means 40 semi-annual periods. The total coupon payments will be $40 multiplied by 40, resulting in $1600.
Calculate the total amount paid for the bond: Mary purchased the bond for $1050.
Calculate the future value (FV) of the bond: The future value is the par value of $1000 plus the total coupon payments of $1600, resulting in $2600.
Calculate the EAY using the following formula:
EAY = \((FV / Purchase Price) ^ {(1 / N)} - 1\)
where N is the number of years until maturity.
In this case, N = 20, FV = $2600, and the purchase price is $1050.
Plugging the values into the formula:
EAY = \(($2600 / $1050) ^{ (1 / 20) }- 1\)
Calculating the expression:
EAY = \((2.47619047619) ^ {0.05\) - 1
EAY ≈ 0.0106
Rounded to two decimal places, Mary's expected effective annual yield (EAY) is approximately 1.06%.
To learn more about effective annual yield click on,
https://brainly.com/question/29110740
#SPJ4
What are the y-intercept and the asymptote of g(x) = 3x – 5? (0, –5); y = 3 (0, –2); y = 5 (0, –4); y = –5 (0, 5); y = –3
Answers
The y-intercept of the equation g(x) = 3^x - 5 is (0, -4) and the asymptote of the equation g(x) = 3^x - 5 is y = -5
How to determine the y-intercept?The equation of the function g(x) is given as:
g(x) = 3^x - 5
The y-intercept is a point on the graph where the value of x is 0
This is represented by x= 0 or (0, y)
This means that we substitute 0 for x in the above equation
So, we have:
g(0) = 3^0 - 5
Evaluate the exponent 3^0
g(0) = 1 - 5
Evaluate the difference of 1 and 5
g(0) = -4
Rewrite this point as
(0, -4)
This means that the y-intercept of the equation g(x) = 3^x - 5 is (0, -4)
How to determine the asymptote?The equation of the function g(x) is given as:
g(x) = 3^x - 5
The asymptote is a point on the graph where that is parallel to the graph
In the above equation, we have:
g(x) = 3^x - 5
Express the radical as 0
y = 0 - 5
Evaluate the difference of 0 and 5
y = -5
This means that the asymptote of the equation g(x) = 3^x - 5 is y = -5
Read more about asymptote at:
https://brainly.com/question/1851758
#SPJ1
Clear my choice
Which expression is equivalent to
(-√8 -√2) + √-36
Answers
The expression equivalent to (-√8 -√2) + √-36 is -3√2 + 6i.
What are complex numbers?A real number and an imaginary number are effectively combined to create a complex number. The complex number is written as a+ib, where a and ib are real and imaginary numbers, respectively. Additionally, I = -1 and both a and b are real numbers.
Consequently, a complex number is a straightforward illustration of the addition of two integers, specifically a real number and an imaginary number. It consists of two parts: one that is entirely real, the other entirely fantastical.
The given expression is:
(-√8 -√2) + √-36
The expression can be written as:
(-2√2 - √2) + √(i)²(6)²
Taking the common term:
√2(-2 - 1) + 6i
Simplifying the expression:
-3√2 + 6i
Hence, the expression equivalent to (-√8 -√2) + √-36 is -3√2 + 6i.
Learn more about complex numbers here:
https://brainly.com/question/20566728
#SPJ1
which document do you need to have on hand when preparing to file your tax return? a. auto insurance card b. birth certificate c. driver’s license d. form w-2 e. form w-4
Answers
The document do you need to have on hand when preparing to file your tax return is W-2
What is a tax return?A tax return is a form or forms filed with a tax authority that reports income, expenses, and other pertinent tax information.
To file your return, you'll need the following on hand: Your W-2: This form will come from your company and list not only your salary for the year, but the amount of taxes withheld along the way. You're required to file your W-2 along with your taxes, so if you're a salaried employee, this form is non-negotiable.
For more references on tax returns, click;
https://brainly.com/question/21214035
#SPJ1
Select all expressions that equal the distance between the points on the number line
| 3 | + | -2 |
| -2 + 3 |
| -3 - 2 |
3 - ( -2 )
| 3 - ( -2 ) |

Answers
The expressions that are equal to the distance between point A and point B would be
| 3 | + | -2 |
| -3 - 2 |
3 - ( -2 )
| 3 - ( -2 ) |
What is a number line?
In elementary mathematics, a number line is a picture of a graduated straight line that serves as visual representation of the real numbers.
In the given number line, the distance between point A and point B is 5.
Now we have to check each option
| 3 | + | -2 | = 3 + 2 = 5
| -2 + 3 | = | 1 | = 1
| -3 - 2 | = | -5 | =5
3 - ( -2 ) = 3+ 2 = 5
| 3 - ( -2 ) | = |3 + 2| = 5
Hence, the expressions that are equal to the distance between point A and point B would be
| 3 | + | -2 |
| -3 - 2 |
3 - ( -2 )
| 3 - ( -2 ) |
To learn more about the number line, visit:
https://brainly.com/question/24644930
#SPJ1
