Answers
hopes this helps
Related Questions
143=n-27. PLZ HELP DUE TOMORROW
Answers
Answer:
n=170
Step-by-step explanation:
add 27 to 143
then the equation is n=170
-n= -170
Answer
n=170
Alejandra is packing a bag for a flight. The airline has a baggage weight limit, and Alejandra has already packed her bag with some essentials. All she has left is her outfits and shoes, which must weigh less than 30 pounds total. what is written equation for this situation?
Answers
So its X \(\leq\) 30
Step-by-step explain
The x equals the unknown amount or weight of her luggage.
It has can only be 30 at most
This equation shows that
A number has to be less then or equal to 30
(Chapter 13) The curve r(t)= <0, t^2, 4t> is a parabola
Answers
We can see that the first component of the vector equation is always zero, so the parabola lies in the xz-plane.
Moreover, the second component is a quadratic function of t, which gives us a vertical parabola when plotted in the yz-plane. The third component is a linear function of t, so the curve extends infinitely in both directions. Therefore, we have a vertical parabola in the xz-plane.
This statement is referring to a specific vector-valued function, which we can write as:
f(t) = (0, t^2, ct)
where c is a constant.
The second component of this vector function is t^2, which is a quadratic function of t. When we plot this function in the yz-plane (i.e., we plot y = t^2 and z = 0), we get a vertical parabola that opens upward. This is because as t increases, the value of t^2 increases more and more quickly, causing the curve to curve upward.
The third component of the vector function is ct, which is a linear function of t. When we plot this function in the xz-plane (i.e., we plot x = 0 and z = ct), we get a straight line that extends infinitely in both directions. This is because as t increases or decreases, the value of ct increases or decreases proportionally, causing the line to extend infinitely in both directions.
To know more about parabola,
https://brainly.com/question/28973830
#SPJ11
Please help me out. Please

Answers
julian rolled a normal 6-sided die 12 times. his rolls were as follows: 2, 4, 3, 3, 5, 1, 2, 6, 3, 1, 3, 5, 4. what is the probability that he will roll a 3 on the next roll?
Answers
The probability that Julian will roll a 3 on the next roll is approximately 16.67%. The probability of rolling a 3 on a normal 6-sided die is independent of the previous rolls. This means that regardless of the outcomes of Julian's previous rolls, the probability remains the same.
Explanation
On a 6-sided die, there is 1 favorable outcome for rolling a 3 (the number 3 itself) out of 6 possible outcomes (1, 2, 3, 4, 5, and 6).
To find the probability, you can use the formula:
Probability = (Number of favorable outcomes) / (Total number of outcomes)
In this case:
Probability of rolling a 3 = 1 (favorable outcome) / 6 (total outcomes)
Probability of rolling a 3 = 1/6 ≈ 0.1667 or 16.67%
So, the probability that Julian will roll a 3 on the next roll is approximately 16.67%.
To learn more about probability : brainly.com/question/27292589
#SPJ11
Find the slope of the line below:

Answers
Answer:
Step-by-step explanation:
3
( you will get brainlist and 100 points and a 5.0 and thanks if you do this!!)
Step 2. Identify three (3) regions of the world. Think about what these regions have in common.
Step 3. Conduct internet research to identify commonalities (things that are alike) about the three (3) regions that you chose for this assignment. You should include at least five (5) commonalities. Write a report about your findings.
Answers
Report on Commonalities Among Three Chosen Regions
For this assignment, three regions of the world have been selected to identify commonalities among them. The chosen regions are North America, Europe, and East Asia. Through internet research, several commonalities have been identified that are shared among these regions. Below are five commonalities found:
Economic Development:
All three regions, North America, Europe, and East Asia, are characterized by significant economic development. They are home to some of the world's largest economies, such as the United States, Germany, China, and Japan. These regions exhibit high levels of industrialization, technological advancement, and trade activities. Their economies contribute significantly to global GDP and are major players in international commerce.
Technological Advancement:
Another commonality among these regions is their emphasis on technological advancement. They are known for their innovation, research and development, and technological infrastructure. Companies and industries in these regions are at the forefront of technological advancements in fields such as information technology, automotive manufacturing, aerospace, pharmaceuticals, and more.
Cultural Diversity:
North America, Europe, and East Asia are culturally diverse regions, with a rich tapestry of different ethnicities, languages, and traditions. Immigration and historical influences have contributed to the diversity seen in these regions. Each region has a unique blend of cultural practices, cuisines, art, music, and literature. This diversity creates vibrant multicultural societies and fosters an environment of cultural exchange and appreciation.
Democratic Governance:
A commonality shared among these regions is the prevalence of democratic governance systems. Many countries within these regions have democratic political systems, where citizens have the right to participate in the political process, elect representatives, and enjoy individual freedoms and rights. The principles of democracy, rule of law, and respect for human rights are important pillars in these regions.
Education and Research Excellence:
North America, Europe, and East Asia are known for their strong education systems and institutions of higher learning. These regions are home to prestigious universities, research centers, and educational initiatives that promote academic excellence. They attract students and scholars from around the world, offering a wide range of educational opportunities and contributing to advancements in various fields of study.
In conclusion, the regions of North America, Europe, and East Asia share several commonalities. These include economic development, technological advancement, cultural diversity, democratic governance, and education and research excellence. Despite their geographical and historical differences, these regions exhibit similar traits that contribute to their global significance and influence.
Answer:
For this assignment, three regions of the world have been selected to identify commonalities among them. The chosen regions are North America, Europe, and East Asia. Through internet research, several commonalities have been identified that are shared among these regions. Below are five commonalities found:
Economic Development:
All three regions, North America, Europe, and East Asia, are characterized by significant economic development. They are home to some of the world's largest economies, such as the United States, Germany, China, and Japan. These regions exhibit high levels of industrialization, technological advancement, and trade activities. Their economies contribute significantly to global GDP and are major players in international commerce.
Technological Advancement:
Another commonality among these regions is their emphasis on technological advancement. They are known for their innovation, research and development, and technological infrastructure. Companies and industries in these regions are at the forefront of technological advancements in fields such as information technology, automotive manufacturing, aerospace, pharmaceuticals, and more.
Cultural Diversity:
North America, Europe, and East Asia are culturally diverse regions, with a rich tapestry of different ethnicities, languages, and traditions. Immigration and historical influences have contributed to the diversity seen in these regions. Each region has a unique blend of cultural practices, cuisines, art, music, and literature. This diversity creates vibrant multicultural societies and fosters an environment of cultural exchange and appreciation.
Democratic Governance:
A commonality shared among these regions is the prevalence of democratic governance systems. Many countries within these regions have democratic political systems, where citizens have the right to participate in the political process, elect representatives, and enjoy individual freedoms and rights. The principles of democracy, rule of law, and respect for human rights are important pillars in these regions.
Education and Research Excellence:
North America, Europe, and East Asia are known for their strong education systems and institutions of higher learning. These regions are home to prestigious universities, research centers, and educational initiatives that promote academic excellence. They attract students and scholars from around the world, offering a wide range of educational opportunities and contributing to advancements in various fields of study.
In conclusion, the regions of North America, Europe, and East Asia share several commonalities. These include economic development, technological advancement, cultural diversity, democratic governance, and education and research excellence. Despite their geographical and historical differences, these regions exhibit similar traits that contribute to their global significance and influence.
Please help me thanks
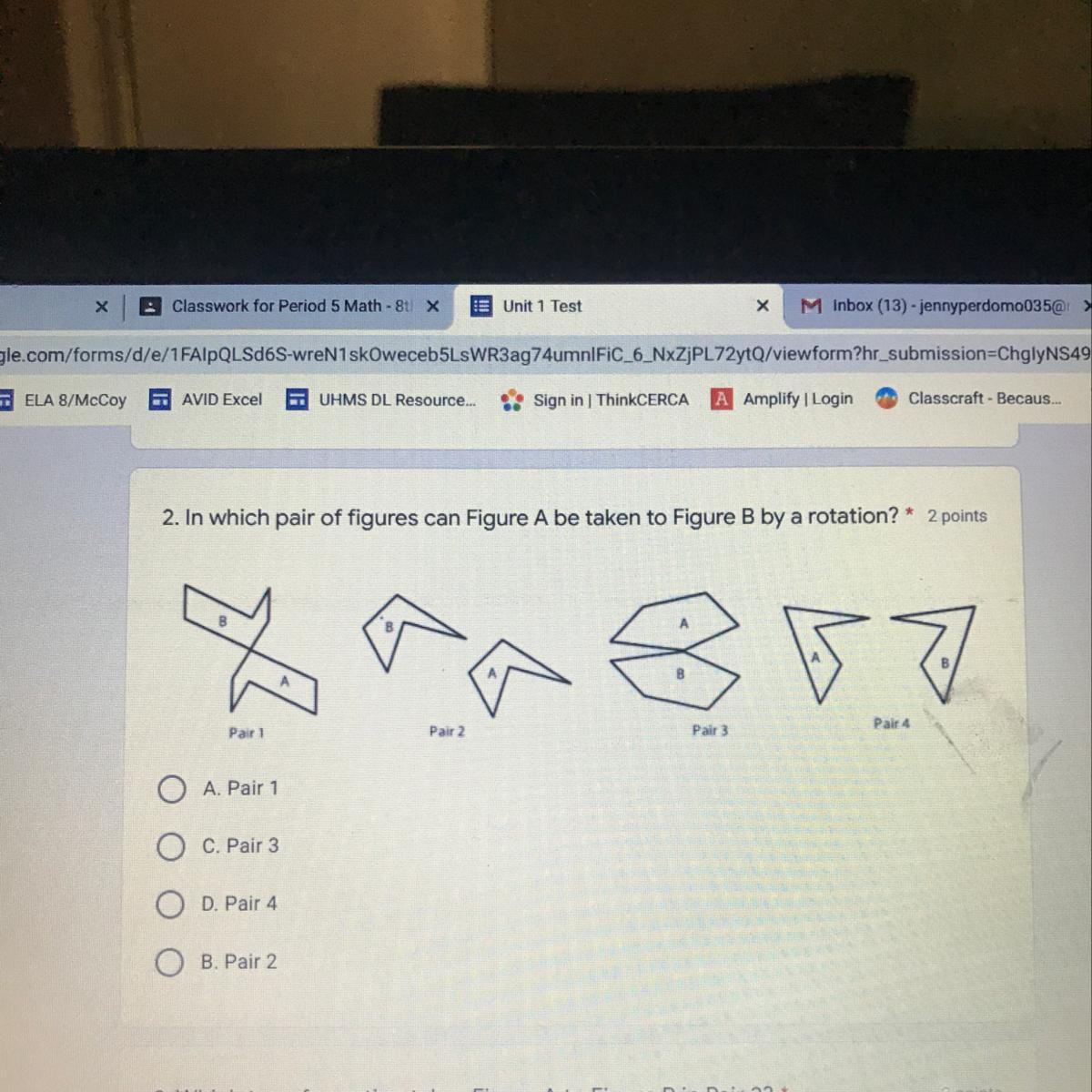
Answers
Answer:
A. Pair 1
Step-by-step explanation:
B is just repositioned, C is a reflection, and D is also a reflection.
he wins 2 blue ball and lose 3 red balls, after 5 days he has the same amount of blue and red balls how many red balls have in the start?.
Answers
If someone wins 2 blue balls and loses 3 red balls every day for 5 days, and ends up with the same number of blue and red balls, then they must have started with 25 red balls.
Explanation:
Let x be the number of red balls the person started with. After 5 days, they would have won 2 × 5 = 10 blue balls and lost 3 × 5 = 15 red balls. So, the person would have a total of (x - 15) red balls and (10 + 2 × 5) = 20 blue balls. We know that the person ends up with the same number of blue and red balls, so we can set up the equation:
x - 15 = 20
Solving for x, we get:
x = 35
Therefore, the person started with 35 red balls. However, this does not satisfy the condition that the person ends up with the same number of blue and red balls, since 35 - 15 = 20 ≠ 20 blue balls. So, we made a mistake in our assumption that the person started with x red balls. Let's try again:
Let y be the number of red balls the person started with. After 5 days, they would have won 2 × 5 = 10 blue balls and lost 3 × 5 = 15 red balls. So, the person would have a total of (y - 15) red balls and 20 blue balls. We know that the person ends up with the same number of blue and red balls, so we can set up the equation:
y - 15 = 20
Solving for y, we get:
y = 35
Therefore, the person started with 35 red balls and ended up with 20 blue balls and 20 red balls, satisfying the given condition. So, the answer is that the person started with 25 red balls.
To learn more about equation click here, brainly.com/question/29538993
#SPJ11
Water freezes at 32°F
Write an inequality for all temperatures that water is NOT frozen
Answers
Answer:
33<x for not being frozen water
Step-by-step explanation:
Question 7 3 point(s) Refer to the diagram below to answer the following questions: (a) What is the midpoint of segment Given: Points S(-2,6) amd T(2,-4) S ST? (b) What is the length of segment ST? T Response *Hint Use maximize tool if images or video cut off BI U TK -LEX show more tools Font Medium 2 x, X A

Answers
We have the following:
The points S(-2,6) and T(2,-4)
a.
the midpoint is:
\(m=(\frac{x_1+x_2}{2},\frac{y_1+y_2}{2})\)replacing:
\(m=(\frac{-2+2}{2},\frac{6+-4}{2})=(0,1)\)b.
the length is segment:
\(d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}\)replacing:
\(\begin{gathered} d=\sqrt[]{(2_{}-(-2)_{})^2+(-4-6_{})^2} \\ d=\sqrt[]{(2_{}+2)^2+(-4-6_{})^2} \\ d=\sqrt[]{(4)^2+(-10)^2} \\ d=\sqrt[]{16+100}=\sqrt[]{116}=10.8 \\ \end{gathered}\)The length is 10.8
Please anwser it ill give brainliest

Answers
Answer:
1st Column: F, D, I
2nd Column: E, C, G
3rd Column: C, G, A
Step-by-step explanation:
1 meter = 100 centimeter
1 centimeter = 10 millimeter
A garden in the shape of an equilateral triangle
has sides whose lengths are 10 meters. What is
the area of the garden?
Answers
Answer:
area is \(25\sqrt{3}\)
Step-by-step explanation:
the hight is \(5\sqrt{3}\)
\(5\sqrt{3}\)*10/2=\(25\sqrt{3}\)
The area of the garden that has a shape of equilateral triangle will be 43.3 square meters.
What is the area of the triangle?Assume 'h' is the height of the triangle and 'b' be the base of the triangle. Then the area of the triangle is given as,
A = (1/2) × h·b
A garden in the shape of an equilateral triangle has sides whose lengths are 10 meters.
The height of the triangle is given as,
sin 60° = h / 10
h = 10 x sin 60°
h = 10 x √3 / 2
h = 5√3 meters
Then the area of the triangle is given as,
A = (1/2) x 10 x 5√3
A = 5 x 5√3
A = 25 x 1.732
A = 43.3 square meters
The area of the garden that has a shape of equilateral triangle will be 43.3 square meters.
More about the area of the triangle link is given below.
https://brainly.com/question/19305981
#SPJ2
Hey I need help on this question thanks Love ya'll so much!

Answers
Answer:
Madeline picks 6 pieces of fruit per hour
Step-by-step explanation:
divide total amount of fruit she picked on that day by the amount of hours she spent picking
12/2 = 6
42/7 = 6
Paige bought 8 bags of sand, she had a total of 30Ib of sand, and each bag cost $1.80/bag: A: What is the weight of sand per bag b) what is the price of sand per Ib
Answers
The weight of sand per bag and price of sand per Ib is 3.75 lb per bag and $1.80 respectively.
Unit priceNumber of bags of sand = 8Total weight of bags of sand = 30 lbCost of each bag of sand = $1.80Weight of sand per bag = Total weight of bags of sand ÷ Number of bags of sand
= 30 lb / 8
= 3.75 lb per bag
price of sand per Ib = $1.80
Learn more about unit price:
https://brainly.com/question/14286952
#SPJ1
Planet x is 7 light-years away from earth. planet y is 5 2/3 light-years away from earth. how much farther away is planet x?
Answers
The distance of planet x from the earth in kilometers is 63000000000 km.
What is light-year?Light-year is the distance light travels in one year. Light zips through interstellar space at 186,000 miles (300,000 kilometers) per second and 5.88 trillion miles (9.46 trillion kilometers) per year.
For most space objects, we use light-years to describe their distance. A light-year is the distance light travels in one Earth year. One light-year is about 6 trillion miles (9 trillion km).
Since one light-year is 9 × 10⁹ km
The distance of planet x is 7 light-year from earth.
Therefore;
7 × 9× 10⁹
= 63× 10⁹km
= 63000000000 km
learn more about light-year from
https://brainly.com/question/76984
#SPJ4
Guys please help me which one is the correct answer.

Answers
Answer:
I think 3 terms
I hope it's helps you
Answer:
2
Step-by-step explanation:
the letters count as term basically
PLEASE HELPP (check my work)

Answers
1.a) The total revenue received for CD sales was $12,090,000.
1.b) The total revenue received for downloads was $2,499,000.
1.c) The total amount that Craze-K received in royalties last year was $1,240,065.
2) The total royalties that Mr. Pambruccian received last year were $45,210.01.
How the total figures were determined:a) Rap Singer:Commission = 8.5%
CDs Downloads Total
Number of items sold 1.86 million 2.1 million
Selling price per unit $6.50 $1.19
Total sales revenue $12,090,000 $2,499,000 $14,5898,000
(1.86 m x $6.50) (2.1 m x $1.19)
Sales commission = $1,027,650 $212,415 $1,240,065
b) Books:Royalties = 4%
Selling price per book = $45.99
The total number of books sold = 24,576
The total revenue (sales) = $1,130,250,24 (24,576 x $45.99)
Royalties received = $45,210.01 ($1,130,250.24 x 4%)
Learn more about the total revenue at https://brainly.com/question/25623677.
#SPJ1
A white dwarf star of \( 1.2 \) solar masses and \( 0.0088 \) solar radii, will deflect light from a distance source by what angle (in aresecs)? Round to TWO places past the decimal
Answers
The deflection angle of light by the white dwarf star is approximately \(\(0.00108 \times 206,265 = 223.03\)\)arcseconds (rounded to two decimal places).
To calculate the deflection angle of light by a white dwarf star, we can use the formula derived from Einstein's theory of general relativity:
\(\[\theta = \frac{4GM}{c^2R}\]\)
where:
\(\(\theta\)\) is the deflection angle of light,
G is the gravitational constant \((\(6.67430 \times 10^{-11} \, \text{m}^3 \, \text{kg}^{-1} \, \text{s}^{-2}\)),\)
M is the mass of the white dwarf star,
c is the speed of light in a vacuum \((\(299,792,458 \, \text{m/s}\)),\) and
(R) is the radius of the white dwarf star.
Let's calculate the deflection angle using the given values:
Mass of the white dwarf star, \(\(M = 1.2 \times \text{solar mass}\)\)
Radius of the white dwarf star, \(\(R = 0.0088 \times \text{solar radius}\)\)
We need to convert the solar mass and solar radius to their respective SI units:
\(\(1 \, \text{solar mass} = 1.989 \times 10^{30} \, \text{kg}\)\(1 \, \text{solar radius} = 6.957 \times 10^8 \, \text{m}\)\)
Substituting the values into the formula, we get:
\(\[\theta = \frac{4 \times 6.67430 \times 10^{-11} \times 1.2 \times 1.989 \times 10^{30}}{(299,792,458)^2 \times 0.0088 \times 6.957 \times 10^8}\]\)
Evaluating the above expression, the deflection angle \(\(\theta\)\) is approximately equal to 0.00108 radians.
To convert radians to arcseconds, we use the conversion factor: 1 radian = 206,265 arcseconds.
Therefore, the deflection angle of light by the white dwarf star is approximately \(\(0.00108 \times 206,265 = 223.03\)\)arcseconds (rounded to two decimal places).
Hence, the deflection angle is approximately 223.03 arcseconds.
Learn more about radius here:
https://brainly.com/question/14963435
#SPJ11
Evaluate The Given Integral By Making An Appropriate Change Of Variables. ∬R56x−Yx−5ydA,Where R Is The Parallelogram
Answers
The value of the given integral by making an appropriate change of variables is 365/6.
We have to evaluate the given integral by making an appropriate change of variables. The integral is:∬R56x−Yx−5ydA, where R is the parallelogram.
We will make the change of variable as:
u = x - y and v = x - 5
The Jacobian of transformation is ∂(u, v) / ∂(x, y) = (1 -1 / 0 1) = 1
The limits of the integration can be found as:
For x = 0, v = -5 and u = 0 - y = -y, or y = -u
For x = 6, v = 1 and u = 6 - y, or y = 6 - u
So, the limits of the integration can be written as:
∬R56x−Yx−5ydA = ∬R'5uv(u+v)dvdu
Where R' is the region in the uv plane obtained by applying the transformation.
The limits of the integration in R' are:
0 ≤ u ≤ 5
-5 + u ≤ v ≤ 1 + u
Now, we can evaluate the integral:
∬R56x−Yx−5ydA = ∬R'5uv(u+v)dvdu
= ∫₀⁵ ∫_{-5+u}^{1+u} 5uv(u+v)dvdu
= 365/6
Therefore, the value of the given integral by making an appropriate change of variables is 365/6.
Learn more about Jacobian of transformation here:
https://brainly.com/question/30887183
#SPJ11
Select the correct answer. The sum of two consecutive numbers is 157. This equation, where n is the first number, represents the situation: 2n + 1 = 157. What is the first number? A. 77 B. 78 C. 79 D. 80
Answers
Answer:
\(n=78\)
Step-by-step explanation:
Step - \(b \times y - s\) step explanations
\(2n + 1 = 157\)
\(2n = 157 - 1\)
\(2n = 156\)
\(n = 156\div2\)
\(\bold{n = 78}\)
3. Select all the expressions that are
equivalent to -3/4 (32 +24e-4f).
A. 24+ 18e - 3f
B.-18e + 3f - 24
C. -24 18e + 3f
D. 8-6e- f
E. 3(-8-6e+f)
Answers
Answer: E only
Step-by-step explanation:
calculate log 1.125 please
Answers
Answer:
0.05115
Step-by-step explanation:
Plug it into your calculator and you'll get the same value
PLEASE HELP ASAP!!!
1) what is the measure of <4? Explain
2) what is the sum of the measures of angles in a triangle?
3) <1 is in a triangle <4 and 53 angle. What is the sum of those two angles?
4) what is the measure of <1 explain
5) find the measure of <2 explain how you found it
6) what is the measure of <3 explaining
I will give Brainlist!!!

Answers
Answer:
1. 90 degrees because of supplementary angles
2. 180 degrees
3. 90 degrees
4. 37 degrees, since the sum of the interior angles add to 180, 180-90-53 = 37.
5. 127 degrees, supplementary angles 180-53
6 . 23 degrees, use the triangle sum theorem 180-30-127
5. (16 marks) It is given that the moment generating function of a negative binomial random variable is mx(t)= (1 - p)^r /(1 - pe^t)^r where p and r are the parameters. Find the expected value and variance using the moment generating function.
Answers
To find the expected value and variance of a negative binomial random variable with moment generating function mx(t) = (1 - p)^r / (1 - pe^t)^r, we need to use the following formulas:
The nth moment of a random variable is given by mx^(n)(0), the nth derivative of the moment generating function evaluated at t = 0.
The expected value of a random variable is given by mx^(1)(0).
The variance of a random variable is given by mx^(2)(0) - [mx^(1)(0)]^2.
Using these formulas, we can find the expected value and variance of the negative binomial random variable.
First, let's find the first two derivatives of the moment generating function:
mx'(t) = r(1-p)^rpe^t / (1-pe^t)^(r+1)
mx''(t) = r(1-p)^rpe^t(r+1-pe^t) / (1-pe^t)^(r+2)
Now we can evaluate the moment generating function and its derivatives at t = 0 to find the expected value and variance:
mx(0) = (1 - p)^r / (1 - p)^r = 1
mx'(0) = r(1-p)^r p / (1-p)^(r+1)
mx''(0) = r(r+1)(1-p)^r p / (1-p)^(r+2) + r(1-p)^r p / (1-p)^(r+1)
Using the formulas for the expected value and variance, we have:
E[X] = mx'(0) = r(1-p) / p
Var[X] = mx''(0) - [mx'(0)]^2 = r(1-p) / p^2
Therefore, the expected value of the negative binomial random variable is E[X] = r(1-p) / p, and the variance is Var[X] = r(1-p) / p^2, where p and r are the parameters of the distribution.
Learn more about binomial random variable here:
https://brainly.com/question/31311574
#SPJ11
What is (2,6) reflected over the y-axis?
Answers
Answer:
(-2,6)
Step-by-step explanation:
please help me asap

Answers
Answer:
y = 2, 5, 8, 11, 14
Step-by-step explanation:
For this question, it's simply asking us to solve for y, at given values of x. So everytime we just need to substitute the given value for x and solve for y.
x + y = 8
When x = 6,
6 + y = 8
substract 6 from both sides:
-6 + 6 + y = 8 - 6
y = 2
When x = 3
3 + y = 8
y = 8 - 3
y = 5
When x = 0
0 + y = 8 --> 0 + y = y
y = 8
When x = - 3
- 3 + y = 8
y = 8 + 3
y = 11
When x = - 6
-6 + y = 8
y = 8 + 6
y = 14
I hope this makes sense :)
By what degree might a variable without a clear operational definition affect statistics performed on it?
Results could be totally different.
grades are an example of a sample.
Samples are chosen at random from the population.
Answers
A variable without a clear operational definition can greatly impact the accuracy and reliability of statistics performed on it. To ensure accurate and meaningful results, it is important to have clear, well-defined variables in any research study.
The lack of a clear definition can lead to inconsistencies in data collection, making it difficult to accurately interpret and analyze the results.
Step 1: When a variable has no clear operational definition, researchers may measure or interpret it in different ways, leading to inconsistencies in data collection.
Step 2: These inconsistencies can affect the reliability and validity of the collected data, which in turn impacts the accuracy of the statistical analysis performed.
Step 3: As a result, the findings from such analyses may not accurately represent the true relationships or patterns within the sample or the population, leading to incorrect conclusions.
To learn more about statistical analysis : brainly.com/question/30591800
#SPJ11
what is the circumference of a circle with diameter 14-inches long
Answers
Answer:
the circumstance is 43.98
Will a large-sample confidence interval be valid if the population from which the sample is taken is not normally distributed? explain
Answers
A normal distribution is a type of continuous probability distribution for a real-valued random variable in statistics.
Yes, the large-sample confidence interval will be valid.
What is meant by normal distribution?A normal distribution is a type of continuous probability distribution for a real-valued random variable in statistics.
The normal distribution, also known as the Gaussian distribution, is a symmetric probability distribution about the mean, indicating that data near the mean occur more frequently than data far from the mean.
The confidence interval will be valid regardless of the shape of the population distribution as long as the sample is large enough to satisfy the central limit theorem.
What does a large sample confidence interval for a population mean?A sample is considered large when n ≥ 30.
By 'valid', it means that the confidence interval procedure has a 95% chance of producing an interval that contains the population parameter.
To learn more about normal distribution, refer to:
brainly.com/question/23418254
#SPJ4
