Ida is trying choose between three different Linear Algebra textbooks. She knows that they have received the following reviews online. 10 is the highest review: Textbook A: 2, 6, 6, 7, 7, 8, 9 Textbook B: 4, 4, 6, 8, 8, 9 10 Textbook C: 5, 5, 6, 6, 6, 7, 10 Which textbook does she choose if she chooses the text with the highest mean review? [ Select ] Which textbook does she choose if she excludes the outlier and then chooses the text with the highest mean review? [ Select ] Does the set of data with the largest range contain an outlier? [ Select ]

In quadrant IV, \(\cos(A)\) is positive. So

\(\sin^2(A) + \cos^2(A) = 1 \implies \cos(A) = \sqrt{1-\sin^2(A)} \approx 0.6258\)

Then by the definition of tangent,

\(\tan(A) = \dfrac{\sin(A)}{\cos(A)} \approx \dfrac{-0.78}{0.6258} \approx \boxed{-1.2465}\)

Answer:

To the nearest hundredth, this is 30.79 units

Step-by-step explanation:

To find the length of the arc, we simply apply the length of arc formula

Mathematically, that would be;

theta/360 * 2 * pi * r

Theta here is 98

r is 18 units

So the length of the arc will be;

98/360 * 2 * 22/7 * 18

= 30.7876 units

to the nearest hundredth, this is;

30.79 units

We are 99% confident that the true average stance duration among elderly individuals lies within the range of 744.56 ms to 857.44 ms.

To test whether the true average stance duration is larger among elderly individuals than among younger individuals, we can perform a one-tailed independent samples t-test. The null hypothesis (H0)

Using the t-test, we compare the means and standard deviations of the two samples and calculate the test statistic

a) To calculate a 99% confidence interval for the true average stance duration among elderly individuals, we can use the sample mean, sample standard deviation, and the t-distribution.

Given:

Older adults: n = 28, sample mean = 801, sample standard deviation = 117

Using the formula for a confidence interval for the mean, we have:

Margin of error = t * (sample standard deviation / √n)

Since the sample size is relatively large (n > 30), we can use the z-score instead of the t-score for a 99% confidence interval. The critical z-value for a 99% confidence level is approximately 2.576.

Calculating the margin of error:

Margin of error = 2.576 * (117 / √28) ≈ 56.44

The confidence interval is then calculated as:

Confidence interval = (sample mean - margin of error, sample mean + margin of error)

Confidence interval = (801 - 56.44, 801 + 56.44) ≈ (744.56, 857.44)

b) To test whether the true average stance duration is larger among elderly individuals than among younger individuals, we can perform a one-tailed independent samples t-test.

The null hypothesis (H0): The true average stance duration among elderly individuals is equal to or less than the true average stance duration among younger individuals.

The alternative hypothesis (Ha): The true average stance duration among elderly individuals is larger than the true average stance duration among younger individuals.

. With the given data, perform the t-test and obtain the p-value.

c) To construct a 95% confidence interval for the difference in means between older and younger adults, we can use the formula for the confidence interval of the difference in means.

Given:

Older adults: n1 = 28, sample mean1 = 801, sample standard deviation1 = 117

Younger adults: n2 = 16, sample mean2 = 780, sample standard deviation2 = 72

Calculating the standard error of the difference in means:

Standard error = √((s1^2 / n1) + (s2^2 / n2))

Standard error = √((117^2 / 28) + (72^2 / 16)) ≈ 33.89

Using the t-distribution and a 95% confidence level, the critical t-value (with degrees of freedom = n1 + n2 - 2) is approximately 2.048.

Calculating the margin of error:

Margin of error = t * standard error

Margin of error = 2.048 * 33.89 ≈ 69.29

The confidence interval is then calculated as:

Confidence interval = (mean1 - mean2 - margin of error, mean1 - mean2 + margin of error)

Confidence interval = (801 - 780 - 69.29, 801 - 780 + 69.29) ≈ (-48.29, 38.29)

Comparison with part (b): In part (b), we performed a one-tailed test to determine if the true average stance duration among elderly individuals is larger than among younger individuals. In part (c), the 95% confidence interval for the difference in means (-48.29, 38.29) includes zero. This suggests that we do not have sufficient evidence to conclude that the true average stance duration is significantly larger among elderly individuals compared to younger individuals at the 95% confidence level.

Know more about 99% confident here:

https://brainly.com/question/30762304

#SPJ11

Answer:

options b is correct

Does the answer help you?

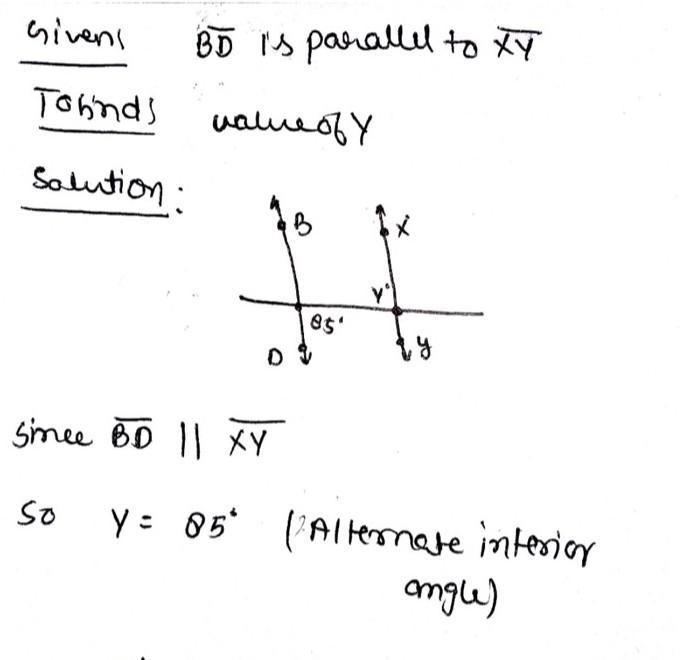
Answer:

B. \(85\)

Explanation:

This is a simple test of your knowledge about this sort of problem. When you get 2 parallel lines intersected the way you see in the picture, the lower right angle of the first intersection is equal to the upper left angle of the first line as well as the upper left and lower right angles of the second line so with that knowledge you can immediately identify that since the question gives you the measure of an angle equal to the angle you need to identify; you don't even need to do any math to find the answer for this question.

The point that is not within the range of the radio transmitter tower is

(55, 185).

The range of the radio transmitter tower is given as 175 miles. Out of the five given points, the distance between the origin and point (55, 185) is calculated as sqrt(55² + 185²) = 192.3 miles which is greater than the range of the tower. Therefore, this point is not within the range of the tower.

On the other hand, the distance between the origin and the other four points (40, 120), (60, 125), (85, 100), and (90, 150) are calculated to be 124.6 miles, 131.8 miles, 141.4 miles, and 159.8 miles respectively which are all less than the range of the tower. Hence, these four points are within the range of the radio transmitter tower.

In conclusion, out of the five given points, only the point (55, 185) is not within the range of the radio transmitter tower, which can be received up to 175 miles away.

Learn more about Range:

brainly.com/question/29204101

#SPJ11

The car travelled an arc length of 7.85 ft (approx) to the nearest hundredth. Therefore, the option (B) 4.31 feet is incorrect. The correct option is (D) 6.63 feet.

Given, d = 20 ft Formula used: We know that Arc Length (S) = rΘ where Θ is in radians. In degrees, the formula can be modified as S = (ΠrΘ)/180.Here, d = 20 ft or r = 10 ft. Since 20 ft is the diameter, we divide it by 2 to get the radius. Now, we are to calculate the arc length.

To calculate arc length, we need Θ.Let’s try to find Θ.We know that the circumference of the circle is C = 2Πr. Here, the diameter is 20ft. Thus, r = 20/2 = 10 ft.

Circumference of the circle, C = 2Πr= 2Π × 10= 20ΠThe distance travelled by the car is 1/8th of the circumference, as it has to travel one-eighth of the circle.

1/8th of the circumference = (1/8)×(20Π) ft= 2.5Π ft≈ 7.85398 ft (to the nearest hundredth). Thus, the car travelled an arc length of 7.85 ft (approx) to the nearest hundredth.

Therefore, the option (B) 4.31 feet is incorrect. The correct option is (D) 6.63 feet.

To know more about Length  visit :

https://brainly.com/question/2497593

#SPJ11

Step-by-step explanation:

(x + 2)² - 5 = 7

Once in standard form, identify a, b and c from the original equation and plug them into the quadratic formula.

○ x = -2± √12

Answer:

no solution..........

Answer:

5:2

Step-by-step explanation:

This means there are 5 red apples as long as there are 2 green apples.

The midpoint of AB is M(4,1), If the coordinates of A are (2,8), then the coordinates of B are (6,-6).

The midpoint formula states that the midpoint of a line segment in a coordinate plane is given by the average of the x-coordinates and the average of the y-coordinates.

The midpoint of AB is M(4,1) and the coordinates of A are (2,8).

Therefore, we can write the following equation: x-coordinate of the midpoint = (x-coordinate of A + x-coordinate of B) / 2y-coordinate of the midpoint = (y-coordinate of A + y-coordinate of B) / 2

Substituting the given values into the above equation, we get:4 = (2 + x-coordinate of B) / 2 1 = (8 + y-coordinate of B) / 2

Simplifying the equations above, we get: x-coordinate of B = 6 y-coordinate of B = -6

Therefore, the coordinates of B are (6,-6).

For more questions on midpoint

https://brainly.com/question/28667736

#SPJ8

The amount of liquid that can be processed in 2 hours at a unit rate of 1 gallon per 18.25 minutes is calculated to be approximately 6.58 gallons.

Assuming the unit rate of 1 gallon per 18.25 minutes, we can convert 2 hours to minutes by multiplying it by 60, which gives us 120 minutes.

So, in 120 minutes, the amount of liquid that can be processed at a unit rate of 1 gallon per 18.25 minutes would be:

(120 minutes) / (18.25 minutes/gallon) = 6.58 gallons

Therefore, the amount of liquid that can be processed in 2 hours at a unit rate of 1 gallon per 18.25 minutes is found out to be  approximately 6.58 gallons.

Learn more about Time and  rate :

https://brainly.com/question/24353923

#SPJ4

The complete question is :

What is the amount of liquid that can be processed in 2 hours at a unit rate of 1 gallon per 18.25 minutes?

Take the equation and distribute the 16 on the right:

    64x - 16 = 16(4x - 1)

    64x - 16 = 64x - 16

Since what is on both sides is identical, you'll always get a true statement no matter what value you pick for x.

This equation has infinitely many solutions.

In school suspension

The system can be written as a vector equation as [5, 1, -3] [x1, x2, x3]^T = [8, 0]^T and as a matrix equation as AX = B, where A = [5 1 -3; 0 2 4], X = [x1; x2; x3], and B = [8; 0].

To write the given system as a vector equation, we group the variables and the constants into vectors and write the equations in a matrix form. Thus, the system can be written as [5x1 + x2 - 3x3; 2x2 + 4x3] = [8; 0], which is a vector equation.

To write the system as a matrix equation, we can write the coefficients of the variables in a matrix A, the variables in a vector X, and the constants in a vector B. Thus, the system can be written as AX = B, where A = [5 1 -3; 0 2 4], X = [x1; x2; x3], and B = [8; 0].

We can then solve for X by finding the inverse of A and multiplying both sides of the equation by it.

To know more about vector equation, refer here:

https://brainly.com/question/30561769#

#SPJ11

Step-by-step explanation:

please mark me as brainlest

The infinite geometric series -10, -20, -40, ... diverges when it is obtained by multiplying the previous term by -2.

An infinite geometric series converges if the absolute value of the common ratio (r) is less than 1. In this case, the common ratio is -2 (-20 divided by -10), which has an absolute value of 2. Since the absolute value of the common ratio is greater than 1, the series diverges.

To further understand why the series diverges, we can examine the behavior of the terms. Each term in the series is obtained by multiplying the previous term by -2. As we progress through the series, the terms continue to grow in magnitude. The negative sign simply changes the sign of each term, but it doesn't affect the overall behavior of the series.

For example, the first term is -10, the second term is -20, the third term is -40, and so on. We can see that the terms are doubling in magnitude with each successive term, but they never approach a specific value. This unbounded growth indicates that the series does not have a finite sum and therefore diverges.

Learn more about geometric series here:

https://brainly.com/question/30264021

#SPJ11

Answer:

80°

Step-by-step explanation:

both triangles are scalene triangle and the have equal properties

angles in triangles sum up to 180°

30°+70° +D=180°

D=180-100=80°

Answer:

45

Step-by-step explanation:

The slope of the line in the example is 25 and the y-intercept is 10. These values can be used to write the equation of the line in slope-intercept form, y = 25x + 10.

The slope, m, represents the rate of change of the height of the giant kelp plant. In this case, the slope is 25, meaning that for every day that passes, the height of the giant kelp plant increases by 25 centimeters.

The y-intercept, b, represents the height of the giant kelp plant at time = 0. In this case, the y-intercept is 10, meaning that the giant kelp plant is 10 centimeters tall at time = 0.

So in this situation the slope represents how much the height of the kelp plant changes with respect to time and the y-intercept represents the initial height of the kelp plant at time = 0.

Answer:

g(x) = - (7x + 4)

or

g(x) = -7x - 4

Step-by-step explanation:

When a function f(x) is reflected over the x axis the reflected function g(x) = -f(x)

Given the coordinates of the intersection point, the bearing of the tangent length TI, and the coordinates of the control points, we can perform the necessary calculations to find the desired coordinates, bearings, and distances.



i) To calculate the coordinates of the points on the centerline of the curve at exact 25-meter multiples of the through chainage, we start with the coordinates of the intersection point (2000.000mE, 4000.000mN) and the bearing of the tangent length TI (132° 45'30").

We can use the formula: x = x0 + R * sinθ and y = y0 + R * cosθ, where x0 and y0 are the coordinates of the intersection point, R is the radius of the curve, θ is the angle in radians.

First, we need to calculate the radius R of the circular curve. This can be done using the formula: R = (Lc / 2) / sin(A / 2), where Lc is the length of the curve and A is the deflection angle of the curve.

Since the curve is not explicitly mentioned in the question, we will assume a specific length for demonstration purposes. Let's assume Lc = 100 meters and A = 30°.

Now, we can calculate the radius R using the formula: R = (100 / 2) / sin(30° / 2) ≈ 100.93 meters.

Using this radius, we can find the coordinates of points on the centerline of the curve at exact 25-meter multiples of the through chainage by incrementing the angle θ by the appropriate amount and calculating the corresponding x and y coordinates.

ii) To calculate the bearings and distances required to set out the circular curve from Control Point A (1959.00mE, 4002.00mN) and Control Point B (2040.00mE, 4015.00mN), we need to determine the bearing and distance from each control point to the center of the curve.

Using the coordinates of the control points and the coordinates of the intersection point, we can calculate the bearing using the formula: θ = atan2(y2 - y1, x2 - x1), where (x1, y1) and (x2, y2) are the coordinates of the control point and the intersection point, respectively.

The distance can be calculated using the formula: d = sqrt((x2 - x1)^2 + (y2 - y1)^2).

By applying these formulas to the given control points and intersection point, we can determine the bearings and distances required to set out the circular curve from Control Point A and Control Point B.

Learn more about curve here : brainly.com/question/30958460

#SPJ11

Given the coordinates of the intersection point, the bearing of the tangent length TI, and the coordinates of the control points.

We can perform the necessary calculations to find the desired coordinates, bearings, and distances. i) To calculate the coordinates of the points on the centerline of the curve at exact 25-meter multiples of the through chainage, we start with the coordinates of the intersection point (2000.000mE, 4000.000mN) and the bearing of the tangent length TI (132° 45'30").

We can use the formula: x = x0 + R * sinθ and y = y0 + R * cosθ, where x0 and y0 are the coordinates of the intersection point, R is the radius of the curve, θ is the angle in radians. First, we need to calculate the radius R of the circular curve. This can be done using the formula: R = (Lc / 2) / sin(A / 2), where Lc is the length of the curve and A is the deflection angle of the curve.

Since the curve is not explicitly mentioned in the question, we will assume a specific length for demonstration purposes. Let's assume Lc = 100 meters and A = 30°. Now, we can calculate the radius R using the formula: R = (100 / 2) / sin(30° / 2) ≈ 100.93 meters. Using this radius, we can find the coordinates of points on the centerline of the curve at exact 25-meter multiples of the through chainage by incrementing the angle θ by the appropriate amount and calculating the corresponding x and y coordinates.

ii) To calculate the bearings and distances required to set out the circular curve from Control Point A (1959.00mE, 4002.00mN) and Control Point B (2040.00mE, 4015.00mN), we need to determine the bearing and distance from each control point to the center of the curve.

Using the coordinates of the control points and the coordinates of the intersection point, we can calculate the bearing using the formula: θ = atan2(y2 - y1, x2 - x1), where (x1, y1) and (x2, y2) are the coordinates of the control point and the intersection point, respectively. The distance can be calculated using the formula: d = sqrt((x2 - x1)^2 + (y2 - y1)^2).

By applying these formulas to the given control points and intersection point, we can determine the bearings and distances required to set out the circular curve from Control Point A and Control Point B.

Learn more about curve here : brainly.com/question/30958460

#SPJ11

Both planes A and B will take 45 minutes per client such that on Friday it takes 6 hours while on Saturday it takes 12 hours.

Determine the known quantities and designate the unknown quantity as a variable while trying to set up or construct a linear equation to fit a real-world application.

Let's say plane A takes x number of hours per client while plane B takes y number of hours per client.

On Friday, 3 clients did plane A while 5 did plane B.

So, total time = 3x + 5y

3x + 5y = 6

On Saturday,9 clients did plane A while 7 did plane B.

So,total time = 9x + 7y

9x + 7y = 12

Solving both equations,

(9x + 7y) - (9x + 15y) = 12 - (18)

-8y = -6

y = 3/4

And, 9x = 12 - 7(3/4)

x = 3/4

So, both planes take the same time which is 3/4 number of hours.

3/4 × 60 = 45 minutes.

Hence "Both planes A and B will take 45 minutes per client such that on Friday it takes 6 hours while on Saturday it takes 12 hours".

For more about the equation,

https://brainly.com/question/10413253

#SPJ1

Answer:

He needs to get $178 to average at least $150.

Step-by-step explanation:

105, 132, 165, 170, and 178 has an average of 150.

Answer:

Step-by-step explanation:

Percent means per one hundred, so to leave a 15% tip

28(15/100)=$4.20

The tip will be $4.20

Answer:

y= (3x-1)/2

Step-by-step explanation:

Answer:

33 braids

Step-by-step explanation:

What you want to do is first put in the equation. You put in 0.75x + 4=29.

Then you subtract 4 from 29 and get 25. Then divide 25 by 0.75 and you get the answer 33.3333333333. Round it to the nearest whole number and you get the final answer of 33 braids.

Answer:

(0, -2)

Step-by-step explanation:

y= 2x - 2

To find the y-intercept, or where the graph hits the y-axis, you plug 0 in for x

y= 2(0) - 2

y= -2

because 2 times 0 is 0, then 0 - 2 is -2

If they ask for just the value, it is -2

If they ask for the coordinate, it is (0, -2)

Answer:

the answer is -2

Step-by-step explanation:

We move all terms to the left:

Multiply

We add all the numbers and all the variables.

We move all terms containing x to the left hand side, all other terms to the right hand side