Sienna plays a game of chess and a game of pool.
Work out the probability that she does not win either of the two games.
Give your answer as a fraction in its simplest form.
Chess
10
32
10
FE
Win
Lose
Draw
1/3
|w/ w/~/
2
1
3
/2/3 1/3)
23
Pool
Win
Lose
Win
Lose
Win
Lose
Answers
The probability that Sienna does not win either of the two games is 1/15.
The probability that Sienna does not win either game is: 1- (winning chess - winning pool)
The probability that Sienna does not win either game = 1 - (6/10 + 1/3)
The probability that Sienna does not win either game = 1 - (18 + 10/30)
The probability that Sienna does not win either game = 1 - (28/30)
The probability that Sienna does not win either game = 30-28/30
The probability that Sienna does not win either game = 2/30
The probability that Sienna does not win either game= 1/15
Hence, there is a 1/15 chance that Sienna will lose either game.
To learn more about probability, refer:-
https://brainly.com/question/30034780
#SPJ1

Related Questions
How do you set up factor by grouping for the
polynomial:

Answers
GIVING BRAINLIEST!!
Lin drove to the campground, but forgot to pack her
tent and had to drive back home. This graph shows her
time and distance traveled.
How many hours did it take her to get to the
campground?
I’m stuck on this! Thank you so so much in advance!

Answers
Answer:
From home it looks like it took her 2 hours and 30 minutes
Step-by-step explanation:
If you look when she started, 10 A.M, and look when she first arrived at the campground, 12:30 P.M, you can see it took her 2 and a half hours.
Express E in its simplest form- b) Express 36 : '22 in its simplest form. 4 3 2 c) Express the fractions — . I and E as equivalent fractions with a denominator of It} 20. x 3 2 d) Workout;+;—§ e) Find the mean of the following dataset: 301, 285, 21]. 351. 35. 2135. 311. 25. 45. 310. 301. 305 Round your answer to two decimal places.
Answers
a) , 36 : '22 in its simplest form is 18 : 11., d) the mean is 301.25.
a) To express 36 : '22 in its simplest form, we need to find the greatest common divisor (GCD) of 36 and 22. The GCD of 36 and 22 is 2. Divide both numbers by the GCD to simplify the fraction. 36 ÷ 2 = 18 and 22 ÷ 2 = 11. So, 36 : '22 in its simplest form is 18 : 11.
b) To express the fractions x 3 2 and I in equivalent fractions with a denominator of 20, we need to multiply the numerator and denominator by the same number. For x 3 2, multiply both the numerator and denominator by 10. This gives us x 3 2 = 10 x 3 2 ÷ 2 10 ÷ 2 = 15 1. For I, multiply both the numerator and denominator by 20. This gives us I = 1 x 20 2 x 20 = 20 40.
c) To evaluate Workout;+;—§, substitute the values into the expression: 2 + 5 - 4 ÷ 2. Start by performing the division: 4 ÷ 2 = 2. Then, perform the addition: 2 + 5 = 7. Finally, perform the subtraction: 7 - 2 = 5.
d) To find the mean of the dataset: 301, 285, 21, 351, 35, 2135, 311, 25, 45, 310, 301, 305, add up all the numbers and divide by the total number of values. Adding all the numbers together gives us 3615. Since there are 12 numbers in the dataset, the mean is 3615 ÷ 12 = 301.25. Rounded to two decimal places, the mean is 301.25.
know more about simplest form
https://brainly.com/question/23302257
#SPJ11
What percentage of the data in a normal distribution is more than 1 standard deviation above the mean?
Answers
34% of the data in a normal distribution is more than 1 standard deviation above the mean.
In a normal distribution, about 68% of the data falls within one standard deviation above or below the mean. This means that roughly 34% of the data falls one standard deviation above the mean.
To be more precise, we can use the empirical rule or the 68-95-99.7 rule, which states that:
Approximately 68% of the data falls within one standard deviation of the mean.
Approximately 95% of the data falls within two standard deviations of the mean.
Approximately 99.7% of the data falls within three standard deviations of the mean.
Therefore, if we assume that the normal distribution is perfectly symmetrical, we can estimate that roughly 34% of the data falls more than one standard deviation above the mean.
To learn more about normal distribution here:
https://brainly.com/question/20875827
#SPJ4
Find the distance from the point to the given plane. (1, −3, 4), 3x + 2y + 6z = 5
Answers
The distance from the point to the given plane is 16/7 units.
To find the distance from a point to a given plane, we can use the formula:
distance = |ax + by + cz - d| / √(a^2 + b^2 + c^2)
where (x, y, z) are the coordinates of the point, and a, b, c, and d are the coefficients of the plane equation.
In this case, the point is (1, -3, 4) and the plane equation is 3x + 2y + 6z = 5.
Let's plug in the values:
distance = |(3*1) + (2*(-3)) + (6*4) - 5| / √(3^2 + 2^2 + 6^2)
Simplifying:
distance = |3 - 6 + 24 - 5| / √(9 + 4 + 36)
distance = |16| / √49
distance = 16 / 7
Therefore, the distance from the point (1, -3, 4) to the plane 3x + 2y + 6z = 5 is 16/7 units.
To know more about coefficients, visit:
brainly.com/question/1594145
#SPJ11
Some pls answer !! With work if possible

Answers
The sum of the series when evaluated are ∑aₙ² + 1 = 45, ∑bₙ² - 1 = 35, ∑(2aₙ + bₙ) = 0, ∑(aₙ + 3bₙ) = 0, ∑(aₙ/bₙ)² = 2, ∑(aₙ/bₙ)³ = -2, ∑aₙ² + ∑bₙ² = 80 and ∑aₙ² - ∑bₙ² = 0
Evaluating the sum of the seriesSeries 61
This series represents the summation of aₙ² + 1 from n = 1 to 5
From the graph, we have
a₁ = -4; a₂ = -2; a₃ = 0; a₄ = 2 and a₅ = 4
So, we have
∑aₙ² + 1 = (-4)² + 1 + (-2)² + 1 + (0)² + 1 + (2)² + 1 + (4)² + 1
∑aₙ² + 1 = 45
Series 62
This series represents the summation of bₙ² - 1 from n = 1 to 5
From the graph, we have
b₁ = 4; b₂ = 2; b₃ = 0; b₄ = -2 and b₅ = -4
So, we have
∑bₙ² - 1 = (4)² - 1 + (2)² - 1 + (0)² - 1 + (-2)² - 1 + (-4)² - 1
∑bₙ² - 1 = 35
Using the above formats, the sum of the remaining series is
Series 63
∑(2aₙ + bₙ) = (2 * 4 - 4) + (2 * 2 - 2) + (2 * 0 - 0) + (2 * -2 + 2) + (2 * -4 + 4)
∑(2aₙ + bₙ) = 0
Series 64
∑(aₙ + 3bₙ) = (4 - 3 * 4) + (2 - 3 * 2) + (0 - 3 * 0) + (-2 + 3 * 2) + (-4 + 3 * 4)
∑(aₙ + 3bₙ) = 0
Series 65
∑(aₙ/bₙ)² = (2/-2)² + (4/-4)²
∑(aₙ/bₙ)² = 2
Series 66
∑(aₙ/bₙ)³ = (2/-2)³ + (4/-4)³
∑(aₙ/bₙ)³ = -2
Series 67
∑aₙ² + ∑bₙ² = (-4)² + (4)² + (-2)² + (2)² + (0)² + (0)² + (2)² + (-2)² + (4)² + (-4)²
∑aₙ² + ∑bₙ² = 80
Series 68
∑aₙ² - ∑bₙ² = (-4)² - (4)² + (-2)² - (2)² + (0)² - (0)² + (2)² - (-2)² + (4)² - (-4)²
∑aₙ² - ∑bₙ² = 0
Read more about sigma notation at
https://brainly.com/question/29133967
#SPJ1
i am having trouble with a question on my geometry homework. on how to do it

Answers
a) Given the triangle ABC, you have to do a counterclockwise 90º rotation of the figure.
To make said rotation you have to invert the coordinates of each point of the figure and invert the sign of the x-coordinate of the image point.
In general:
Preimage point; Image point
P(x,y) → P'(-y,x)
The y-coordinate turns into the x-coordinate and the x-coordinate turns into the y-coordinate.
The x-coordinate of the image point must have the opposite sing as the original one.
So for triangle ABC:
A to A'
(3,-2) → (-(-2),3)= (2,3)
B to B'
(3,-6) → (-(-6),3)= (6,3)
C to C'
(9,-2) → (-(-2),9)= (2,9)
The coordinates for the 90º counterclockwise rotation are A'(2,3), B'(6,3) and C(2,9)
b) Triange A'B'C' was translated a certain number of units, its new position is given as triangle A''B''C'':
A''(-3,-4)
B''(1,-4)
C''(-3,2)
To determine what kind of translation was done, first step is to draw triangle A''B''C'' and compare it to triangle A'B'C':
As you can see in the graphic, triangle A'B'C' was translated horizontally to the left a k number of units and vertically downwards a m number of units.
Horizontal translation
These translations are made over the x-axis, the translation factor k is added (movement to the rigth) or subtracted (movement to the left) from the x-coordinate of each point:
In this case the translation was made to the left, so:
Preimage point; Image point
P(x,y) → P'(x-k,y)
Vertical translation
These translations are made over the y-axis, this means that the translation factor m will be added (↑up) or subtracted (↓down) from the y-coordinates of each point.
For the example, the movement was downwards so we can express it as:
Preimage point; Image point
P(x,y) → P'(x,y-m)
You can unite both movements in the same expression as:
Preimage point; Image point
P(x,y) → P'(x-k,y-m)
Going a little further you can determine the amount of units the figure was translated by comparing a set of points from the preimage and image:
Given A'(2,3) and A''(-3,-4)
For the horizontal movement compare the x-coordinates. We know that to determine the x-coordinate of A'', k units were subtacted from the x-coordinate of A', so:
2-k=-3
-k=-3-2
-k=-5
k=5
For the vertical movement, compare the y-coordinates of both point. We know that m units were subtracted from the y-coordinate of A' to determine the y-coordinate of A'', so:
3-m=-4
-m=-4-3
-m=-7
m=7
This means that the translation rule for A'B'C' → A''B''C'' is (x-5,y-7)


jim purchased notebooks. they were dollars each. write an equation to represent the total cost that jim paid.
Answers
Jim purchased notebooks. they were dollars each. Therefore, the equation to represent the total cost that Jim paid is:y = x dollars.
The amount or equivalent paid or charged for something : price. The average cost of a college education has gone up dramatically.
the outlay or expenditure (as of effort or sacrifice) made to achieve an object. He achieved fame, but at the cost of losing several friends.
Given that Jim purchased notebooks which were x dollars each, we can write an equation to represent the total cost that Jim paid as follows:
Let the total cost Jim paid be y.
Then, since he purchased x notebooks, the equation that represents the total cost that Jim paid is:
y = x dollars
for such more question on cost
https://brainly.com/question/2292799
#SPJ11
if two lines are parallel and one has a slope of -1/7, what is the slope of the other line?
Answers
-1/7, since parallel lines have equal slopes.
A frame is made from wire. The frame is a trapezium Calculate the total amount of wire needed to make the frame.

Answers
By find the missing side and sum the 4 sides
Chris is an hourly employee at a market. Which of the following statements is most likely to describe his job?
A.
He receives the same amount on his paycheck each week.
B.
He is expected to work overtime without compensation.
C.
He is paid a set amount each year and receives full benefits.
D.
He may be paid less each week if no work is available.
Answers
He may be paid less each week if no work is available, option D is correct.
Chris being an hourly employee suggests that he is paid based on the number of hours he works.
He may be paid less each week if no work is available reflects a common practice for hourly employees where their pay may vary depending on the availability of work.
If there is no work available, Chris may receive less pay for that week.
Hence, He may be paid less each week if no work is available, option D is correct.
To learn more on Statistics click:
https://brainly.com/question/30218856
#SPJ1
The lines represented by the equations 20y−24x=−20 and 5y−6x=−5 are...
a)perpendicular
b)the same line
c)neither parallel nor perpendicular
d)parallel
Answers
Answer:
parallel i think
Step-by-step explanation:
parallel because it's a unknown error
Answer:
Choice B
Step-by-step explanation:
The best way to compare two lines is to solve for y.
The first equation:
For \(20y-24x=-20\) the first thing we should do is add the 24x on both sides which will cancel out itself on the left side \(20y=24x-20\). Now all we have to do is divide the 20 on both sides to leave us with \(y=\frac{6}{5}-1\).
The second equation:
For \(5y-6x=-5\) the first thing we should do is add the 6x on both side which will cancel itself on the left side \(5y=6x-5\). Now all we have to do is divide the 5 on both sides to leave us with \(y=\frac{6}{5}x-1\).
Now for the comparison:
They are the same exact line. If you were to graph them they would overlap each other.
help me out i will give brainlist

Answers
Answer:
no
Step-by-step explanation:
0.06 = \(\frac{6}{100}\) ← that is 6 books out of 100
she requires to have 60 out of 100 , that is
\(\frac{60}{100}\) = 0.6
Two Way Table 1
SUV Sedan Totals
Male
21
39
60
45
180
Female
Total 156
84
The two-way table represents the results of a random survey taken to determine the preferred vehicle for male and female drivers.
Given that the participant is a female, which choice is the conditional relative frequency that she prefers an SUV?
es )
A)
0.25
B)
0.55
C)
0.75
D)
0.87

Answers
Answer: 0.75
Step-by-step explanation: took the same question
Answer:
0.75
Step-by-step explanation:
i just need no. 12 done

Answers
Answer:
y= -25
Step-by-step explanation:
Hope this helps!!
Question Write an absolute value equation that has the solutions x=2 and x=9
Answers
Absolute value equation that has the solutions x=2 and x=9 is |x - 5.5| = 3.5
5.5 is the mid-point between 2 and 9: (2+9)/2=5.5and the equation says that the points x= 2 and x= 9 are equally remoted at the distance of 3.5 from their mid-point.3.5 is the half of the distance 9-2 = 7 between the given points (the given values) x= 2 and x= 9.absolute value equation is |x - 5.5| = 3.5
Generalizations of the absolute cost for actual numbers arise in a huge form of mathematical settings. as an example, an absolute value is likewise defined for the complicated numbers, the quaternions, ordered jewelry, fields and vector spaces. absolutely the price is closely associated with the notions of value, distance, and norm in numerous mathematical and bodily contexts.
To learn more about Absolute value equation visit , https://brainly.com/question/2166748
#SPJ9
In a school, there are 1000 boys and a number of girls. The 48% of the total number of students that were successful in an examination was made up of 50% of the boys and 40% of the girls. What is the number of girls in the school?
Answers
Step-by-step explanation:
Let's call the number of girls in the school "g". We know that there are 1000 boys, so the total number of students is 1000 + g.
The problem states that 48% of the total number of students were successful in the examination. Therefore, we can write an equation:
0.48(1000 + g) = 0.5(1000) + 0.4(g)
Simplifying and solving for g:
480 + 0.48g = 500 + 0.4g
0.08g = 20
g = 250
Therefore, the number of girls in the school is 250.
Answer:
250
Step-by-step explanation:
Hi dear,
Firstly, let the girls be G
1000 + G = Total number of students
50% of boy = 1000 × 0.5 = 500
40% of girls = G × 0.4 = 0.4G
0.48 • (1000 + G) = 480 + 0.48G
480 + 0.48G = 500 + 0.4G
Collect Like Terms
0.48G - 0.4G = 500 - 480
0.08G = 20
G = 20/0.08
G = 250
Therefore, the girls are 250( two hundred and fifty)in the school
9,15,21,27,33
arithmetic, geometric or neither
Answers
Answer:
Arithmetic
Step-by-step explanation:
It is arithmetic because it keeps adding 6.
\(9+6=15\)
\(15+6=21\)
\(21+6=27\)
\(27+6=33\)
therefore, being Arithmetic.
Hope this helped!
brainliest please!
Answer:
Arithmetic
Step-by-step explanation:
#1) KEEP IN MIND: Arithmetic-add Geometric-multiply
#2) KEEP IN MIND: For checking do the opposite: arithmetic-subtract geometric-divide
Geometric check:
33/27=1.23
27/21=1.28
This is not geometric because it would have the same answer.
Arithmetic check:
33-27=6
27-21=6
This is arithmetic because it has the same answer.
Find an expression which represents the sum of (3x+9y)(3x+9y) and (5x+7y)(5x+7y) in simplest terms.
Answers
The expressions (7x - 6y) and (3x - 5y) added together result in 10x - 11y.
What is unitary method?Area is the entire amount of space occupied by a flat (2-D) surface or an object's shape.
On a sheet of paper, draw a square using a pencil. It has two dimensions. The area of a shape on paper is the area that it occupies.
Consider your square as being composed of smaller unit squares.
The number of unit squares necessary to completely cover the surface area of a specific 2-D shape is used to calculate the area of a figure. Some typical units for measuring area are square cms, square feet, square inches, square meters, etc.
Draw unit squares with 1-centimeter sides in order to calculate the area of the square figures shown below. The shape will therefore be measured.
According to our question-
(7x – 6y) and (3x – 5y
Then the sum of the expressions will be
(7x – 6y) + (3x – 5y)
7x – 6y + 3x – 5y
10x – 11y
Hence, The expressions (7x - 6y) and (3x - 5y) added together result in 10x - 11y.
learn more about unitary method click here:
brainly.com/question/24587372
#SPJ4
(a) Develop a first-order method for approximating f" (1) which uses the data f (x - 2h), f (x) and f (x + 3h). (b) Use the three-point centred difference formula for the second derivative to ap- proximate f" (1), where f (x) = 1-5, for h = 0.1, 0.01 and 0.001. Furthermore determine the approximation error. Use an accuracy of 6 decimal digits for the final answers of the derivative values only.
Answers
(a) Using a first-order method, we can approximate f"(1) as:
f"(1) ≈ [f(x-2h) - 2f(x) + f(x+3h)] / (5\(h^2\))
(b) The exact value of f"(1) is -1, so the approximation error for each of the above calculations is:
Error = |1.6 - (-1)| ≈ 2.6
(a) Using a first-order method, we can approximate f"(1) as:
f"(1) ≈ [f(x-2h) - 2f(x) + f(x+3h)] / (5\(h^2\))
(b) Using the three-point centered difference formula for the second derivative, we have:
f"(x) ≈ [f(x-h) - 2f(x) + f(x+h)] / \(h^2\)
For f(x) = 1-5 and x = 1, we have:
f(0.9) = 1-4.97 = -3.97
f(1) = 1-5 = -4
f(1.1) = 1-5.03 = -4.03
For h = 0.1, we have:
f"(1) ≈ [-3.97 - 2(-4) + (-4.03)] / (\(0.1^2\)) ≈ 1.6
For h = 0.01, we have:
f"(1) ≈ [-3.997 - 2(-4) + (-4.003)] / (\(0.01^2\)) ≈ 1.6
For h = 0.001, we have:
f"(1) ≈ [-3.9997 - 2(-4) + (-4.0003)] / (0.00\(1^2\)) ≈ 1.6
The exact value of f"(1) is -1, so the approximation error for each of the above calculations is:
Error = |1.6 - (-1)| ≈ 2.6
Therefore, the first-order method and three-point centered difference formula provide an approximation to f"(1), but the approximation error is relatively large.
For more such answers on the first-order method
https://brainly.com/question/31402376
#SPJ11
we are asked to develop a first-order method for approximating the second derivative of a function f(1), using data points f(x-2h), f(x), and f(x+3h). A first-order method uses only one term in the approximation formula, which in this case is the point-centred difference formula.
This formula uses three data points and approximates the derivative using the difference between the central point and its neighboring points. For part (b) of the question, we are asked to use the three-point centred difference formula to approximate the second derivative of a function f(x)=1-5, for different values of h. The approximation error is the difference between the true value of the derivative and its approximation, and it gives us an idea of how accurate our approximation is. (a) To develop a first-order method for approximating f''(1) using the data f(x-2h), f(x), and f(x+3h), we can use finite differences. The formula can be derived as follows: f''(1) ≈ (f(1-2h) - 2f(1) + f(1+3h))/(h^2) (b) For f(x) = 1-5x, the second derivative f''(x) is a constant -10. Using the three-point centered difference formula for the second derivative: f''(x) ≈ (f(x-h) - 2f(x) + f(x+h))/(h^2) For h = 0.1, 0.01, and 0.001, calculate f''(1) using the formula above, and then determine the approximation error by comparing with the exact value of -10. Note that the approximation error is expected to decrease as h decreases, and the final answers for derivative values should be reported to 6 decimal digits.
Learn more about first-order method here: brainly.com/question/16292276
#SPJ11
Find the difference in slopes. Can anyone help me with this? I need it, its a grade for my mah class.

Answers
Answer:
Slope of the graphed line, using 2 points (-1, 3) and (0, 0):
m₁ = 3/-1 = -3Slope of the line containing the points (-6, 2) and (-9, 5):
m₂ = (5 - 2)/(-9 - (-6)) = 3/-3 = -1The difference in number between the slopes:
-3 - (-1) = -2The difference in slopes:
The slope of the graphed line is 3 times steeper.Answer:
i like to know this answer
List and appraise a piece of music base on timbre
write a formula for a linear function f(x) that models the situation, where x is the number of years after 2007. in 2007 the average adult ate 52 pounds of chicken. this amount will increase by 0.8 pounds per year until 2012.
Answers
Step-by-step explanation:
x = number of years after 2007.
x = 0 for 2007.
PC(x) is a function that calculates how many pounds of chicken the average adult will eat every year (x) after 2007 (up to 2012).
PC(x) = 0.8x + 52
0 <= x <= 5
in 2007 (x = 0) the average adult ate 52 pounds.
in every year after 2007 until 2012 0.8 pounds get added to the amount of the previous year.
so, in 2012 (x = 5) the average adult will eat
PC(5) = 0.8×5 + 52 = 4 + 52 = 56 pounds of chicken.
A politician claims that a proposal for a new traffic law is broadly supported by both political parties and that a person from either political party is equally likely to support the proposed legislation. He cites two recent polls that said 70% of a random sample of 550 people from his political party supports the law, and 65% of a random sample of 420 people from the other political party supports the law. The 95 percent confidence interval for the difference in population proportions is (–0.010, 0.110). Based on the interval, is the politician’s claim justified?
The politician’s claim is not justified because the two sample proportions are different.
The politician’s claim is justified because the interval contains 0, which indicates no difference in the population proportions.
The politician’s claim may be justified because the interval contains 0, which indicates no difference in the population proportions. However, because the interval also contains positive and negative values, it is also plausible that there is a difference in the proportions of party members who support the new traffic law.
The politician’s claim is not justified because the interval contains both positive and negative numbers.
Answers
The politician's claim may be justified because the interval contains 0, indicating no difference in population proportions, but the presence of both positive and negative values within the interval suggests that there could still be a difference in support for the new traffic law between the two political parties.
Based on the given information, the politician's claim may be justified because the 95 percent confidence interval for the difference in population proportions (-0.010, 0.110) contains 0, indicating no difference in the population proportions. However, it is important to note that the interval also contains both positive and negative values, which suggests that there could potentially be a difference in the proportions of party members who support the new traffic law.
While the interval contains 0, which supports the politician's claim, the presence of positive and negative values suggests uncertainty. This means that it is possible for there to be a difference in support between the two political parties. Without further information or analysis, it is difficult to definitively conclude whether the claim is justified or not.
For more such questions on confidence interval
https://brainly.com/question/17097944
#SPJ8
In Little Kitty City, the tax rate is 7.25%. What would the tax be on a $500 TV? (Hint: Change 7.25% to a decimal by moving the decimal two spots to the left.
Answers
Answer:
Tax amount = $36.25
Step-by-step explanation:
The tax rate is 7.25%.
We need to find the tax be on a $500 TV.
It can be calculated as follows :
\(C=500\times 7.25\%\\\\=500\times \dfrac{7.25}{100}\\\\=\$36.25\)
So, the required tax on $500 TV is $36.25.
Consider the following data drawn independently from normally distributed populations: (You may find it useful to appropriate table: z table or t table)
xˉ1 = −17.1
s1^2 = 8.4
n1=22
xˉ2 = −16.0
s2^2 = 8.7
n2 = 24
a. Construct the 90% confidence interval for the difference between the population means. Assume the population va unknown but equal. (Round final answers to 2 decimal places.)
confidence interval is __ to __
Answers
The 90% confidence interval for the difference in the population means is -2.51 to 0.31
Calculating the 90% confidence interval for the population mean differenceFrom the question, we have the following parameters that can be used in our computation:
xˉ₁ = −17.1
s₁² = 8.4
n₁ = 22
xˉ₂ = −16.0
s₂² = 8.7
n₂ = 24
Calculate the pooled variance using
P = (df₁ * s₁² + df₂ * s₂²)/df
Where
df₁ = 22 - 1 = 21
df₂ = 24 - 1 = 23
df = 22 + 24 - 2 = 44
So, we have
P = (21 * 8.4 + 23 * 8.7)/44
P = 8.56
Also, we have the standard error to be
SE = √(P/n₁ + P/n₂)
So, we have
SE = √(8.56/22 + 8.56/24)
SE = 0.86
The z score at 90% CI is 1.645, and the CI is calculated as
CI = (x₁ - x₂) ± z * SE
So, we have
CI = (-17.1 + 16.0) ± 1.645 * 0.86
This gives
CI = -1.1 ± 1.41
Expand and evaluate
CI = (-2.51, 0.31)
Hence, the confidence interval is -2.51 to 0.31
Read more about confidence interval at
https://brainly.com/question/15712887
#SPJ1
Find the area of each figure
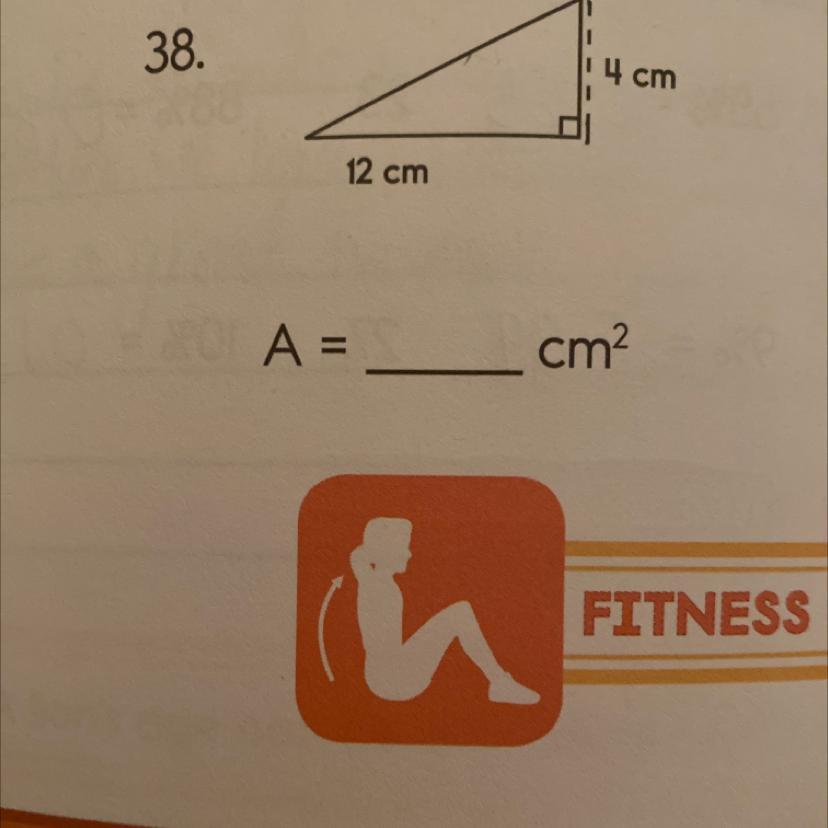
Answers
Answer:
24
Step-by-step explanation:
12*4/2
15. Davis wants to pour 5 gallons of punch into
gallon jugs How many jugs will he need?
81
(А)
2
NI
(В)
BE
2
© 10
D 15
Answers
The question is incomplete! Complete question along with answer and step by step explanation is provided below.
Question:
Davis wants to pour 5 gallons of punch into ½ gallon jugs How many jugs will he need?
A. 2½
B. 5½
C. 10
D. 15
Answer:
Number of jugs = 10
Davis will need 10 jugs to pour 5 gallons of punch into ½ gallon jugs.
Step-by-step explanation:
David has 5 gallons of punch that he wants to pour into jugs.
The capacity of 1 jug is ½ gallon.
The required number of jugs may be found as
Number of jugs = gallons of punch/capacity of jug
For the given case, we have
Gallons of punch = 5
capacity of jug (in gallons) = ½ = 0.5
So, the required number of jugs is,
Number of jugs = 5/½
Number of jugs = 5/0.5
Number of jugs = 10
Therefore, Davis will need 10 jugs to pour 5 gallons of punch into ½ gallon jugs.
could you help me with 11% and 9% thank you Assuming that the current interest rate is 10 percent, compute the present value of a five-year, 10 percent coupon bond with a face value of $1,000. What happens when the interest rate goes to 11 percent? What happens when the interest rate goes to 9 percent?
Answers
As the interest rate increases from 10 percent to 11 percent, the present value of the bond decreases from $1,074.47 to $1,058.31. Conversely, when the interest rate decreases to 9 percent, the present value increases to $1,091.19. This is because the discount rate used to calculate the present value is inversely related to the interest rate, meaning that as the interest rate increases, the present value decreases, and vice versa.
To compute the present value of a five-year, 10 percent coupon bond with a face value of $1,000, we need to discount the future cash flows (coupon payments and face value) by the appropriate interest rate.
Step 1: Calculate the present value of each coupon payment.
Since the bond has a 10 percent coupon rate, it pays $100 (10% of $1,000) annually. To calculate the present value of each coupon payment, we need to discount it by the interest rate.
Using the formula: PV = C / (1+r)^n
Where PV is the present value,
C is the cash flow,
r is the interest rate, and
n is the number of periods.
At an interest rate of 10 percent, the present value of each coupon payment is:
PV1 = $100 / (1+0.10)^1 = $90.91
Step 2: Calculate the present value of the face value.
The face value of the bond is $1,000, which will be received at the end of the fifth year. We need to discount it to its present value using the interest rate.
At an interest rate of 10 percent, the present value of the face value is:
PV2 = $1,000 / (1+0.10)^5 = $620.92
Step 3: Calculate the total present value.
To find the present value of the bond, we need to sum up the present values of each coupon payment and the present value of the face value.
Total present value at an interest rate of 10 percent:
PV = PV1 + PV1 + PV1 + PV1 + PV1 + PV2
PV = $90.91 + $90.91 + $90.91 + $90.91 + $90.91 + $620.92
PV = $1,074.47
When the interest rate goes to 11 percent, we would repeat the above steps using the new interest rate.
Total present value at an interest rate of 11 percent:
PV = PV1 + PV1 + PV1 + PV1 + PV1 + PV2
PV = $90.91 + $90.91 + $90.91 + $90.91 + $90.91 + $620.92
PV = $1,058.31
When the interest rate goes to 9 percent, we would repeat the above steps using the new interest rate.
Total present value at an interest rate of 9 percent:
PV = PV1 + PV1 + PV1 + PV1 + PV1 + PV2
PV = $90.91 + $90.91 + $90.91 + $90.91 + $90.91 + $620.92
PV = $1,091.19
Learn more about interest rate:
https://brainly.com/question/29451175
#SPJ11
Is 9,912 - - 798 positive or negative?
positive negative
PLS HELP ITS ON IXL I CANT GET IT WRONG!!
Answers
Answer:
positive
Step-by-step explanation:
assuming what you mean is subtracting a negative number, this will be positive.
subtracting a negative number will make the number positive, for example
8 - (-3) is the same as 8 + 3
In the country of Apexico, 6% of the population was unemployed in February of 2008. If there were 168,000,000 people willing and able to work in Apexico
during that month, how many people were unemployed?
A. 10,080,000
B. 28,000,000
C. 178,080,000
D. 157,920,000
Answers
The number of unemployed people in Apexico in February 2008 was 10,080,000. So, correct option is A.
To calculate the number of unemployed people in Apexico in February 2008, we can use the following formula:
Number of Unemployed = Total Population x Unemployment Rate
Substituting the given values:
Number of Unemployed = 168,000,000 x 0.06 = 10,080,000
Option A is the correct answer.
This calculation is based on the assumption that the unemployment rate is constant across the entire population of Apexico.
This calculation provides a rough estimate of the number of unemployed people in Apexico in February 2008, but it should be interpreted with caution.
To learn more about population click on,
https://brainly.com/question/9738551
#SPJ1