solve the following problem below

To find the combined rate of all the individuals working together, you must add the individual rates.

When calculating the combined rate of individuals working together, you need to add up the individual rates. Each worker contributes their own rate of work or productivity, and by adding these rates together, you can determine the combined rate at which they are working collectively.

This allows you to assess the overall efficiency and output of the team or group. By summing up the individual rates, we have better understanding of the overall productivity and performance of the group.

Read more about individual rates

brainly.com/question/31251379

#SPJ1

Answer:

1.Nonagon-convex, regular

2.Quadrilateral-convex, irregular

3.Hexagon-concave, irregular

Answer:

1.-Nonagon-convex, regular

2.-Quadrilateral-convex, irregular

3.-Hexagon-concave, irregular

Answer:

F. 122º

Step-by-step explanation:

By definition, the sum of 2 supplementary angles is 180º

Hence 58º + x = 180º

x = 180º - 58º - 122º

Answer: 2.5

Step-by-step explanation: r= sqrt(V/pi*h)

sqrt(171/(8.7)(3.14)

Step-by-step explanation:

solution given.:

radius=[r] let

height[h]=8.7in

volume[V] of cylinder=171in³

π=3.14

we have

volume[V] of cylinder=πr²h

171=πr×8.7

r²=\( \frac{171}{3.14×8.7}\)

r=\( \sqrt{6.26} \)

r=2.5in

so radius is 2.5in

The height of the tree to the nearest hundredth of a meter will be 6.40 meters.

Trigonometry is the branch of mathematics which set up a relationship between the sides and angles of right-angle triangles.

Let h be the height of the tree in meters. Then, based on the similar triangles formed by Ethan, his shadow, the tree, and its shadow, we have:

h / x = Ethan's height/distance to Ethan's feet from the tree

and

h / (x + 28.45) = Ethan's height/distance to Ethan's feet from the tip of the shadows

where x is the length of Ethan's shadow in meters. We can solve for x using the first equation as follows:

x = (Ethan's height/distance to Ethan's feet from the tree) * h

Substituting the given values, we get:

x = (1.85 / 24.3) x h = 0.076 x h

We can now substitute this value of x into the second equation and solve for h:

h / (0.076 x h + 28.45) = 1.85 / 24.3

Multiplying both sides by 0.076h + 28.45, we get:

h = (1.85 / 24.3) x (0.076h + 28.45)

Expanding the right side and simplifying, we get:

h = 0.070 x h + 5.95

Subtracting 0.070h from both sides, we get:

0.930h = 5.95

Dividing both sides by 0.930, we get:

h = 6.40 meters (rounded to the nearest hundredth)

Therefore, the height of the tree is approximately 6.40 meters.

To know more about trigonometry follow

https://brainly.com/question/26226884

#SPJ1

A function of the form y= A sin (Bx-C)+D that has period 8, phase shift -2, and the range -12 ≤y≤-4 is y = 4 sin (π/4 x + π/2) - 8

To write a function of the form y = A sin (Bx - C) + D that has a period of 8 and phase shift of -2, we can use the general formula:

y = A sin [(2π/P)(x - C)] + D

where P is the period, C is the phase shift, and D is the vertical shift. In this case, P = 8 and C = -2, so we have:

y = A sin [(2π/8)(x + 2)] + D

Simplifying the equation, we get:

y = A sin (π/4 x + π/2) + D

To find the amplitude A and vertical shift D that satisfy the range -12 ≤ y ≤ -4, we can use the fact that the sine function oscillates between -1 and 1. If we set A = 4, then the maximum value of y is 4 + D, and the minimum value of y is -4 + D. To ensure that the range is -12 ≤ y ≤ -4, we need to choose D such that:

4 + D ≤ -4 and -4 + D ≥ -12

Solving these inequalities, we get:

-8 ≤ D ≤ 0

Therefore, a function that satisfies the given conditions is:

y = 4 sin (π/4 x + π/2) - 8

To learn more about function click on,

https://brainly.com/question/30077307

#SPJ1

Answer:

2. 40

Step-by-step explanation:

Since we know that 9/15 has to be the same thing as 24/y

We know that 9/15 is .60 or 60%.

24/y has to be the same

If we slowly try every answer. 24/30, 24/40, 24/48, 24/58

We know that 24/40 equals .60 or 60% as well.

Therefore, your answer would be 2. 40

Answer:

1/6

Step-by-step explanation:

A (6,1)

B (12,2)

slope = (2-1)/(12-6)

1/6

28

Step-by-step explanation:

a^2=b^2+c^2-2bc\cos A

a

2

=b

2

+c

2

−2bccosA

From reference sheet.

s^2 = 34^2+16^2-2(34)(16)\cos 56

s

2

=34

2

+16

2

−2(34)(16)cos56

Plug in values.

s^2 = 1156+256-2(34)(16)(0.559193)

s

2

=1156+256−2(34)(16)(0.559193)

Square and find cosine.

s^2 = 1156+256-608.40188

s

2

=1156+256−608.40188

Multiply.

s^2 = 803.59812

s

2

=803.59812

Add.

s=\sqrt{803.59812} \approx28.348 \approx28

s=

803.59812

​

≈28.348≈28

Answer:

B

Explanation: dont got one

Answer:

The company charged $500 for its services,and Mia gives the movers a 16% tip. Now, we can add the tip amount to the cost of the service to find the total amount Mia paid: Total amount = Cost of service + Tip amount = $500 + $80 = $580

Step-by-step explanation:

Answer:

The first one

Step-by-step explanation:

She cant buy anything over $15, but she can buy something thats $15 :))

Answer:

1. 19/100

2. 3/10

3. 80%

4. 85%

5. 3/20

Using the double-declining-balance method of depreciation, the completion of the table as shown is as follows:

Year Cost   Accumulated   Book Value  Depreciation Accumulated  Book

                   Depreciation        B.O.Y         Expense      Depreciation   Value

                        B.O.Y                                                               E.O.Y       E.O.Y

1    $29,000          $0           $29,000         $11,600            $11,600   $17,400

2   $29,000    $11,600          $17,400          $6,960           $18,560  $10,440

3   $29,000   $18,560          $10,440           $4,176           $22,736   $6,264

What is the double-declining-balance method?

The double-declining-balance method is one of the depreciation methods in use, but it allocates more cost to the earlier years of the asset's life.

This depreciation method uses twice the straight-line rate, which is computed as the product of 100/estimated useful life multiplied by 2.

For each year's depreciation expense, the double rate is applied at the end of the year's balance.

For the last year, the difference between the previous year's ending balance and the residual value is taken as the depreciation expense.

Auto  = $29,000

Estimated useful life = 5 years

Residual = $2,000

Depreciable amount = $27,000 ($29,000 - $2,000)

Straight-line depreciation rate = 20% (100/5)

Double-declining depreciation rate = 40% (20% x 2)

Depreciation Expense:

1st year = $11,600 ($29,000 x 40%)

2nd year = $6,960 ($17,400 x 40%)

3rd year =  $4,176 ($10,440 x 40%)

4th year = $2,506 ($6,264  x 40%)

5th year = $1,758 ($3,758 - $2,000)

Year Cost   Accumulated   Book Value  Depreciation Accumulated  Book

                  Depreciation        B.O.Y         Expense     Depreciation   Value

                        B.O.Y                                                               E.O.Y       E.O.Y

1    $29,000          $0           $29,000         $11,600            $11,600   $17,400

2   $29,000    $11,600          $17,400          $6,960           $18,560  $10,440

3   $29,000   $18,560          $10,440           $4,176           $22,736   $6,264

4   $29,000  $22,736           $6,264          $2,506           $25,242   $3,758

5  $29,000  $25,242            $3,758           $1,758           $27,000  $2,000

Learn more about the double-declining-balance depreciation method here;

https://brainly.com/question/29335025

#SPJ1

Answer:

1. n= 20 /13

2. n=10

3. n=35

4. n=9

That's all I could do, I got tired of typing lol

Step-by-step explanation:

1.  4/n=13/5 we cross multiply, (4)*(5)=13*n, 20=13n Flip the equation.  13n=20, divide 13 on both sides, 13n/13=20/13, n= 20 /13

2. 9/6= 15/n, we cross multiply 9*n=(15)*(6), 9n=90, Divide both sides by 9. 9n/9=90/9 n=10

3. 28/4=n/5 Cross-multiply, (28)*(5)=n*(4), 140=4n Flip the equation.4n=140 Divide both sides by 4. 4n/4=140/4 n=35

4. n/6=6/4 we cross multiply,n*(4)=(6)*(6), 4n=36, Divide both sides by 4 4n/4=36/4.  n=9

Answer:

You got it right don't worry!

Step-by-step explanation:

It's most likely because the forms can be kinda tricky. If you don't put the exact answer your teacher put as correct, then it will mark it as wrong. I'd say just ask your teacher to look it over.

answer/explanation:

hope this helps

The Volume of the solid formed by the curves y = 4x³ , y = 4x, x ≥ 0 ; about the x axis is 64π/21  .

In the question ,

it is given that ,

the given curves are y = 4x³ , y = 4x, x ≥ 0 ,

we have to find the volume about x axis ,

the region formed by the given curves about x axis is shown below in the figure .

So , the Volume of the required region is

V = \(\int\limits^1_0 {\pi [(4x)^{2} - (4x^{3} )^{2} }] \, dx\)

On simplification ,

we get ,

V = \(\pi \int\limits^1_0 { [16x^{2} - 16x^{6} }] \, dx\)

V = π[ x³/3 - x⁷/7 ]¹₀

= 16π( 1/3 - 1/7 - 0 - 0)

= 16π((7 - 3)/21)

= 64π/21

Therefore , the required Volume is 64π/21   .

The given question is incomplete , the complete question is

Find the volume V of the solid obtained by rotating the region bounded by the given curves about the specified line. y = 4x³ , y = 4x, x ≥ 0 ; about the x-axis  .

Learn more about Volume here

https://brainly.com/question/12894092

#SPJ4

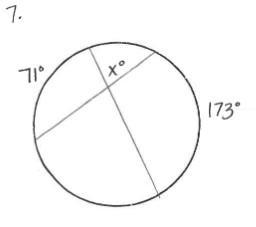
Answer:

Step-by-step explanation:

THE ANSWER IS : C

=

2

π

r

=

2

π

71

≈

446.10616

9514 1404 393

Answer:

  70

Step-by-step explanation:

If we consider the terms to have decreasing powers of x, then the middle term is the one with x^4y^4 as its variables. The full value of that term is ...

  (8C4)(2x)^4(3y)^4 = 70(6xy)^4

Substituting for x and y, this is ...

  70(6(1/3)(1/2))^4 = 70(1^4) = 70

The middle term is 70 when (x, y) = (1/3, 1/2).

_____

The value of nCk is n!/(k!(n-k)!). Then 8C4 is 8!/(4!)^2 = (8·7·6·5)/(4·3·2·1) = 70.

The polynomials in this problem that are in standard format are given as follows:

A. \(t^4 - 1\)

B. -2t² + 3t + 4.

C. 4t - 7.

The standard format of a polynomial is to write it in descending order of the exponents of the variable, with each term separated by a plus or minus sign.

For example, the standard format of a polynomial p(x) of the nth degree is given as follows:

\(p(x) = a_nx^n + a_{n-1}x^{n-1} + ... + a_2x^2 + a_1x + a_0\)

In which \(a_n, a_{n - 1}, \cdots, a_0\) are the coefficients.

For this problem, in all of the options a, b and c, the exponents are in decreasing order, hence the polynomials are in standard format.

More can be learned about polynomials at brainly.com/question/4142886

#SPJ1

MANOVA is a variation of ANOVA that allows us to identify and remove the effects of a third variable and increase statistical power.

Multivariate ANOVA (MANOVA) extends the capabilities of analysis of variance (ANOVA) by assessing multiple dependent variables simultaneously. ANOVA statistically tests the differences between three or more group means. For example, if we have three different teaching methods and you want to evaluate the average scores for these groups, we can use ANOVA. However, ANOVA does have a drawback. It can assess only one dependent variable at a time. This limitation can be an enormous problem in certain circumstances because it can prevent us from detecting effects that actually exist.

This statistical procedure tests multiple dependent variables at the same time. By doing so, MANOVA can offer several advantages over ANOVA.

To learn more about MANOVA.

https://brainly.com/question/29854848

#SPJ4

Answer:

Is 3(b+c) equivalent expressions?

A. True

Using Maxwell's equations, we can determine the magnetic flux density. One of the Maxwell's equations is:

\(\displaystyle \nabla \times \mathbf{H} = \mathbf{J} + \frac{\partial \mathbf{D}}{\partial t}\),

where \(\displaystyle \nabla \times \mathbf{H}\) is the curl of the magnetic field intensity \(\displaystyle \mathbf{H}\), \(\displaystyle \mathbf{J}\) is the current density, and \(\displaystyle \frac{\partial \mathbf{D}}{\partial t}\) is the time derivative of the electric displacement \(\displaystyle \mathbf{D}\).

In this problem, there is no current density (\(\displaystyle \mathbf{J} =0\)) and no time-varying electric displacement (\(\displaystyle \frac{\partial \mathbf{D}}{\partial t} =0\)). Therefore, the equation simplifies to:

\(\displaystyle \nabla \times \mathbf{H} =0\).

Taking the curl of the given magnetic field intensity \(\displaystyle \mathbf{R} =2\cos( 10^{10} t-600x)\hat{a}_{z}\, \text{Am}\):

\(\displaystyle \nabla \times \mathbf{R} =\nabla \times ( 2\cos( 10^{10} t-600x)\hat{a}_{z}) \, \text{Am}\).

Using the curl identity and applying the chain rule, we can expand the expression:

\(\displaystyle \nabla \times \mathbf{R} =\left( \frac{\partial ( 2\cos( 10^{10} t-600x)) \hat{a}_{z}}{\partial y} -\frac{\partial ( 2\cos( 10^{10} t-600x)) \hat{a}_{z}}{\partial z}\right) \mathrm{d} x\mathrm{d} y\mathrm{d} z\).

Since the magnetic field intensity \(\displaystyle \mathbf{R}\) is not dependent on \(\displaystyle y\) or \(\displaystyle z\), the partial derivatives with respect to \(\displaystyle y\) and \(\displaystyle z\) are zero. Therefore, the expression further simplifies to:

\(\displaystyle \nabla \times \mathbf{R} =-\frac{\partial ( 2\cos( 10^{10} t-600x)) \hat{a}_{z}}{\partial x} \mathrm{d} x\mathrm{d} y\mathrm{d} z\).

Differentiating the cosine function with respect to \(\displaystyle x\):

\(\displaystyle \nabla \times \mathbf{R} =-2( 10^{10}) \sin( 10^{10} t-600x)\hat{a}_{z} \mathrm{d} x\mathrm{d} y\mathrm{d} z\).

Setting this expression equal to zero according to \(\displaystyle \nabla \times \mathbf{H} =0\):

\(\displaystyle -2( 10^{10}) \sin( 10^{10} t-600x)\hat{a}_{z} \mathrm{d} x\mathrm{d} y\mathrm{d} z =0\).

Since the equation should hold for any arbitrary values of \(\displaystyle \mathrm{d} x\), \(\displaystyle \mathrm{d} y\), and \(\displaystyle \mathrm{d} z\), we can equate the coefficient of each term to zero:

\(\displaystyle -2( 10^{10}) \sin( 10^{10} t-600x) =0\).

Simplifying the equation:

\(\displaystyle \sin( 10^{10} t-600x) =0\).

The sine function is equal to zero at certain values of \(\displaystyle ( 10^{10} t-600x) \):

\(\displaystyle 10^{10} t-600x =n\pi\),

where \(\displaystyle n\) is an integer. Rearranging the equation:

\(\displaystyle x =\frac{ 10^{10} t-n\pi }{600}\).

The equation provides a relationship between \(\displaystyle x\) and \(\displaystyle t\), indicating that the magnetic field intensity is constant along lines of constant \(\displaystyle x\) and \(\displaystyle t\). Therefore, the magnetic field intensity is uniform in the given medium.

Since the magnetic flux density \(\displaystyle B\) is related to the magnetic field intensity \(\displaystyle H\) through the equation \(\displaystyle B =\mu H\), where \(\displaystyle \mu\) is the permeability of the medium, we can conclude that the magnetic flux density is also uniform in the medium.

Thus, the correct expression for the magnetic flux density in the given medium is:

\(\displaystyle B =6\cos( 10^{10} t-600x)\hat{a}_{z}\).

Answer:

2/3

Step-by-step explanation:

There are 4 possible outcomes that include a restaurant, a hardware store, or both. There are 6 possible store combinations (Possibilities = 1 grocery × 3 for the second store × 2 for the third store = 6). So the probability of having a group of stores with a restaurant or a hardware store is:

4/6 = 2/3

Answer:

Step-by-step explanation:

A=60

B=75

C=45

D=60

E=75

F=45

G=90

Answer:

its the same as 48

Step-by-step explanation:

Answer:

both sides are the same so it has to be also 48