what does conflixt mean
Answers
Answer: conflict means to clash with someone or something conflict is to disagree with someone
Related Questions
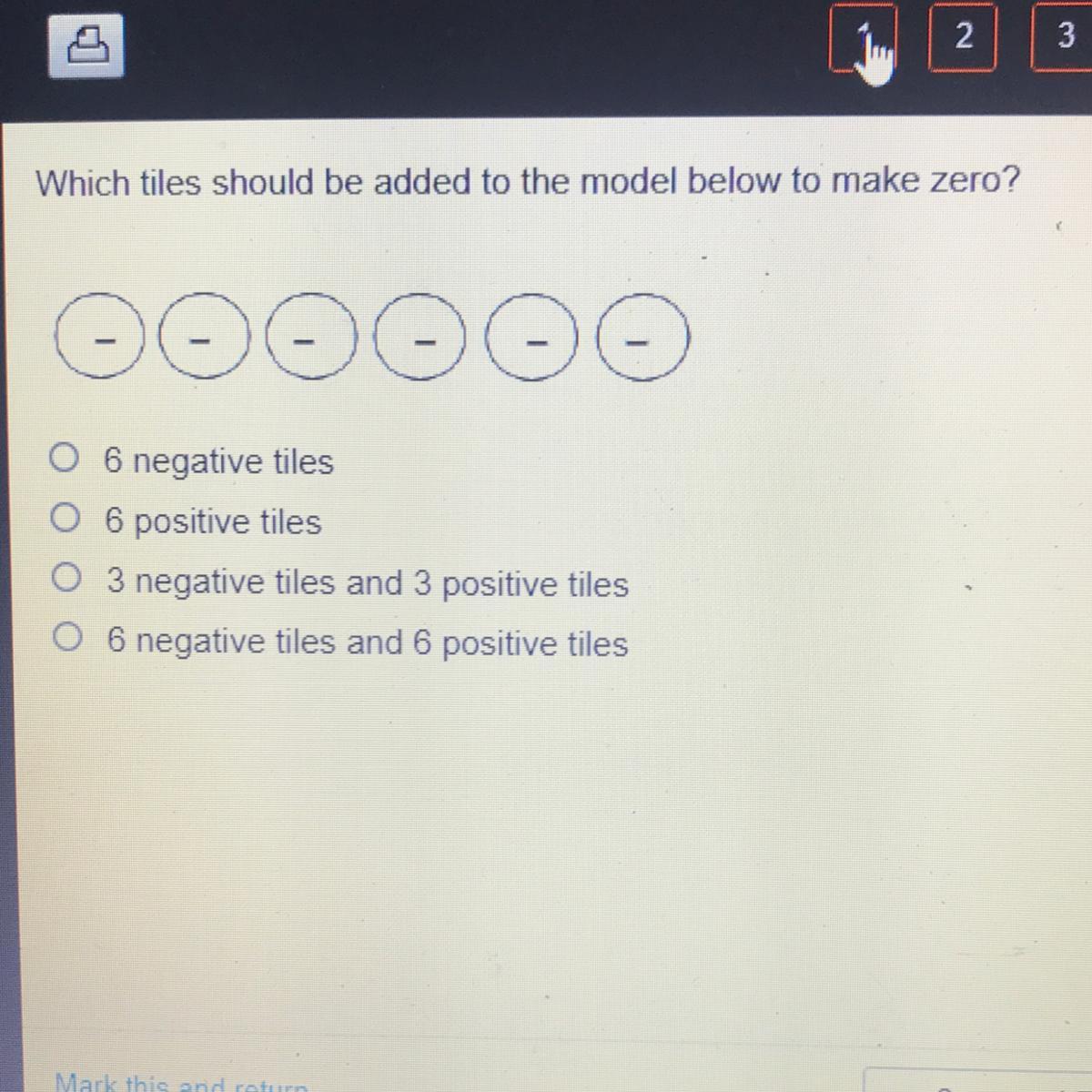
Which tiles should be added to the model below to make zero?
000000
6 negative tiles
6 positive tiles
3 negative tiles and 3 positive tiles
O 6 negative tiles and 6 positive tiles
Answers
Answer:
3 negative 3 positive
Step-by-step explanation:
-3+3=0
-10 + 2d=8 pls help me
Answers
Answer:
=-1
Step-by-step explanation:
-10+2d=8
-10 from 8
2d=-2
divode both sides by 2
d=-1
Answer:
1234minutos de los pobres de los pinzones
Solve x2 + 2 = 6 by graphing the related function.
A) 2
B) there are two solutions +v8
C) no real number solutions
D) +2
Answers
The solutions of the given equation are 2 or -2
What is an equation?An equation is a mathematical statement that shows that two mathematical expressions are equal.
Given is an equation, x²+2 = 6
x²+2 = 6
Solving we get,
x²+2 = 6
x² = 6-2
x² = 4
x = ±2
Hence, there are two solutions of the equation 2 or -2
Learn more about equations, click;
https://brainly.com/question/29657983
#SPJ1

help pleaseee
will name the brainliest

Answers
So basically choose B. You basically had to find the prime factors of each term in order to find the GCF
Answer:
2m^4
Step-by-step explanation:
What is 3/4 + 2/8 = ???
Answers
Answer: 1
Step-by-step explanation:
Answer:
1
Step-by-step explanation:
You can change the 3/4 to 6/8 and 2/8 + 6/8 = 8/8.
You could also change 2/8 to 1/4 and 3/4 + 1/4 = 4/4.
8/8 and 4/4 both equal 1.
Hope you get a good grade :)
let f and g be differentiable functions for which the following information is known: f(2) = 5, g(2) = 3, f′(2) = 1 2, g′(2) = 2. let h be the new function defined by the rule h(x) = 3f(x) −4g(x).
Answers
The value of h(x) at x = 2 is 3.
Given that f(x) and g(x) are differentiable functions with f(2) = 5, g(2) = 3, f′(2) = 1/2, and g′(2) = 2, and the new function h(x) is defined as h(x) = 3f(x) − 4g(x), we can find h'(2) by using the derivative rules for sums and products:
h'(x) = 3f'(x) - 4g'(x)
By plugging in the given values for f'(2) and g'(2), we get:
h'(2) = 3(1/2) - 4(2) = 3/2 - 8 = -6.5
Therefore, the derivative of h(x) at x = 2 is -6.5.
To find h(2), we can plug in the given values for f(2) and g(2) into the equation for h(x):
h(2) = 3f(2) - 4g(2) = 3(5) - 4(3) = 15 - 12 = 3
In conclusion, the derivative of h(x) at x = 2 is -6.5 and the value of h(x) at x = 2 is 3.
For more similar questions on derivative :
brainly.com/question/13475543
#SPJ11
Use the z-score formula, x-μ Z = -, and the information below to find the mean, 0 μ. Round your answer to one decimal place, if necessary. z = 2.25, x = 22.2, and = 1.6
Answers
The mean is 18.6.
Given the following information; z = 2.25, x = 22.2, and σ = 1.6, to find the mean, we have to apply the formula for z-score. z = (x - μ)/σWhere; z-score is represented by z, the value of X is represented by x, the mean is represented by μ and the standard deviation is represented by σSubstituting the values into the equation above;2.25 = (22.2 - μ)/1.6Multiplying both sides of the equation by 1.6, we have;1.6(2.25) = (22.2 - μ)3.6 = 22.2 - μ Subtracting 22.2 from both sides of the equation;3.6 - 22.2 = - μ-18.6 = - μ Multiplying both sides of the equation by -1, we have;μ = 18.6
Simply said, a z-score, also known as a standard score, informs you of how far a data point is from the mean. Technically speaking, however, it's a measurement of how many standard deviations a raw score is from or above the population mean.
You can plot a z-score on a normal distribution curve. Z-scores range from -3 standard deviations, which would fall to the extreme left of the normal distribution curve, to +3 standard deviations, which would fall to the far right. You must be aware of the mean and population standard deviation in order to use a z-score.
The z-score can show you how that person's weight compares to the mean weight of the general population.
Know more about mean here:
https://brainly.com/question/29141288
#SPJ11
In a recent study, the serum cholesterol levels in men were found to be normally distributed with a mean of 196.7 and a standard deviation of 39.1. Units are in mg/dL. What percentage of men have a cholesterol level that is greater than 240, a value considered to be high? Round your percentage 1 decimal place. (Take your StatCrunch answer and convert to a percentage. For example, 0.8765—87.7.) ______ %
Answers
The required percentage of men who have a cholesterol level greater than 240 is 9.4%.
Given, the serum cholesterol levels in men were found to be normally distributed with a mean of 196.7 and a standard deviation of 39.1. A value of 240 is considered to be high and we need to find the percentage of men who have a cholesterol level that is greater than 240.Statistical tools: We will use the Normal distribution tool from Statcrunch to find the required percentage of men. Normal Distribution tool from Statcrunch: For accessing the normal distribution tool, go to Stat > Calculators > Normal
In the normal distribution tool: Type the mean and the standard deviation of the population in the corresponding boxes.
Type 240 in the “Input X Value” box as we are looking for the probability of the men who have a cholesterol level greater than 240. Check the “above” checkbox as we are finding the probability of the cholesterol level greater than 240.
Click the “Compute” button to get the probability/proportion that represents the percentage of men who have a cholesterol level greater than 240. Hence, the answer is 9.4 %.
To learn more about percentage, visit:
https://brainly.com/question/28463297
#SPJ11
What is the exponent in the expression 4 5(exponet) ? 4 05 09 20
Answers
Answer:
i think it would be 05
if you mean 4^5 than the exponent if definitely 05
Hope this helped!
Step-by-step explanation:
There are 32 students going on a field trip. One fourth of them are girls. How many
boys are going on the trip?
Answers
Answer: 8
Step-by-step explanation:
1/4 = .25
32 x .25 = 8
there are 8 boys on the trip
plz mark brainliest
1/2=2/5y needhelp with this asap plz
Answers
Answer:
y=5/4
Step-by-step explanation:
Solve for y by simplifying both sides of the equation, then isolating the variable.
Plss help I’ll mark brainlist

Answers
Answer:
If it's 16, then it's -4 > x
Step-by-step explanation:
Ignore the inequality sign first and solve 12 = -3x
This would equal -4 = x
Now, when you divide or multiply both sides by a negative, you have to change the inequality sign so you change it from -4 > x to -4 < x
7 2/3 into a decimal
Answers
calculate p(84 ≤ x ≤ 86) when n = 9.
Answers
The probability of observing a sample mean between 84 and 86 when n = 9 is approximately 0.5878.
To calculate p(84 ≤ x ≤ 86) when n = 9, we first need to determine the distribution of the sample mean. Since the sample size is n = 9, we can use the central limit theorem to assume that the distribution of the sample mean is approximately normal with mean μ = 85 and standard deviation σ = σ/√n = σ/3, where σ is the population standard deviation.
Next, we need to standardize the values of 84 and 86 using the formula z = (x - μ) / (σ / √n). Plugging in the values, we get:
z(84) = (84 - 85) / (σ/3) = -1 / (σ/3)
z(86) = (86 - 85) / (σ/3) = 1 / (σ/3)
To calculate the probability between these two z-scores, we can use a standard normal table or a calculator with a normal distribution function. The probability can be expressed as:
P(-1/σ ≤ Z ≤ 1/σ) = Φ(1/σ) - Φ(-1/σ)
where Φ is the cumulative distribution function of the standard normal distribution.
Therefore, to calculate p(84 ≤ x ≤ 86) when n = 9, we need to determine the value of σ and use the formula above. If σ is known, we can plug in the value and calculate the probability. If σ is unknown, we need to estimate it using the sample standard deviation and replace it in the formula.
For example, if the sample standard deviation is s = 2, then σ = s * √n = 2 * √9 = 6. Plugging in this value in the formula, we get:
P(-1/6 ≤ Z ≤ 1/6) = Φ(1/6) - Φ(-1/6) = 0.2061 - 0.7939 = 0.5878
Therefore, the probability of observing a sample mean between 84 and 86 when n = 9 is approximately 0.5878.
To know more about sample mean refer here:
https://brainly.com/question/31101410
#SPJ11
Answer:
Step-by-step explanation:
The probability of observing a sample mean between 84 and 86 when n = 9 is approximately 0.5878.
To calculate p(84 ≤ x ≤ 86) when n = 9, we first need to determine the distribution of the sample mean. Since the sample size is n = 9, we can use the central limit theorem to assume that the distribution of the sample mean is approximately normal with mean μ = 85 and standard deviation σ = σ/√n = σ/3, where σ is the population standard deviation.
Next, we need to standardize the values of 84 and 86 using the formula z = (x - μ) / (σ / √n). Plugging in the values, we get:
z(84) = (84 - 85) / (σ/3) = -1 / (σ/3)
z(86) = (86 - 85) / (σ/3) = 1 / (σ/3)
To calculate the probability between these two z-scores, we can use a standard normal table or a calculator with a normal distribution function. The probability can be expressed as:
P(-1/σ ≤ Z ≤ 1/σ) = Φ(1/σ) - Φ(-1/σ)
where Φ is the cumulative distribution function of the standard normal distribution.
Therefore, to calculate p(84 ≤ x ≤ 86) when n = 9, we need to determine the value of σ and use the formula above. If σ is known, we can plug in the value and calculate the probability. If σ is unknown, we need to estimate it using the sample standard deviation and replace it in the formula.
For example, if the sample standard deviation is s = 2, then σ = s * √n = 2 * √9 = 6. Plugging in this value in the formula, we get:
P(-1/6 ≤ Z ≤ 1/6) = Φ(1/6) - Φ(-1/6) = 0.2061 - 0.7939 = 0.5878
Therefore, the probability of observing a sample mean between 84 and 86 when n = 9 is approximately 0.5878.
Dana has some coins. She wants to
display them in an array. Which of the
following numbers of coins provides only
2 arrays for Dana to choose from?
Answers
which of the
following numbers of coins provides only
arrays for Dana to choose from
Answer: D/29
you first read the question yourself then you find the array Dana could choose from
Juan took a test last week. He answered 17 questions correctly which is 85% correct. How many total questions were on the test?
Answers
Answer:20 questions I believe
Step-by-step explanation:
Answer:
20
Step-by-step explanation:
17 divided by 85%
Simplify by opening the parentheses: (2a−b)+(m−1)
Answers
Answer:
2a -b+m-1
Step-by-step explanation:
(2a−b)+(m−1)
Remove the parentheses
2a -b+m-1
There are no like terms to combine
2a -b+m-1
Savannah has a package of 20 candies with an equal number of candies colored white, blue, orange, purple, and yellow. If Savannah selected a yellow candy the first time, what is the
probability that she will randomly select, without replacement, another yellow candy?
A 3/19
B 1/5
C 4/19
D 1/4
Answers
Answer:
A 3/19
Step-by-step explanation:
There are 4 candies of each color. If you take out one yellow, you not only have three yellows; you have 19 candies. 3/19 is the answer.
Answer:
3/19
Step-by-step explanation:
There is an equal number of colored candies, in which there are 20 candies in all, and 5 colors:
20/5 = 4
Each color has 4 candies.
Savannah was able to obtain a yellow candy, and she does not replace it:
4/20 removing one yellow candy = 3/19
A) 3/19 is her chance of getting another yellow.
I need this for finals.
A: x = 7, y = 1.
B: x = 7, y = -1
C: x = 1, y = -7
D: x = -1, y = 6

Answers
Answer:
B. x = 7; y = -1
Step-by-step explanation:
xy = -7
x + y = 6
A and D don't work since the product of xy is not -7.
Try B: x = 7; y = -1
xy = -7
(7)(-1) = -7
-7 = -7
It works on the first equation.
x + y = 6
7 + (-1) = 6
6 = 6
It works on the second equation.
Answer: B. x = 7; y = -1
Perform the indicated operations and simplify.
(x - 3y)² + 3(x + y)(x − 4y) + x(3x + 4y + 3)
Answers
Let's simplify the expression step by step: Expand the squared term:
(x - 3y)² = (x - 3y)(x - 3y) = x² - 6xy + 9y²
Expand the second term:
3(x + y)(x − 4y) = 3(x² - 4xy + xy - 4y²) = 3(x² - 3xy - 4y²)
Expand the third term:
x(3x + 4y + 3) = 3x² + 4xy + 3x
Now, let's combine all the expanded terms:
(x - 3y)² + 3(x + y)(x − 4y) + x(3x + 4y + 3)
= x² - 6xy + 9y² + 3(x² - 3xy - 4y²) + 3x² + 4xy + 3x
Combining like terms:
= x² + 3x² + 3x² - 6xy - 3xy + 4xy + 9y² - 4y² + 3x
= 7x² - 5xy + 5y² + 3x
The simplified form of the expression is 7x² - 5xy + 5y² + 3x.
Learn more about expression here:
https://brainly.com/question/28170201
#SPJ11
Write the equation in standard form. Identity the center and radius.
x² + y2 + 8x-4y-7=0

Answers
Answer:
equation;
\((x + 4) {}^{2} + (y - 2) {}^{2} = 27\)
Center (-4,2)
Radius is
\(3 \sqrt{3} \)
Step-by-step explanation:
Since the x^2 and y^2 have the same coeffiecent this will be a circle in a form of
\((x - h) {}^{2} + (y - k) {}^{2} = {r}^{2} \)
Where (h,k) is center
r is the radius
So first we group like Terms together
\( {x}^{2} + 8x + {y}^{2} - 4y - 7 = 0\)
Add 7 to both sides
\( {x}^{2} + 8x + {y}^{2} - 4y = 7\)
\(( {x}^{2} + 8x) +( {y}^{2} - 4y) = 7\)
Since the orginal form of the equation of the circle has a perfect square we need to complete the square for each problem
\( (\frac{8}{2} ) {}^{2} = 16\)
and
\(( - \frac{4}{2} ) {}^{2} = 4\)
so we have
\( {x}^{2} + 8x + 16 + {y}^{2} - 4y + 4y = 7 + 16 + 4\)
\( {x}^{2} + 8x + 16 + {y}^{2} - 4y + 4y = 27\)
\((x + 4) {}^{2} + (y - 2) {}^{2} = 27\)
To find our center, h is -4 and k is 2
so the center is (-4,2)
The radius is
\( \sqrt{27} = 3 \sqrt{3} \)
So the radius is 3 times sqr root of 3.
In ΔNOP, the measure of ∠P=90°, NP = 35, ON = 37, and PO = 12. What ratio represents the sine of ∠N?
Answers
Answer:
12/37
Step-by-step explanation:
SOH - CAH - TOA
O = opposite
A = adjacent
H = hypotenuse
Sin = O/H
Sin N = 12/37
Answer:Find sin N
sinN=
hypotenuse/opposite
12/37
Step-by-step explanation:
The line segment XY with endpoints X(3,1) and Y (2,-2) is rotated 90° counterclockwise about (-6,4). What are the endpoints of XY?
Answers
The endpoints after the line segments have been rotated 90 degrees counterclockwise about (-6, 4 ) is x = (-1 3) y = (2 2).
What is the explanation for the above?When a line segment is rotated 90° counterclockwise about a point it is depicted as follows:
(x y) → (-y x); hence
x(3 1) → x( -1 3 )
y(2 -2) → y(2 2)
The rules indicate that for rotation in the direction of 90 degrees:
(x,y) becomes (y, -x) (x,y) rotation at 90 degrees counterclockwise produces (-y,x) 180-degree rotation → (x, y) Both clockwise and counterclockwise rotations produce (-x,-y)To rotate the figure 90 degrees counterclockwise around a point, each point (x, y) will rotate (y, -x).
Learn more about rotating line segments:
https://brainly.com/question/26641809
#SPJ1
Estimate the area of the following figure shown below. each square is 9ft
5.5 ft^2
6ft^2
49.5 ft^2
54 ft^2

Answers
Answer:
(c) 49.5 ft²
Step-by-step explanation:
We choose to estimate the area by comparison to the figure attached. Its area is (5.25)(9 ft²) = 47.25 ft². We don't believe the discrepancies are so large that we need to add another whole square to the area estimate.
Our choice is 49.5 ft².

Anna makes more money than Nancy use the graph at the right to make a table and write a rule for the relationship between their earnings

Answers
Answer: Nancy = X , Anna = Y X rule is plus 8 and Y rule is plus 12
Step-by-step explanation:

-5(-x – 3) - x + 2 = 1
Answers
Write a quadratic function with zeroes 0 and – 7. Write your answer using the variable x and in standard form with a leading coefficient of 1.
Answers
This is the standard form of a quadratic function with leading coefficient 1, and 0 and -7.
If the zeros of a quadratic function are 0 and -7, then the function can be written as:
f(x) = a(x - 0)(x - (-7))
Simplifying:
f(x) = a(x)(x + 7)
To find the value of 'a', we can use one of the points on the parabola. Since we know that the x-intercept is 0, we can substitute x = 0 and y = 0 into the equation:
0 = a(0)(0 + 7)
0 = 0
This tells us that a can be any value, as long as it's not 0. Let's choose a = 1 for simplicity. Then the quadratic function with zeros 0 and -7 is:
f(x) = (x)(x + 7)
f(x) = x^2 + 7x
To learn more about quadratic :
https://brainly.com/question/21145944
#SPJ1
The associative property works with expressions that use
Answers
The associative property works with expressions that use a property of addition and multiplication that states that the way the terms are grouped in an expression does not affect the final result.
In associative property, when you add or multiply several numbers together, you can regroup them in any way you like, and the sum or product will be the same.
For example, suppose we have the expression (2 + 3) + 4. By the associative property of addition, we can regroup the terms as 2 + (3 + 4) and the result will be the same, which is 9.
The associative property also works with more elaborate expressions, such as those involving variables, exponents, and parentheses. For instance, the expression 2a(bc) can be regrouped as (2ab)c or a(2bc), and the result will be the same. Similarly, the expression (3x)^2 y can be written as 9x^2 y, or as 3x (3xy), and the result will still be the same.
To know more about associative property:
https://brainly.com/question/30111262
#SPJ4
Evaluate -8 × |2|.
-16
-10
16
10
Answers
Answer:
\( - 8 \times |2 = - 16| \)
please solve this QUICKLY!!!!!!!!! (my life depends on it)

Answers
