Which expression can be used to represent the volume of this prism?
Response
14 × 3 units³
6 × 11 units³
48 × 3 units³
8 × 9 units³

Answers
Answer:
48 × 3 units³.
Step-by-step explanation:
V = l · w · h
8 · 3 · 6 = 144.
Answer is 144³.
Related Questions
An urn holds 9 identical balls except that 1 is white, 3 are black, and 5 are red. An exp How many outcomes are in the sample space for this experiment? How many outcomes are in the event "no ball is
Answers
Answer c
Step-by-step explanation:
How many solutions does the system of equations have?
7
2
4
O A. No solution
B. One solution: x = 0, y = 0
O c. One solution: x = 1, y = 5
O D. Infinitely many solutions
Answers
Answer:
We need to see the system of equations.
Step-by-step explanation:
Show us the system of equations.
what the slope of (15,9) (-10,3)
Answers
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️
\(slope = \frac{3 - 9}{ - 10 - 15} \\ \)
\(slope = \frac{ - 6}{ - 25} \\ \)
\(slope = \frac{6}{25} \\ \)
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️
Answer:
The slope is \(\frac{6}{25}\) or \(0.24\).
Step-by-step explanation:
This is the formula to calculate the slope from two given points: \(slope=\frac{y_2-y_1}{x_2-x_1}\) . Now, substitute (x1, y1) and (x2, y2) for (15,9) and (-10,3). \(slope=\frac{3-9}{-10-15}\) . That equation becomes \(slope=\frac{-6}{-25}\) . You have to change \(\frac{-6}{-25}\) to \(\frac{6}{25}\) . The slope is 6/25 or in decimal form, 0.24.
What is the area of the figure, 100 points pls hurry

Answers
Answer:
69.75 in^2
Step-by-step explanation:
Please see the attached image.
Let's first find the area of the bottom rectangle. The area of a rectangle is the length times the width.
9*4.5=40.5 in^2
Now let's find the area of the triangle on top. The area of a triangle is the base times the height divided by 2.
9*6.5/2=29.25 in^2
Now add the areas of the two shapes to get the total area of the figure.
40.5+29.25=69.75 in^2

Answer:
69.75 in²
--
First divide the shape into rectangles and triangles.
There is one triangle and one rectangle
Then . .
Solve the area of the bottom rectangle
-formula A = L x W
9 × 4.5 = 40.5 in²Then . .
Find the area of the triangle on top
-formula
A = 1/2 × b × h
Plug in . .
A = 1/2 × 9 × 6.5 = 29.25in²Finally add the two areas together to get the finale area!
40.5 + 29.25 = 69.75 in²
9:14=a:7
Find a pls help me
Answers
A box contains 7 plain pencils and 1 pen. A second box contains 3 color pencils and 3 crayons. One item from
each box is chosen at random. What is the probability that a pen from the first box and a crayon from the
second box are selected?
Write your answer as a fraction in simplest form.
Answers
The probability of selecting a pen from the first box is 1/8 (since there is only 1 pen out of 8 items in the box). The probability of selecting a crayon from the second box is 3/6 (since there are 3 crayons out of 6 items in the box).
To find the probability of both events happening together, we multiply the probabilities:
P(pen and crayon) = P(pen) x P(crayon)
P(pen and crayon) = (1/8) x (3/6)
Simplifying the fraction 3/6 to 1/2:
P(pen and crayon) = (1/8) x (1/2)
Multiplying the numerators and denominators:
P(pen and crayon) = 1/16
Therefore, the probability of selecting a pen from the first box and a crayon from the second box is 1/16.
Write True and false
A test statistic based on point estimation is used to construct the decision rule which defines the rejection region.
Answers
The given statement is False. A test statistic based on point estimation is not used to construct the decision rule which defines the rejection region.
In hypothesis testing, a test statistic is calculated using sample data and a specific hypothesis to assess the strength of evidence against the null hypothesis. The decision rule, which determines whether to reject or fail to reject the null hypothesis, is based on the test statistic's distribution under the null hypothesis, rather than the point estimate itself.
The construction of the decision rule involves selecting a significance level (alpha), which represents the probability of rejecting the null hypothesis when it is actually true. The rejection region is determined based on the chosen significance level and the distribution of the test statistic. If the calculated test statistic falls within the rejection region, the null hypothesis is rejected; otherwise, it is not rejected.
Point estimation, on the other hand, is used to estimate an unknown parameter of interest, such as the population mean or proportion, based on sample data. It involves calculating a single value (point estimate) that represents the best guess for the parameter value. The point estimate is not directly involved in constructing the decision rule or defining the rejection region in hypothesis testing.
Learn more about test statistic here:
https://brainly.com/question/31746962
#SPJ11
False. A test statistic based on point estimation is not used to construct the decision rule that defines the rejection region.
The process of hypothesis testing involves constructing a decision rule to determine whether to accept or reject a null hypothesis based on sample data. The decision rule is typically defined using a critical region or rejection region, which is a range of values for the test statistic.
Point estimation, on the other hand, is a method used to estimate an unknown population parameter based on sample data. It involves calculating a single value (point estimate) that serves as an estimate of the population parameter.
While point estimation and hypothesis testing are both important concepts in statistics, they serve different purposes. Point estimation is used to estimate population parameters, whereas hypothesis testing involves making decisions based on sample data.
The decision rule for hypothesis testing is typically constructed based on the significance level (alpha) and the distribution of the test statistic, such as the t-distribution or the standard normal distribution. The test statistic is calculated using sample data and compared to critical values or calculated p-values to determine whether to reject the null hypothesis.
Therefore, the statement that a test statistic based on point estimation is used to construct the decision rule defining the rejection region is false.
Learn more about statistic here:
https://brainly.com/question/32201536
#SPJ11
PLEASE HELP ME WITH MY PERSONAL NARRATIVE ESSAY

Answers
its a personal essay...
What is the value of the expression below.

Answers
Answer:
138
Step-by-step explanation:
Answer:
C. 138
Step-by-step explanation:
Substitute the values for their given variables:
6(5)² - 5(4) + 8 ⇒ 150 - 20 + 8 ⇒ 138
Find the Laplace transform where of the function f(t) =
{ t, 0 < t < {π + t π < t < 2π where f(t + 2 π) = f(t).
Answers
The Laplace Transform of f(t) isL{f(t)} = L{t} + L{t + π}u(t − π) − L{t − 2π}u(t − 2π) + ...
= (1/s^2) + e^{−πs}(1/s^2) − e^{-2πs}(1/s^2) + ...= (1/s^2)[1 + e^{−πs} − e^{−2πs} + ...]
Given function is,f(t) ={ t, 0 < t < π π < t < 2π}
where f(t + 2 π) = f(t)
Let's take Laplace Transform of f(t)
L{f(t)} = L{t} + L{t + π}u(t − π) − L{t − 2π}u(t − 2π) + ...f(t + 2π) = f(t)
∴ L{f(t + 2 π)} = L{f(t)}⇒ e^{2πs}L{f(t)} = L{f(t)}
⇒ [e^{2πs} − 1]L{f(t)} = 0L{f(t)} = 0
when e^{2πs} ≠ 1 ⇒ s ≠ 0
∴ The Laplace Transform of f(t) is
L{f(t)} = L{t} + L{t + π}u(t − π) − L{t − 2π}u(t − 2π) + ...
= (1/s^2) + e^{−πs}(1/s^2) − e^{-2πs}(1/s^2) + ...
= (1/s^2)[1 + e^{−πs} − e^{−2πs} + ...]
The Laplace Transform of f(t) isL{f(t)} = L{t} + L{t + π}u(t − π) − L{t − 2π}u(t − 2π) + ...
= (1/s^2) + e^{−πs}(1/s^2) − e^{-2πs}(1/s^2) + ...= (1/s^2)[1 + e^{−πs} − e^{−2πs} + ...]
Learn more about Laplace Transform
brainly.com/question/30759963
#SPJ11
Calculate the following integrals:
i. ∫ (x^-5 + 1/x) dx
ii. ∫5 ln(x+3)+7√x dx
iii. ∫3xe^x2 dx
iv. ∫xe7 dx
Answers
i. To calculate the integral of (x^-5 + 1/x) dx, we can split the integral into two separate integrals:
∫ x^-5 dx + ∫ (1/x) dx.
Integrating each term separately:
∫ x^-5 dx = (-1/4) * x^-4 + ln|x| + C, where C is the constant of integration.
∫ (1/x) dx = ln|x| + C.
Combining the results:
∫ (x^-5 + 1/x) dx = (-1/4) * x^-4 + ln|x| + ln|x| + C = (-1/4) * x^-4 + 2ln|x| + C.
ii. To calculate the integral of 5 ln(x+3) + 7√x dx, we can use the power rule and the logarithmic integration rule.
∫5 ln(x+3) dx = 5 * (x+3) ln(x+3) - 5 * ∫(x+3) dx = 5(x+3)ln(x+3) - (5/2)(x+3)^2 + C.
∫7√x dx = (7/2) * (x^(3/2)) + C.
Combining the results:
∫5 ln(x+3)+7√x dx = 5(x+3)ln(x+3) - (5/2)(x+3)^2 + (7/2)x^(3/2) + C.
iii. To calculate the integral of 3xe^x^2 dx, we can use the substitution method. Let u = x^2, then du = 2x dx.
Substituting u and du into the integral:
(3/2) * ∫e^u du = (3/2) * e^u + C = (3/2) * e^(x^2) + C.
iv. To calculate the integral of xe^7 dx, we can use the power rule and the exponential integration rule.
∫xe^7 dx = (1/7) * x * e^7 - (1/7) * ∫e^7 dx = (1/7) * x * e^7 - (1/7) * e^7 + C.
The results of the integrals are:
i. ∫ (x^-5 + 1/x) dx = (-1/4) * x^-4 + 2ln|x| + C.
ii. ∫5 ln(x+3)+7√x dx = 5(x+3)ln(x+3) - (5/2)(x+3)^2 + (7/2)x^(3/2) + C.
iii. ∫3xe^x^2 dx = (3/2) * e^(x^2) + C.
iv. ∫xe^7 dx = (1/7) * x * e^7 - (1/7) * e^7 + C.
To learn more about Integration - brainly.com/question/31744185
#SPJ11
Consider a general linear programming problem and suppose that we have a nondegenerate basic feasible solution to the primal. Show that the complementary slackness conditions lead to a system of equations for the dual vector that has a unique solution.
Answers
Linear programming problems are mathematical optimization problems where a linear objective function is subject to linear constraints. These problems can be solved using a variety of methods, including the simplex method and interior point methods.
A nondegenerate basic feasible solution is a solution to a linear programming problem where all the constraints are satisfied and the number of non-zero variables is equal to the number of constraints. This means that the solution is not at the corner of the feasible region and there is no redundant constraint.
Complementary slackness conditions are a set of conditions that must be satisfied by any optimal solution to a linear programming problem. These conditions state that the product of the slack variables (the difference between the left-hand side and right-hand side of a constraint) and the corresponding dual variable must be equal to zero.
Suppose we have a nondegenerate basic feasible solution to the primal. Then, the complementary slackness conditions will lead to a system of equations for the dual vector. Since the solution is nondegenerate, this system of equations will have a unique solution. This is because there are no redundant constraints, so the number of equations will be equal to the number of variables. Additionally, the complementary slackness conditions ensure that the system is not underdetermined or overdetermined.
Therefore, if we have a nondegenerate basic feasible solution to the primal, the complementary slackness conditions will lead to a system of equations for the dual vector that has a unique solution. This is an important result in linear programming, as it helps us to understand the relationship between primal and dual problems and the existence and uniqueness of solutions.
Learn more about Linear programming here:
https://brainly.com/question/31758568
#SPJ11
A medical researcher wanted to test and compare the impact of three different dietary supplements as a means to examine to what extent dietary supplements can speed up wound healing times. She randomly selected 36 patients and then randomly divided this group into three subgroups: a ‘Placebo’ group who ingested sugar-pills; a ‘Vitamin X’ group who took vitamin pills; and a ‘Kale’ group who took Kale pills. The study involved the groups taking their pill-based supplements three times a day for one week and at the end, their wound healing times were recorded
What sort of research design is this?
a. Repeated-measures factorial design.
b. Independent factorial design.
c. ANOVA.
d. Multiple linear regression.
Answers
The research design described is an independent factorial design, as it involves randomly assigning participants to different groups and manipulating the independent variable (type of dietary supplement) to examine its impact on the dependent variable (wound healing times).
The research design described in the scenario is an independent factorial design. In this design, the researcher randomly assigns participants to different groups and manipulates the independent variable (type of dietary supplement) to examine its impact on the dependent variable (wound healing times). The independent variable has three levels (Placebo, Vitamin X, and Kale), and each participant is assigned to only one of these levels. This design allows for comparing the effects of different dietary supplements on wound healing times by examining the differences among the three groups.
In this study, the researcher randomly divided the 36 patients into three subgroups, ensuring that each subgroup represents a different level of the independent variable. The participants in each group took their assigned pill-based supplement three times a day for one week, and at the end of the week, their wound healing times were recorded. By comparing the wound healing times among the three groups, the researcher can assess the impact of the different dietary supplements on the outcome variable.
Overall, the study design employs an independent factorial design, which allows for investigating the effects of multiple independent variables (the different dietary supplements) on a dependent variable (wound healing times) while controlling for random assignment and reducing potential confounding variables.
Learn more about divided here: https://brainly.com/question/15381501
#SPJ11
this years cornells took one more than three times as many field trips as last you class this year cornells class took a total 7 trips how many field trips did his class took last year
Answers
explanation: one more than three times of seven is two
you subtract one from seven to find the answer because it’s one more, to get six
six divided by 3 is 2 leaving you with your answer
If you have 40.495 / 1,000,000 - 4,000+ 6,000,900
Answers
...................
what
(6, -3) answer choices
(3, -6)
(0, 0)
(0, 5)

Answers
Answer:
(6, - 3 )
Step-by-step explanation:
The solution to a system of equations given graphically is at the point of intersection of the 2 lines.
The lines intersect at (6, - 3 ) ← solution
Multiply x - 5 and x + 3
Answers
Answer:
X²-2X-15
Step-by-step explanation:
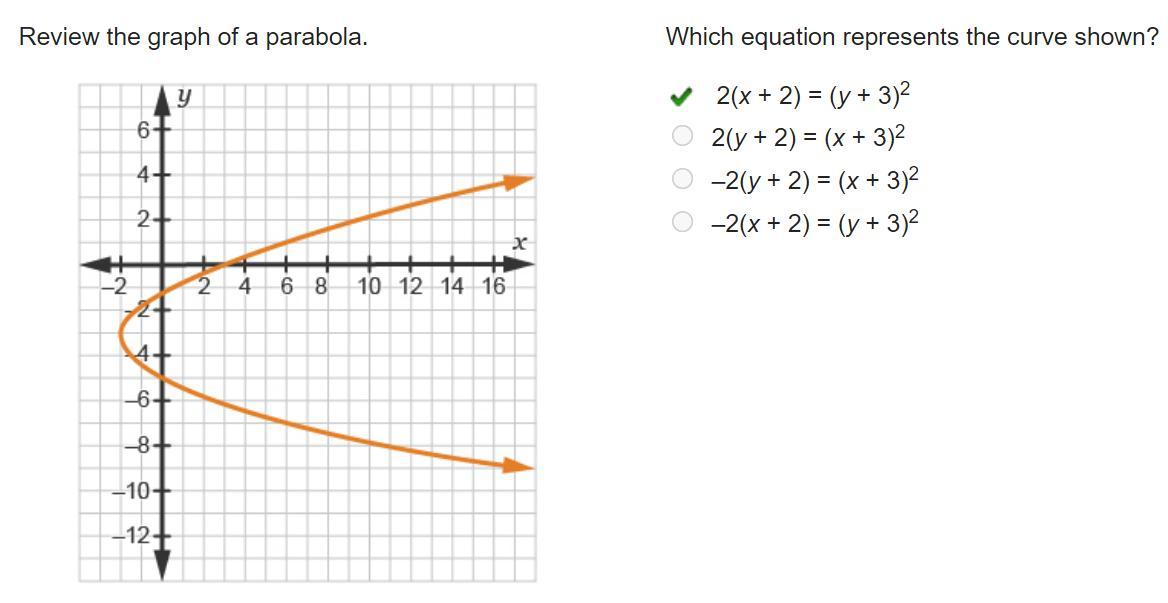
Which equation represents the curve shown? 2(x + 2) = (y + 3)2 2(y + 2) = (x + 3)2 –2(y + 2) = (x + 3)2 –2(x + 2) = (y + 3)2
Answers
Answer: 2(x+2)=(y+3)^2
Step-by-step explanation:
Answer:
A. 2(x+2) = (y+3)²
Step-by-step explanation:
Edge
what is the slope of the line that passes through the points (-4,-4) and (-4,-9)? Write your answer in simplest form
Answers
Answer:
undefined
Step-by-step explanation:
We can find the slope of a line using two points by
m = (y2-y1)/(x2-x1)
= (-9- -4)/(-4 - -4)
= (-9+4)/(-4+4)
= -5/0
When we divide by zero, our solutions is undefined
The slope is undefined
Picture below has question/answer choices!!


Answers
The statements that is true about the similarity of the two triangles the option D
D. ΔMNO and ΔJKL are not similar triangles
What are similar triangles?Similar triangles are triangles which have proportional corresponding sides
The parameters in the question are;
The length of segment MN = 20
Length of segment NO = 12
Length of segment OM = 25
Measure of angle ∠O = 56°
Length of segment LJ =- 15
Length of segment JK = 12
Length of segment KL = 9
Measure of angle ∠L = 56°
Two triangles are similar if the ratio of two sides on one triangle are proportional to two sides of another triangle, and the included angle between the two sides are congruent
The included angle between sides ON and MO on triangle MNO is congruent to the included angle between segment LK and JL in triangle JKL
However, the ratio of the sides LK to ON and JL to MO are;
9/12 ≠ 15/25
Therefore, the triangles are not similar
The correct option is option D
Learn more about similar triangles here:
https://brainly.com/question/2644832
#SPJ1
What is the average value of ƒ (x) = 3x² on [-4, 0]?
Answers
The average value of a function ƒ(x) on an interval [a, b], we need to calculate the definite integral of the function over that interval and divide it by the length of the interval (b - a). The average value of ƒ(x) = 3x² on the interval [-4, 0] is 8.
The average value of ƒ(x) = 3x² on the interval [-4, 0].
First, we calculate the definite integral of ƒ(x) over the interval [-4, 0]:
∫(from -4 to 0) 3x² dx
To evaluate this integral, we can use the power rule for integration. The power rule states that for any term of the form ax^n, the integral is (a/(n+1))x^(n+1). Applying this rule, we have:
∫(from -4 to 0) 3x² dx = [3/3 * x^3] (from -4 to 0)
Evaluating the integral at the upper and lower limits, we get:
[3/3 * 0^3] - [3/3 * (-4)^3]
Simplifying further:
0 - [3/3 * (-64)]
0 + 64 = 64
Now, we divide this result by the length of the interval [-4, 0], which is 4 - (-4) = 8:
Average value = 64 / 8 = 8
Therefore, the average value of ƒ(x) = 3x² on the interval [-4, 0] is 8.
Learn more about average value here:
https://brainly.com/question/33320783
#SPJ11
apply properties of operations to write an equivalent expression. +++
Answers
Answer:
uhhh
Step-by-step explanation:
good luck my mane man
A certain species of bird was introduced in a certain county 25 years ago. Biologists observe that the population doubles every 10 years, and now the population is 21,000. What was the initial size of the bird population?
Answers
The initial bird population density is 3713.
Given info;
A certain county received a new species of bird 25 years ago. According to biologists, the population doubles every ten years and is currently 21,000 people.
To get the initial size of the bird population;
We use the formula,
\(P(t)=Po* 2^\frac{t}{10}\)
Where Po is the initial population and t is the time in years.
\(21000= P(25)=Po*2^\frac{25}{10}\)
\(21000= Po*2^\frac{5}{2}\)
\(21000= 4\sqrt{2}*Po\)
\(Po = 21000/4\sqrt{2}\)
\(Po = 3712.31\\Po = 3713\)
Hence, the initial size of the bird population is 3713.
To learn more about population click here:
brainly.com/question/27779235
#SPJ4
the average temperature for a random sample of 56 covid patients was 101.2 with a known population standard deviation of 6. test at a 10% alpha level if the true average temperature of covid patients exceeds 100. what type of error could have occurred? and what are the chances of that happening?
Answers
We reject the null hypothesis and conclude that the true average temperature of COVID patients exceeds 100, with a type I error rate of 10% and a p-value of 0.0068 indicating a low probability of obtaining the observed sample mean if the null hypothesis were true.
To test if the true average temperature of COVID patients exceeds 100, we can use a one-sample z-test.
The null and alternative hypotheses are
Null hypothesis: The true average temperature of COVID patients is less than or equal to 100.
Alternative hypothesis: The true average temperature of COVID patients exceeds 100.
We can calculate the test statistic as
z = (x - μ) / (σ / sqrt(n))
where x is the sample mean, μ is the hypothesized population mean (100 in this case), σ is the population standard deviation, and n is the sample size.
Substituting the given values, we get
z = (101.2 - 100) / (6 / sqrt(56))
z = 2.47
We can find that the p-value is 0.0068. This means that if the true average temperature of COVID patients is actually 100, there is only a 0.68% chance of getting a sample mean of 101.2 or higher.
Since the alpha level is 10%, and the p-value is less than 10%, we reject the null hypothesis and conclude that the true average temperature of COVID patients exceeds 100.
The type of error that could have occurred is a type I error, which is rejecting the null hypothesis when it is actually true. The probability of a type I error is equal to the chosen alpha level, which is 10% in this case.
Learn more about one-sample z-test here
brainly.com/question/30763600
#SPJ4
Maisy hiked for 5 hours and burned 750 calories. She wants to find out how many calories she burned per hour, so she wrote the equation 5x=750 , where x represents the number of calories Maisy burned per hour. How many calories did Maisy burn in one hour?
Answers
Answer:
x = 150
Step-by-step explanation:
Given that,
The equation that is used to find out how many calories she burned per hour, the equation is as follows :
5x = 750
We need to find how many calories did Maisy burn in one hour.
For 1 hour use unitary method such that,
x = (750/5)
x = 150
So, she can 150 calories in one hour.
if the value of a in the quadratic function f(x) = ax^2 + bx + c is 1/2, the function will:

Answers
Answer:
Open up and have a minimum
Step-by-step explanation:
If the x² coefficient is:
Positive, the graph will open up and have a minimum
Negative, the gaph will open down and have a maximum
Answer:
opens up and has a minimum
Step-by-step explanation:
Provided a is not 0, y=ax^2+bx+c will be a parabola.
The parabola will open up and have a minimum if a is positive.
The parabola will open down and have a maximum if a is negative.
Since 1/2 is positive, then your parabola opens up and has a minimum.
in general, the strictest standards with the lowest acceptable levels are the
Answers
Answer:
Step-by-step explanation:
are the what???
PLEASE HELP I WILL GIVE BRAINLEIST!!!
7. The two equations have different solution steps. Do they have the same solution?
Use the distributive property to show why this answer makes sense.

Answers
Answer:
Yes, they have the same solution.
Step-by-step explanation:
Both of these equations have the same solution, just different steps.
To solve Ravi's equation, first we use the distributive property.
2(x+14) = 40
Multiply 2 by x and 14
2x + 28 = 40
Subtract 28 from both sides.
2x + 28 - 28 = 40 - 28
2x = 12
Divide by 2 on both sides.
2x/2 = x
12/2 = 6
so x = 6.
Fran's equation is the same equation as Ravi's second step.
Her equation of 2x + 28 = 40 is Ravi's after distributing 2.
Answer:
2(x+14)=40
2(x+14)=40
÷2 ÷2
x+14=20
-14 -14
x=6
2x+28=40
-28 -28
2x=12
÷2 ÷2
x=6
I hope this is what you wanted:
Make the biggest possible number using the digits below only once 3 , 1 , 3 answer
Answers
Answer:
331 the biggest possible number
Graph the function:
y = -3x + 2
Answers
Answer:
Step-by-step explanation:
