Which expression is equivalent to 2(4f+2g)
Answers
Answer:
8f+4g
Step-by-step explanation:
distribute the 2 to 4f and 2g. Thats all you can do to the expression tho
Related Questions
Given =(5,-2, 3) and >= (1, 1, 2) find an ordered triple that represents 3u - 2v.
Answers
The ordered triple representing \(3u - 2v\) is \((13, -8, 5)\).
To find an ordered triple representing \(3u - 2v\),
where \(u = (5, -2, 3)\) and \(v = (1, 1, 2)\),
we can perform the following operations:
\(3u = 3(5, -2, 3)\)
\(3u = (15, -6, 9)\)
\(2v = 2(1, 1, 2)\)
\(2v = (2, 2, 4)\)
Now, subtracting 2v from 3u gives:
\(3u - 2v = (15, -6, 9) - (2, 2, 4)\)
\(3u - 2v = (15-2, -6-2, 9-4)\)
\(3u - 2v = (13, -8, 5)\)
Therefore, the ordered triple representing 3u - 2v is (13, -8, 5).
For such more questions on ordered triple
https://brainly.com/question/29197252
#SPJ8
Danny "Dimes' Donahue is a neighborhood's 9 year old entrepreneur. His most recent venture is selling homemade brownies that he bakes himself. At a price of $1.50 each, he sells 100. At a price of $1 each he sells 300. Is demand elastic or inelastic over his price range? If demand had the same elasticity for a price decline from $1.00 to $0.50 as it does for the decline from $1.50 to $1, would cutting the price from $1.00 to $0.50 increase or decrease Dannys total revenue?
Answers
If demand had the same elasticity for a price decline from $1.00 to $0.50 as it does for the decline from $1.50 to $1, then cutting the price from $1.00 to $0.50 would result in a proportional increase in quantity demanded, similar to the increase from $1.50 to $1.
What formula we can use?
Danny "Dimes" Donahue is a young entrepreneur who sells homemade brownies. He sells 100 brownies at a price of $1.50 each, and 300 brownies at a price of $1 each. To determine the elasticity of demand over his price range, we can use the formula:
% change in quantity demanded / % change in price
When the price of the brownies decreases from $1.50 to $1, the quantity demanded increases from 100 to 300, which is a 200% increase. Therefore, the % change in quantity demanded is:
(300 - 100) / 100 = 2
When the price decreases from $1 to $0.50, the quantity demanded increases from 300 to 500, which is a 66.7% increase. Therefore, the % change in quantity demanded is:
(500 - 300) / 300 = 0.667
Since the % change in quantity demanded is greater when the price decreases from $1.50 to $1 than it is when the price decreases from $1 to $0.50, the demand is elastic over this price range.
If demand had the same elasticity for a price decline from $1.00 to $0.50 as it does for the decline from $1.50 to $1, then cutting the price from $1.00 to $0.50 would result in a proportional increase in quantity demanded, similar to the increase from $1.50 to $1.
In this case, Danny's total revenue would increase because the increase in quantity demanded would outweigh the decrease in price.
However, without more information about the specific elasticities, we cannot determine the exact impact on Danny's revenue.
To know more about Demand Curve visit:
https://brainly.com/question/30465432
#SPJ1
Find the value of x.
39°
22°
x°

Answers
Answer:
13
Step-by-step explanation:
Every 2 centimeters on a floor plan represents
meters of the house. The dining room is 8 cm by
10 cm on the floor plan, and the bedroom is 6cm by10cm on the floor plan. If installing tile costs $34
per square meter and installing carpet costs $21 per
square meter, how much would it cost to install tile
in the dining room and install carpet in the bedroom?
Show your work.
Answers
Given statement solution is :- It would cost $680 to install tile in the dining room and $315 to install carpet in the bedroom.
To find the cost of installing tile in the dining room and carpet in the bedroom, we need to calculate the areas of both rooms first.
Given:
Every 2 centimeters on the floor plan represents 1 meter of the house.
Dining Room:
On the floor plan, the dining room is 8 cm by 10 cm.
Converting this to meters, the dimensions of the dining room are 8 cm / 2 = 4 meters by 10 cm / 2 = 5 meters.
The area of the dining room is 4 meters * 5 meters = 20 square meters.
Bedroom:
On the floor plan, the bedroom is 6 cm by 10 cm.
Converting this to meters, the dimensions of the bedroom are 6 cm / 2 = 3 meters by 10 cm / 2 = 5 meters.
The area of the bedroom is 3 meters * 5 meters = 15 square meters.
Now, let's calculate the costs.
Cost of Tile:
The cost of installing tile is $34 per square meter.
The area of the dining room is 20 square meters.
Therefore, the cost of installing tile in the dining room is 20 square meters * $34/square meter = $680.
Cost of Carpet:
The cost of installing carpet is $21 per square meter.
The area of the bedroom is 15 square meters.
Therefore, the cost of installing carpet in the bedroom is 15 square meters * $21/square meter = $315.
Therefore, it would cost $680 to install tile in the dining room and $315 to install carpet in the bedroom.
For such more questions on Cost of tile and carpet.
https://brainly.com/question/29574063
#SPJ8
Which side measures will not make a triangle

Answers
With a triangle, the sum of any two side lengths must be greater than the third side length. If this is not true, then the side lengths cannot make a triangle. Let's go through each set of side lengths and determine which would and wouldn't work.
a. 3, 4, 8 - will not make a triangle
3 + 4 = 7 > 8 = false
3 + 8 = 11 > 4 = true
4 + 8 = 12 > 3 = true
b. 7, 6, 12 - will make a triangle
7 + 6 = 13 > 12 = true
7 + 12 = 19 > 6 = true
6 + 12 = 18 > 7 = true
c. 5, 11, 13 - will make a triangle
5 + 11 = 16 > 13 = true
5 + 13 = 18 > 11 = true
11 + 13 = 24 > 5 = true
d. 4, 6, 12 - will not make a triangle
4 + 6 = 10 > 12 = false
4 + 12 = 16 > 6 = true
6 + 12 = 18 > 4 = true
e. 4, 6, 10 - will not make a triangle
4 + 6 = 10 > 10 = false
4 + 10 = 14 > 6 = true
6 + 10 = 16 > 4 = true
Hope this helps!
Find each difference
(- 13n ) - ( 17 - 5n)
Answers
(-13n) + -1(17 + -5n) = 0
(-13n) + (17 * -1 + -5n * -1) = 0
(-13n) + (-17 + 5n) = 0
Reorder the terms:
-17 + (-13n) + 5n = 0
Combine like terms: (-13n) + 5n = -8n
-17 + -8n = 0
Solving
-17 + -8n = 0
Solving for variable 'n'.
Move all terms containing n to the left, all other terms to the right.
Add '17' to each side of the equation.
-17 + 17 + -8n = 0 + 17
Combine like terms: -17 + 17 = 0
0 + -8n = 0 + 17
-8n = 0 + 17
Combine like terms: 0 + 17 = 17
-8n = 17
Divide each side by '-8'.
n = -2.125
Simplifying
n = -2.125
Consider the polynomials p1(t) = 1 + t , p2(t) = 1 -t , and p3(t) = 2 (for all t). By inspection, write a linear dependence relation among p1, p2, and p3. Then find a basis for Span{ p1 , p2 , p3 }.
Answers
Answer:
\(\{p_1,p_2\}$ is a basis of Span\{p_1,p_2,p_3\}\)
Step-by-step explanation:
Given the polynomials:
\(p_1(t) = 1 + t\\ p_2(t) = 1 -t\\p_3(t) = 2\\\)
On Inspection
\((1+t)+(1-t)=1+1+t-t=2\\$Therefore:\\p_1(t)+p_2(t)=p_3(t)\)
By the Spanning Theorem
If one vector in S is a linear combination of the others, we can delete it and get a subset (one vector smaller) \(S' \subseteq S\) that has the same span.
Therefore, since \(p_3(t)=p_1(t)+p_2(t)\)
\(Span\{p_1,p_2,p_3\}=Span\{p_1,p_2\}\)
\(p_1$ and p_2\) are linearly independent because \(p_1\) cannot be written in terms of \(p_2\).
Therefore, \(\{p_1,p_2\}$ is a basis of Span\{p_1,p_2,p_3\}$ as required.\)
A linear dependence relation among the polynomials p₁, p₂, and p₃ is given as p₁ + p₂ = p₃
What is polynomial?Polynomial is an algebraic expression that consists of variables and coefficients. Variable are called unknown. We can apply arithmetic operations such as addition, subtraction, etc. But not divisible by variable.
Consider the polynomials are
\(\rm p_1(t) = 1 + t\\\\ p_2(t) = 1 -t \\\\ p_3(t) = 2\)
a linear dependence relation among p₁, p₂, and p₃ will be
Let add the p₁ and p₂, we have
1 + t + 1 -t = 2
We get the polynomial p₃.
Thus the linear relation is p₁ + p₂ = p₃
More about the polynomial link is given below.
https://brainly.com/question/17822016
How many parts does the net of a cylinder have?
four
three
two
five
Answers
Answer:
the net of a cylinder has 3 parts to it
Answer:
B three
Step-by-step explanation:
The net of a cylinder consists of three parts: One circle gives the base and another circle gives the top. A rectangle gives the curved surface.
Suppose a large shipment of televisions contained 9% defectives. If a sample of size 393 is selected, what is the probability that the sample proportion will differ from the population proportion by less than 3%
Answers
Answer:
0.9624 = 96.24% probability that the sample proportion will differ from the population proportion by less than 3%
Step-by-step explanation:
To solve this question, we need to understand the normal probability distribution and the central limit theorem.
Normal Probability Distribution
Problems of normal distributions can be solved using the z-score formula.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the z-score of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
Central Limit Theorem
The Central Limit Theorem establishes that, for a normally distributed random variable X, with mean \(\mu\) and standard deviation \(\sigma\), the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean \(\mu\) and standard deviation \(s = \frac{\sigma}{\sqrt{n}}\).
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean \(\mu = p\) and standard deviation \(s = \sqrt{\frac{p(1-p)}{n}}\)
Suppose a large shipment of televisions contained 9% defectives
This means that \(p = 0.09\)
Sample of size 393
This means that \(n = 393\)
Mean and standard deviation:
\(\mu = p = 0.09\)
\(s = \sqrt{\frac{p(1-p)}{n}} = \sqrt{\frac{0.09*0.91}{393}} = 0.0144\)
What is the probability that the sample proportion will differ from the population proportion by less than 3%?
Proportion between 0.09 - 0.03 = 0.06 and 0.09 + 0.03 = 0.12, which is the p-value of Z when X = 0.12 subtracted by the p-value of Z when X = 0.06.
X = 0.12
\(Z = \frac{X - \mu}{\sigma}\)
By the Central Limit Theorem
\(Z = \frac{X - \mu}{s}\)
\(Z = \frac{0.12 - 0.09}{0.0144}\)
\(Z = 2.08\)
\(Z = 2.08\) has a p-value of 0.9812
X = 0.06
\(Z = \frac{X - \mu}{s}\)
\(Z = \frac{0.06 - 0.09}{0.0144}\)
\(Z = -2.08\)
\(Z = -2.08\) has a p-value of 0.0188
0.9812 - 0.0188 = 0.9624
0.9624 = 96.24% probability that the sample proportion will differ from the population proportion by less than 3%
What is the gcf of 3/7 and 4/9?
Answers
Answer:
1/63
Step-by-step explanation:
There are a couple of ways to do this.
1)Look for the GCF of the numerators when a common denominator is used.
GCF(3/7, 4/9) = GCF(27/63, 28/63) = (1/63)·GCF(27, 28)
GCF(3/7, 4/9) = 1/63
__
2)Use Euclid's algorithm. If the remainder from division of the larger by the smaller is zero, then the smaller is the GCF; otherwise, the remainder replaces the larger, and the algorithm repeats.
(4/9)/(3/7) = 1 remainder 1/63*
(3/7)/(1/63) = 27 remainder 0
The GCF is 1/63.
__
* The quotient is 28/27 = 1 +1/27 = 1 +(1/27)(3/7)/(3/7) = 1 +(1/63)/(3/7) or 1 with a remainder of 1/63.
_____
Additional comment
3/7 = (1/63) × 27
4/9 = (1/63) × 28
Which expression gives the best estimate of 30 percent of 61?
A.) 1/5 (60)
B.) 1/10 (60)
C.) 1/4 (60)
D.) 1/2 (60)
Answers
Answer:
The answer is A
Step-by-step explanation:
I did the test and I made a 100.
Mark me as branilest
Answer:
The answer is A. Hope it helps!
small bottle of Gatorade holds 75% as much as the large bottle of Gatorade. If the small bottle holds 36 units, how many units does the large bottle hold?
Answers
Answer:
48 unit
Step-by-step explanation:
36=x75%
x=36/75% = 48
double check just in case :)
Use | = PRT to solve.
(time is in years)
| = $300
P = $3,000
T = 0.5 years
Find R.
[?]%
Answers
Answer:R=20%
Step-by-step explanation:
In response to the increasing weight of airline passengers, the Federal Aviation Administration in 2003 told airlines to assume that passengers average 190 pounds in the summer, including clothing and carry‑on baggage. But passengers vary, and the FAA did not specify a standard deviation. A reasonable standard deviation is 35 pounds. Weights are not normally distributed, especially when the population includes both men and women, but they are not very non‑Normal. A commuter plane carries 22 passengers. What is the approximate probability P that the total weight of the passengers exceeds 4500 pounds? Use the four‑step process to guide your work. Give your answer as a percentage precise to two decimal places. P=___?
Answers
The approximate probability P that the total weight of the passengers exceeds 4500 pounds is 10.03%.
Probability is a measure of the likelihood of an event to occur. Many events cannot be predicted with total certainty. We can predict only the chance of an event to occur i.e., how likely they are going to happen, using it. Probability can range from 0 to 1, where 0 means the event to be an impossible one and 1 indicates a certain event.
The probability formula is defined as the possibility of an event to happen is equal to the ratio of the number of favorable outcomes and the total number of outcomes.
Probability of event to happen P(E) = Number of favorable outcomes/Total Number of outcomes
Total of 22 is more than 4500 is equivalent to average of 22 is more than \($\frac{4500}{22}\)=204.545
\($$\begin{aligned}P(\bar{x} > 204.545) & =1-P(\bar{x} < 204.545) \\& =1-P\left(\frac{\bar{x}-\mu}{\sigma / \sqrt{u}} < \frac{204.545-195}{35 / \sqrt{22}}\right) \\& =1-P(z < 1.2792) \\\end{aligned}$$\)
= 1 - 0.8997
= 0.1003
= 10.03%
Therefore, the approximate probability P that the total weight of the passengers exceeds 4500 pounds is 10.03%.
For more such questions on Probability
https://brainly.com/question/12905909
#SPJ4
The HCF of three numbers is 8 and the sum of these numbers is 80. List the possible set of such three numbers.
Answers
Let's denote the three numbers A, B, and C.
Given that the highest common factor (HCF) of these three numbers is 8 and their sum is 80, we can consider possible combinations of numbers that satisfy these conditions.
Since the HCF is 8, all three numbers must be divisible by 8. Additionally, the sum of the numbers is 80, so we need to find combinations of three numbers that satisfy both conditions.
Let's list the possible combinations:
(8, 16, 56): In this case, A = 8, B = 16, and C = 56. All three numbers are divisible by 8, and their sum is 8 + 16 + 56 = 80.(16, 8, 56): Here, A = 16, B = 8, and C = 56. Again, all three numbers are divisible by 8, and their sum is 16 + 8 + 56 = 80.(24, 8, 48): In this combination, A = 24, B = 8, and C = 48. All three numbers are divisible by 8, and their sum is 24 + 8 + 48 = 80.(8, 24, 48): Similarly, A = 8, B = 24, and C = 48. All three numbers are divisible by 8, and their sum is 8 + 24 + 48 = 80.These are the four possible sets of three numbers that satisfy the given conditions: (8, 16, 56), (16, 8, 56), (24, 8, 48), and (8, 24, 48).
Yolanda scored 10 points in a basketball game. She could have scored with one‐point free throws, two‐point field goals, or three‐point field goals. In how many different ways could she have scored her 10 points?
Answers
she could have scored the 10 points in 302400 ways.
The given parameters are
n= total points =10
r1 =one-point free throw = 1
r2 = two-point field goals = 2
r3 = three-point field goals = 3
The number of ways (k) she could have scored the points is:
k=(n!)/(r1!×r2!×r3!)
The factorial function is a mathematical formula represented by an exclamation mark "!".
k= 10!/(1!×2!×3!)
k= 3628800/(1×2×6)
k= 302400
so.. she could have scored the 10 points in 302400 ways.
To learn more about Combinations
visit : brainly.com/question/15301090
#SPJ9
What are the solutions of the following system?
[x2 + y2 = 25
2x+y=-5
0 (0,-5) and (-5, 5)
O (0, -5) and (5, -15)
O (0,-5) and (-4, 3)
O (0, -5) and (4, -13)
Answers
Answer:
5h-fgtx56=y2-6
Step-by-step explanation:
sorry if wrong:(
Figure 1 and Figure 2 below are congruent. Which point corresponds to point C'?

Answers
The point in Figure 2 that corresponds to point L in Figure 1 is the midpoint of line segment NP.
When two figures are congruent, it means that they have the same shape and size. This implies that corresponding points in the two figures have the same position and distance from each other.
In Figure 1, the points I, J, L, K, N, P, and Q are labeled. To find the point in Figure 2 that corresponds to point L, we need to look for a point that has the same relative position and distance as point L in Figure 1.
From Figure 1, we can see that point L is the midpoint of the line segment JK. Therefore, to find the corresponding point in Figure 2, we need to look for a point that is the midpoint of the line segment that corresponds to JK. In Figure 2, the line segment that corresponds to JK is NP, and the midpoint of NP corresponds to the point that is equivalent to point L. Therefore, the point that corresponds to point L in Figure 2 is the midpoint of line segment NP.
It is important to note that identifying corresponding points in congruent figures is a fundamental concept in geometry and is often used to solve problems involving congruence and similarity.
for such more question on congruent figures
https://brainly.com/question/26085366
#SPJ11
Is F=9/5C proportional?
Answers
Answer:
No, it is not
Step-by-step explanation:
Answer:
No it is not
Step-by-step explanation:
let C be the curve y=5sqrtx for 1.1

Answers
We can integrate this S = 2π ∫(1.1 to 4.4) (5√(4x + 25))/(2√x) dx over the given interval (1.1 to 4.4) to find the surface area.
We can evaluate the integral using numerical methods or a calculator to find the final answer.
We have,
To find the surface area of the revolution about the x-axis of the function f(x) = 5√x over the interval (1.1 to 4.4), we can use the formula for the surface area of revolution:
S = ∫(a to b) 2πy√(1 + (f'(x))²) dx
In this case,
f(x) = 5√x, so f'(x) = (d/dx)(5√x) = 5/(2√x).
Let's calculate the surface area:
S = ∫(1.1 to 4.4) 2π(5√x)√(1 + (5/(2√x)²) dx
Simplifying the expression inside the integral:
S = ∫(1.1 to 4.4) x 2π(5√x)√(1 + 25/(4x)) dx
Next, we can integrate this expression over the given interval (1.1 to 4.4) to find the surface area.
To find the surface area of revolution about the x-axis of the function
f(x) = 5√x over the interval (1.1 to 4.4), we need to evaluate the integral:
S = ∫(1.1 to 4.4) 2π(5√x)√(1 + 25/(4x)) dx
Let's calculate the integral:
S = 2π ∫(1.1 to 4.4) (5√x)√(1 + 25/(4x)) dx
To simplify the calculation, let's simplify the expression inside the integral first:
S = 2π ∫(1.1 to 4.4) (5√x)√((4x + 25)/(4x)) dx
Next, we can distribute the square root and simplify further:
S = 2π ∫(1.1 to 4.4) (5√(4x + 25))/(2√x) dx
Thus,
We can integrate this expression over the given interval (1.1 to 4.4) to find the surface area.
We can evaluate the integral using numerical methods or a calculator to find the final answer.
Learn more about the surface area of revolutions here:
https://brainly.com/question/32268747
#SPJ1
PLease see attached. This is an algebra question

Answers
The solution for the given expression is 16.
Power RulesThere are different power rules, see some them:
1. Multiplication with the same base: you should repeat the base and add the exponents.
2. Division with the same base: you should repeat the base and subctract the exponents.
3.Power. For this rule, you should repeat the base and multiply the exponents.
4. Zero Exponent. When you have an exponent equals to zero, the result must be 1.
First, you apply the Power Rules - Power for \((\frac{2^2x^2y}{xy^3} )^2}\). For this rule, you should repeat the base and multiply the exponents. Thus, the result will be:\(\frac{16x^4y^2}{x^2y^6}\).
After that, you should apply the Power Rules - Division . For this rule, you should repeat the base and subctract the exponents. Thus, the result will be:\(\frac{16x^2}{y^4}\).
Now, you should replace the variable x by 4 and the variable y by 2. Thus, the result will be:\(\frac{16*4^2}{2^4}=\frac{16*16}{16} =16*1=16\)
Read more about power rules here:
brainly.com/question/12140519
#SPJ1
Hey can anyone help me understand how to graph this?

Answers
Answer:
Step-by-step explanation:
?
help quick!!! plsssss

Answers
Since an actual mosquito is about 1.6 cm, the scale factor of the diagram is equal to 2.
What is scale factor?In Geometry, the scale factor of a geometric figure can be calculated by dividing the dimension of the image (new figure) by the dimension of the pre-image (actual figure):
Scale factor = Dimension of image (new figure)/Dimension of pre-image (actual figure)
Conversion:
1 cm = 10 mm
1.6 cm = 1.6 × 10 = 16 mm.
Substituting the given parameters into the scale factor formula, we have the following;
Scale factor = 32/16
Scale factor = 2.
Read more on scale factor here: brainly.com/question/20482938
#SPJ1
which one is the coldest AMD why
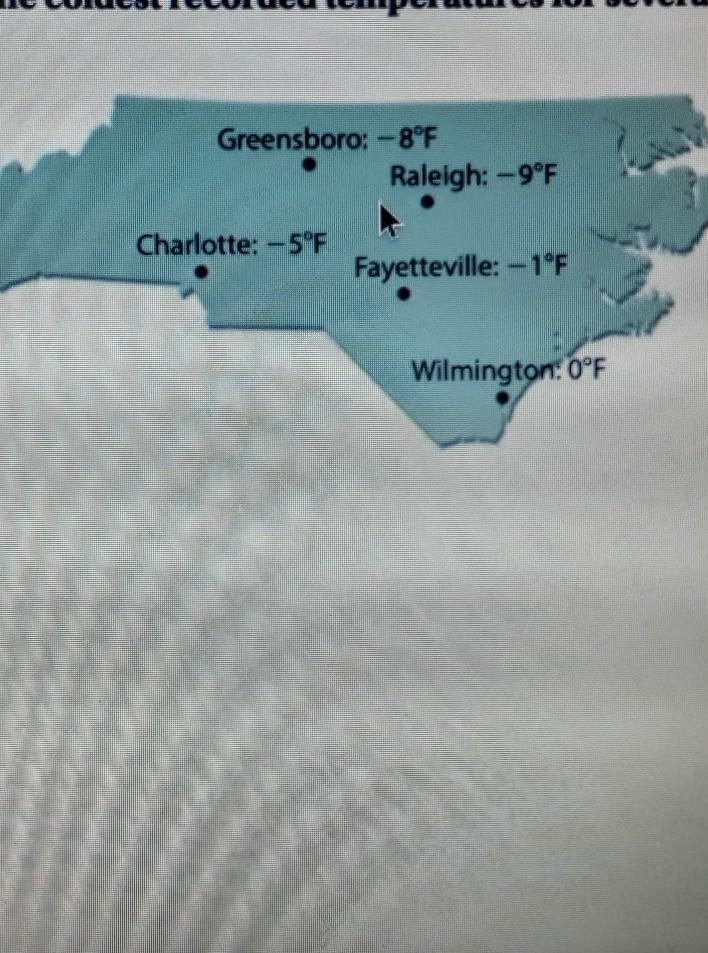
Answers
Answer:
Raleigh.
Step-by-step explanation:
Just look for the Fahrenheit with the smallest number.
Answer:Raleigh
Step-by-step explanation:Your going to go for the smallest and I just took the quiz
y.y3 write without exponts
Answers
Answer:
Y x Y x Y x Y
Step-by-step explanation:
The exponent tells how many times that number is multiplied.
So, x^3 is the same as multiplying x 3 times.
3,998-(-7)= can you please help me with this problem
Answers
Answer:
4005
Step-by-step explanation:
3,998 - (-7) = ?
Two negative signs will make a positive sign.
3,998 - (-7) = 3998 + 7 = 4005
So, the answer is 4005
Please explain your answer to the question in the picture with steps.

Answers

The graph of y = -x + 2 is negative
over the interval (4, 0) and positive over the
interval (-0, 4). What happens on the graph
when x = 4? Explain.
Answers
A cube has an edge length that is a rational number of centimeters. Identify which measurement will require the use of irrational numbers? (1 point)
O the diagonal of one of the cube's faces
O the volume of the cube
O the surface area of the cube
O the area of one of the cube's faces
Answers
Answer:
A) the diagonal of one of the cube's facesStep-by-step explanation:
Let the edge be r, a rational number.
Then the following are:
A) The diagonal of one of the cube's faces,
d = r\(\sqrt{2}\), this is always irrational.B) The volume of the cube,
V = r³ - always rational.C) The surface area of the cube,
S = 6r² - this is always rational.D) The area of one of the cube's faces,
A = r²- this is always rational.what percentage of the wall was painted