PLEASE HURRY
What is the scientific notation for a length of
0.000028 meter?
A. 2.8 x 10^5
B. 0.28 x 10^-4
C. 2.8 x 10^-5
D. 28 x 10^-6
Answers
Answer:
C. 2.8 * 10^-5
Step-by-step explanation:
Ok so its been a while since I've done these but pretty sure c is the answer. (Double check to make sure I'm correct)
Answer:
The answer is C and good luck on whatever you're doing!
Related Questions
A rectangular field measures 616m by 456m. Fencing posts are placed along it's sides at equal distances. What will be the distance between the posts if they are place as far apart as possible?
Answers
Answer: 766.41m
Step-by-step explanation:
Hi, since the situation forms 2 right triangles (see attachment) we have to apply the Pythagorean Theorem for one triangle.
c^2 = a^2 + b^2
Where c is the hypotenuse of the triangle (in this case the diagonal distance between corners of the field, which is the distance between the posts if they are placed as far apart as possible ) and a and b are the other sides.
Replacing with the values given:
c^2 = 616^2 + 456^2
c^2 = 379,456+207,936
c^2 =587,392
c = √587,392
c = 766.41m
So, she is within the area serviced by the tower (20 miles)
Feel free to ask for more if needed or if you did not understand something.

80 divided by 192.0!!!!!!!!!!!!!!!
Answers
Answer: .416666667
Step-by-step explanation: Take 80 and divide it by 192.0= .416666667
Select the correct answer.
The graphs represent functions f and g.
-10.
10
-10
9
Which ordered pair represents (fg)(2) on the graph of the combined function?
OA. (4,42)
OB. (2,9)
O C. (2,81)
OD. (4,81)
Answers
Jelene is cutting a strip of yard that is 11/12 inch long into the pieces that are 2/12 inch long for a collage. How many complete pieces can she make?
Answers
Answer:
5
Step-by-step explanation:
2/12 goes in 11/12 5 times. 1/12 inch will be left.
What is (-4/7) - (-10/7)=?
Answers
Answer: 6/7
Step-by-step explanation:
Answer:
0.85714285714 rounded to 0.86
Step-by-step explanation:
(-4/7) = -0.57<- rounded up
(-10/7)=-1.43<-rounded up
0.57- -1.43=0.86
Use technology to compute each probability and to carefully sketch a graph corresponding to each expression. a. X N(3.7, 4.55), P(3.0 sX3 4.0) b. X~N(62, 100), P50-45) f. X~N(7.6, 12), P(8 XS 9) P(X 2 45)
Answers
To compute the probabilities and sketch the graphs, we can use technology such as a statistical software or a graphing calculator. The steps involved are as follows:
(a) For X ~ N(3.7, 4.55), to find P(3.0 ≤ X ≤ 4.0), we can use the normal distribution function with the given mean and standard deviation. By inputting the values into the software, it will calculate the probability for us. Similarly, for (b) X ~ N(62, 100), to find P(45 ≤ X ≤ 50), and (c) X ~ N(7.6, 12), to find P(8 ≤ X ≤ 9) and P(X ≥ 45), we can follow the same process.
Once the probabilities are computed, we can plot the corresponding graphs to visualize the probability distributions. Each graph will have the X-axis representing the variable X and the Y-axis representing the probability density. The graphs will show the range of X values and the corresponding probabilities within that range.
By utilizing technology, we can efficiently calculate the probabilities and create accurate graphs to visualize the distributions.
To learn more about probabilities click here:
brainly.com/question/32117953
#SPJ11
A parcel box has a height of 3 centimeters and the volume is expressed as 6x2 + 24x + 18. Write
the area of the base. Then determine the dimensions (length and width) of the base.
(HINT: Volume / height = area of the base)
Area of the Base_______
Dimensions of the base_______
and______
Answers
The area of the base of the parcel box is given by the expression A=2x²+8x+6. The dimensions (length and width) are determined from this expression as (2x+2) and (x+3).
In general, the volume of a rectangular box is calculated by the product of the height, width, and length of the box. From the given hint, we can write the volume of the parcel box as a product of the height and the area of the base. This is written as,
\(\begin{aligned}V&=A\times h\\6x^2+24x+18&=A\times 3\\A&=\frac{1}{3}(6x^2+24x+18)\\A&=2x^2+8x+6\end{aligned}\)
The above equation gives the area of the base
Now solving the above quadratic equation to find dimensions, we get,
\(\begin{aligned}A&=2x^2+8x+6\\&=2x^2+6x+2x+6\\&=2x(x+3)+2(x+3)\\&=(2x+2)(x+3)\end{aligned}\)
The dimensions are (2x+2) and (x+3).
To know more about the volume of rectangle:
https://brainly.com/question/29079942
#SPJ4
-2x-4+2x+32-2x-21=18
Answers
Answer:
x = -5.5
Step-by-step explanation:
-2x - 4 + 2x + 32 - 2x - 21 = 18
-2x - 4 + 32 - 21 = 18
-2x + 7 = 18
-2x = 11
x = -5.5
So, the answer is x = -5.5
What would x equal (rounded to the nearest tenth)

Answers
Hypothenuse = 26 cm
With opposite and hypothenuse, you use sine
sin(41) = 0.656059029
Sin(41) = x/26
x = 17.05753
Round to tenth
Solution: x = 17.1 cm
draw the graph of y+2x +1
Answers
Answer:
Step-by-step explanation:
Sorry, but Ummm the question doesn't make sense
plz help you only have to recreate them in another way plz

Answers
in how many ways can we list the digits {1, 1, 2, 2, 3, 4, 5} so that two identical digits are not in consecutive positions?
Answers
There are 144 ways to list the digits {1, 1, 2, 2, 3, 4, 5} such that two identical digits are not in consecutive positions.
We have to list the digits {1, 1, 2, 2, 3, 4, 5} so that two identical digits are not in consecutive positions. We can use the knowledge of permutations and combinations to solve this problem. We can start by selecting the positions for the distinct digits, i.e., (3, 4, 5). There are 3 distinct digits, so there are 3! = 6 ways to arrange them.
Once we have placed the distinct digits, we can place the two 1's and two 2's in the remaining positions such that no two identical digits are in consecutive positions. To do this, we can use the following approach:
We can start by placing one of the 1's. There are 4 positions available for the first 1.
We can then place one of the 2's. There are 3 positions available for the first 2.
We can then place the second 1. There are 2 positions available for the second 1.
We can then place the second 2. There are 1 positions available for the second 2.
Therefore, the total number of ways to list the digits {1, 1, 2, 2, 3, 4, 5} such that two identical digits are not in consecutive positions is:
6 (arrangements of distinct digits) x 4 x 3 x 2 x 1 = 144.
To learn more about permutations and combinations visit : https://brainly.com/question/28065038
#SPJ11
How do you differentiate y=x^(e^x) ?

Answers
We are given the function to differentiate:
\({\quad \qquad \sf \rightarrow y=x^{e^x}}\)
Do take natural log on both sides, then we will be having
\({:\implies \quad \sf ln(y)=ln(x^{e^{x}})}\)
\({:\implies \quad \sf ln(y)=e^{x}ln(x)\quad \qquad \{\because ln(a^b)=bln(a)\}}\)
Now, differentiate both sides by using so called chain rule and the product rule
\({:\implies \quad \sf \dfrac{1}{y}\dfrac{dy}{dx}=e^{x}ln(x)+\dfrac{e^x}{x}}\)
\({:\implies \quad \boxed{\bf{\dfrac{dy}{dx}=x^{e^x}\bigg\{\dfrac{e^x}{x}+ln(x)e^{x}\bigg\}}}}\)
Hence, Option B) is correct
Product rule of differentiation:
\({\boxed{\bf{\dfrac{d}{dx}(uv)=u\dfrac{dv}{dx}+v\dfrac{du}{dx}}}}\)Where, u and v are functions of x
1
Write 0.071 64 correct to 2 significant figures.
Answers
Answer: 0.071
Sig Figs
2
0.071
Decimals
3
0.071
Scientific Notation
7.1 × 10-2
E-Notation
7.1e-2
Words
zero point zero seven
Step-by-step explanation:
The term 0.07164 can be written as 0.072 correct to 2 significant figures is.
What is Simplification?Simplification in mathematical terms is a process to convert a long mathematical expression in simple and easy form.
The given term is 0.07164
In decimal number, the zeros before a non-zero digit are not significant.
In the given term, the zero before digit seven is insignificant,
And after digit 1 the number is 6 which is greater than 5, so it will convert into 6 by round off.
Therefore, the required figure of 0.07164 correct to 2 significant figures is 0.072.
To know more about Simplification on :
https://brainly.com/question/2804192
#SPJ2
Solving special systems
{y=1/2x+4
{-1/2x+y=-5
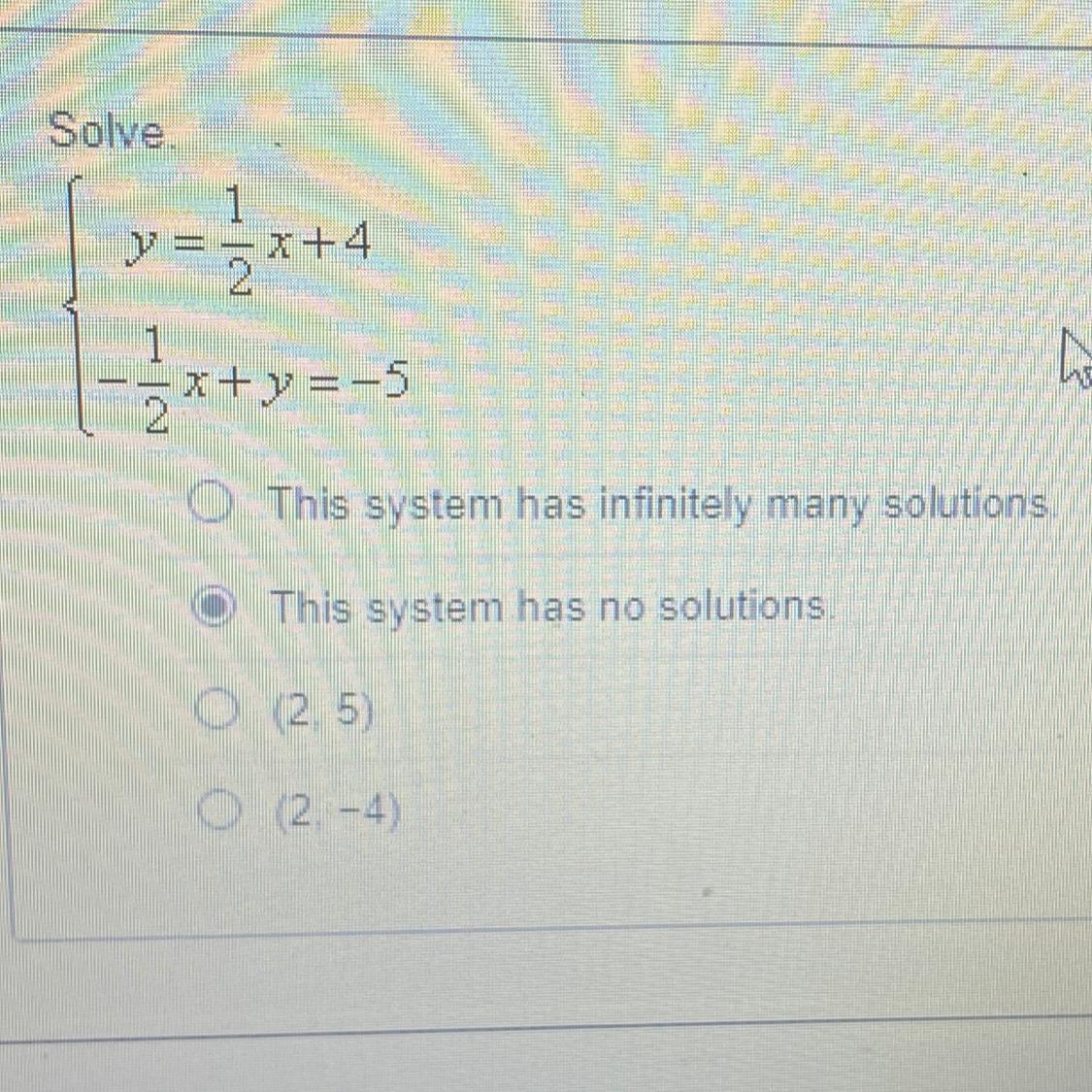
Answers
Answer:
no solution
Step-by-step explanation:
Given the 2 equations
y = \(\frac{1}{2}\) x + 4 → (1)
- \(\frac{1}{2}\) x + y = - 5 → (2)
Substitute y = \(\frac{1}{2}\) x + 4 into (2)
- \(\frac{1}{2}\) x + \(\frac{1}{2}\) x + 4 = - 5 , that is
4 = - 5 ← not possible
This indicates the system has no solution
what is 6(5g + 2) = 30g
Answers
Answer:
g ∈ ∅
Step-by-step explanation:
Expand the expression
30 g + 12 = 30
Cancel out the 30g on both sides
12 = 0
Decide whether the statement is true or false
g ∈ ∅
What is 100 ÷ 5 × 4 + 43
A. 69
B. 144
C. 0.3
D. 1.2
Answers
Answer:
123
Step-by-step explanation:
100 ÷ 5 x 4 + 43
20 x 4 + 43
80 + 43
123
Find the value of x
………..

Answers
Answer:
135°
Step-by-step explanation:
In a hexagon, sum of all angles = 720
x + x + x + x + 90 + 90 = 720
4x + 180 = 720
4x = 720 - 180
4x = 540
x = 135°
Tara and Veronica are in charge of making a banner for the volleyball game this Saturday. How much poster paper will they need for a parallelogram-shaped banner with height 3 12 feet and base 6 14 feet( i need help plz quick helpppppppp smart peeps ill give you (1-0000000000000000000000000) dollers plz
Answers
Answer:
21 7/8 square feet
Step-by-step explanation:
To solve the above Question, we have to used the formula of a parallelogram
The formula is given as:
Base × Height
Base = 6 1/4 feet
Height = 3 1/2 feet
Area = 6 1/4 × 3 1/2
Area = 25/4 × 7/2
Area = 175/8
Area = 21 7/8 ft²
Therefore, they would need 21 7/8 square feet poster paper for the parallelogram shaped banner
Help! Look at the pic of attached. I will mark brainliest!

Answers
Answer:
you did not attach a paper
Step-by-step explanation:
sorry I wish I could help
1. Use the geometric series formula 1 / 1−y=[infinity]∑n=0 y^n1 to express the function as a series:
1 /1−sin^5 x =[infinity]∑n=0
2. To test the series [infinity]∑n=1e^−5n for convergence, you can use the Integral Test. (This is also a geometric series, so we could also investigate convergence using other methods.)
Find the value of ∫1 to [infinity] e^−5x dx=
Answers
1 / (1 - sin^5 x) = [infinity]∑n=0 [sin^5 x]^n * [cos^2 x * (2 - cos^2 x)]^(-1)
the integral ∫1 to [infinity] e^−5x dx converges to a finite value, the series [infinity]∑n=1 e^−5n also converges.
1. To express the function 1 / (1−sin^5 x) as a series using the geometric series formula, we need to first identify the value of y. In this case, y is sin^5 x. Therefore, we have:
1 / (1−sin^5 x) = 1 / [1 - (sin x)^5]
= 1 / [1 - (sin x)^2 * (sin x)^3]
= 1 / [cos^2 x * (1 - sin^2 x) * (1 + sin^2 x + (sin^2 x)^2)]
= 1 / [cos^2 x * cos^2 x * (1 + sin^2 x + (sin^2 x)^2)]
= 1 / [cos^4 x * (1 + sin^2 x + (sin^4 x)/(sin^2 x))]
= 1 / [cos^4 x * (1 + sin^2 x + sin^2 x * (1 - cos^2 x)/(sin^2 x))]
= 1 / [cos^4 x * (1 + sin^2 x + (1 - cos^2 x)/sin^2 x)]
= 1 / [cos^4 x * (2 - cos^2 x)/sin^2 x]
= sin^2 x / [cos^2 x * (2 - cos^2 x)]
Now, we can substitute y = (sin x)^5 into the geometric series formula:
1 / (1 - y) = [infinity]∑n=0 y^n
to get:
1 / (1 - sin^5 x) = sin^2 x / [cos^2 x * (2 - cos^2 x)]
= [infinity]∑n=0 [sin^5 x]^n * [cos^2 x * (2 - cos^2 x)]^(-1)
Therefore,
1 / (1 - sin^5 x) = [infinity]∑n=0 [sin^5 x]^n * [cos^2 x * (2 - cos^2 x)]^(-1)
2. To find the value of ∫1 to [infinity] e^−5x dx using the integral test, we need to first verify that the function f(x) = e^−5x is positive, continuous, and decreasing for x ≥ 1. Since f(x) is a continuous, positive, and decreasing function, we can apply the integral test to determine the convergence of the series.
The integral test states that if a series [infinity]∑n=1 a_n converges and a_n is a positive, decreasing function of n, then the corresponding improper integral ∫1 to [infinity] a(x) dx also converges. Similarly, if the improper integral diverges, then the series also diverges.
In this case, we have:
a_n = e^(-5n)
a_n is a decreasing function of n, since e^(-5n) is a decreasing exponential function.
Therefore, we want to find the value of the improper integral:
∫1 to [infinity] e^−5x dx
Using the substitution u = -5x, du = -5dx, and limits of integration u(1) = -5 and u([infinity]) = -∞, we can rewrite the integral as follows:
∫1 to [infinity] e^−5x dx = ∫-5 to -∞ (1/-5) * e^u du
= (1/-5) * [e^u]_-5^-∞
= (1/-5) * [e^(-∞) - e^(-5)]
= (1/-5) * [0 - 1/e^5]
= 1/(5e^5)
Since the integral ∫1 to [infinity] e^−5x dx converges to a finite value, the series [infinity]∑n=1 e^−5n also converges.
Visit to know more about integrals:-
https://brainly.com/question/22008756
#SPJ11
A 2-column table with 5 rows. Column 1 is labeled Key Words with entries twelve, more than, quotient, a number, eight. Column 2 is labeled Replace with entries 12, +, divided by, k, 8. Write and evaluate the expression. Then, complete the statement. twelve more than the quotient of a number and eight The value of the expression when k = 40 is
Answers
Answer:
17
Step-by-step explanation:
hope it helps
Answer:
The value of the expression when k = 40 is 17.
Step-by-step explanation:
Please give the person that answered before me the brainiest because they deserve it, I just used their answer so you could choose brainiest.
anyone help me with this
Find the volume. Round to two decimal places if necessary. If your answer is correct you will see an image appear on your screen.
its 7in the number i cant put a pic
Answers
Answer:
if it's 5 or more, increase the previous digit by one.
A scientist who studies teenage behavior was interested in determining if teenagers spend more time playing computer games then they did in the 1990s. In 1990s, the average amount of time spent playing computer games was 10. 2 hours per week. Is the amount of time greater than that for this year? twenty students were surveyed and asked how many hours they spent playing video games. The test statistics is equal to 1. 39. What is the p-value?.
Answers
0.3317 is the p-value .
What is the P value?
The likelihood, for a particular statistical model, that the statistical summary would be equal to or more extreme than the actual observed findings when the null hypothesis is true is known as the P value.The test hypothesis should be rejected if the result is statistically significant (P 0.05), which indicates that the test hypothesis is false. There was no effect, according to a P value greater than 0.05.H0 : Mu = 10.2
H1 : Mu > 10.2
Test statistic
t=0.45 (since n is small)
P value = 0.3317
Hence P is greater than 0.10
Learn more about P value
brainly.com/question/28197808
#SPJ4
Which expression is equivalent to 7/8+ 3/8x-1/4y+3/4y-1/2?
Answers
Answer: the answer is in the pics
Step-by-step explanation:


The solution of the expression ( 7/8+ 3/8x-1/4y+3/4y-1/2 ) is 1 / 2 ( 3 / 4 x + y + 3/4).
What is an expression?Expression in maths is defined as the relation of numbers variables and functions by using mathematical signs like addition, subtraction, multiplication and division.
The given expression is ( 7/8+ 3/8x-1/4y+3/4y-1/2 ). The given expression will be simplified as below,
E = ( 7/8+ 3/8x-1/4y+3/4y-1/2 )
E = 1 / 2 ( 7 / 4 + 3/4x -1/2y + 3/2y - 1 )
E = 1/2 ( 3/4x +y + 3/4)
Therefore, the solution of the expression will be E = 1/2 ( 3/4x +y + 3/4).
To know more about an expression follow
https://brainly.com/question/30429467
#SPJ2
Pablo is mailing packages. Each small package costs him $2.20 to send. Each large package costs him $3.70. How much will it cost him to send 1small package and 5 large packages?
Answers
Answer:
$20.70
Step-by-step explanation:
5 × $3.70 = $18.50 + $2.20 =
$20.70
The temperature outside changed from 94°F to 46°F over a period of eight days. If the temperature changed by the same amount each day, what was the daily temperature change?
Answers
Answer:
6°F
Step-by-step explanation:
First, subtract 46 from 94 (94-46) and you will get 48.
Then, divide 48÷8 (because the time period is 8 days) and you will get 6.
Therefore, the daily temperature change is 6°F.
Use a graphing tool to solve the system. {13x + 6y = −30, x − 2y = −4}. Which ordered pair is the best estimate for the solution to the system?
a. (2, -5).
b. (-1, 2).
c. (4, 3). d. (0, -4).
Answers
(B) (-1, 2) ordered pair is the best estimate for the solution to the system.
To solve the system {13x + 6y = −30, x − 2y = −4} using a graphing tool, we need to plot the line for each equation and find the point where they intersect.
This point is the solution to the system.
The intersection point appears to be (-1, 2).
Therefore, the best estimate for the solution to the system is the ordered pair (-1, 2).
Therefore, option b. (-1, 2) is the correct option.
Know more about ordered pair here:
https://brainly.com/question/11139505
#SPJ11
Find the maximum value of p=3x+2y

Answers
Answer:
P = 18
Step-by-step explanation:
You want the maximum value of P = 3x +2y given the constraints ...
5x +y ≤ 162x +3y ≤ 22x ≥ 0y ≥ 0Graphical solutionThe four inequalities define a quadrilateral solution region with vertices ...
(0, 0), (0, 7.333), (2, 6), (3.2, 0)
The value of the objective function P is maximized at (x, y) = (2, 6). Its value there is ...
P(x, y) = 3·2 +2·6 = 6 +12 = 18
The maximum value of P is 18.
__
Additional comment
When the solution region is the overlapping solution spaces of several inequalities, it can be easier to see the solution region if that region is white, rather than four overlapping colors.
In the attached graph, we reversed each of the inequalities so that the solution space is white, and the shaded regions are excluded from the solution space. The boundary lines are all included.
The blue line is the objective function. It is maximized when the line is as far as possible from the origin. We like to plot this line because its slope tells us that we have located the vertex that maximizes P.

Suppose a firm can sell it's output at p per unit and that its production function is given by y = AK∝Lβ, where K > 0 is capital input measured in machine-hours, L > 0 is labor input measured in worker-hours and A,∝, ß > 0 are parameters. The firm is perfectly competitive and the factor prices are r per hour and w per hour. (a) Show by partial differentiation that the production function has the property of increasing marginal productivity of capital (if ∝ > 1) and of labor (if ß > 1). Explain the economic significance of this. Does it explain why we normally assume that a and 3 are less than 1?
Answers
Increasing marginal productivity infers that extra units of capital and labor contribute more to yield, driving productive asset allotment. ∝ and ß < 1 expect reducing returns, adjusting with reality.
The production function has the property of increasing the marginal productivity of capital through Partial Differentiation.To appear that the generation work has to expand the marginal productivity of capital (in case ∝ > 1) and labor (on the off chance that ß > 1), we ought to take fractional subsidiaries with regard to each input calculation. For capital (K), the fractional subsidiary of the generation work is:
\(\dfrac{dy}{dK }= \alpha AK^{(\alpha-1)}L^\beta\)
Since ∝ > 1, (∝ - 1) is positive, which implies that the fractional subordinate \(\dfrac{dy}{dK}\) is positive. This shows that an increment in capital input (K) leads to an increment in yield (y), appearing to expand the marginal efficiency of capital.
Additionally, for labor (L), the fractional subordinate of the generation work is:
\(\dfrac{dy}{dL} = \beta AK^{\alpha}L^{(\beta-1)}\)
Since \(\mathbf{\beta > 1, (\beta-1)}\) it is positive, which implies that the halfway subordinate \(\dfrac{dy}{dL}\) is positive. This demonstrates that an increment in labor input (L) leads to an increment in yield (y), appearing to increase the marginal productivity
The economic importance of increasing marginal productivity is that extra units of capital and labor contribute more to yield as their amounts increment. This suggests that the more capital and labor a firm employments, the higher the rate of increment in yield. This relationship is vital for deciding the ideal assignment of assets and maximizing generation effectiveness.
In most generation capacities, it is accepted that ∝ and ß are less than 1. This presumption adjusts with experimental perceptions and financial hypotheses.
In case ∝ or ß were more prominent than 1, it would suggest that the marginal efficiency of the respective factor increments without bound as the calculated input increments.
In any case, there are decreasing returns to scale, which suggests that as calculated inputs increment, the Marginal efficiency tends to diminish. Therefore, accepting ∝ and ß are less than 1 permits for more reasonable modeling of generation forms and adjusts with the concept of diminishing marginal returns.
Learn more about the Marginal productivity of capital here:
https://brainly.com/question/18492635
#SPJ4