What is the solution to the equation x÷8−6=3?
A. x=1
B. x=24
C. x=48
D. x=72
30 POINTS TO THE RIGHT ANSWER AND BEST EXPLANTION.
Answers
Answer: D. x=72
Step-by-step explanation:
With the equation x/8-6=3 we can start solving by first moving the "-6"
x/8-6(+6)=3(+6)
x/8=9 <-- Rewrite equation after adding "6" to both sides
x/8(*8)=9(*8) <--- Multiply both sides by 8
x=72 <-- After multiplying, solution is found
Related Questions
Find the area of the shaded sector of the circle.
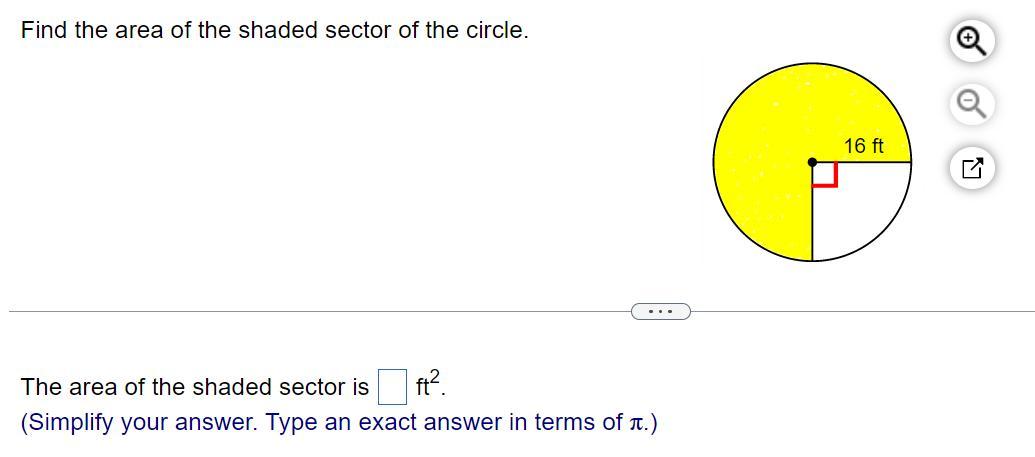
Answers
Answer:
\(192\pi \text{ ft}^2\)
Step-by-step explanation:
We can see that the shaded section of the circle is 3/4 of the total circle. This can be checked by comparing the angles measure of the shaded section with the angle of the entire circle:
\(\dfrac{(360-90)\°}{360\°}\)
\(=\dfrac{270}{360}\)
\(=\dfrac{3}{4}\)
We can find its area by multiplying 3/4 by the area of the entire circle.
\(A = \dfrac{3}{4} \cdot \pi r^2\)
\(A=\dfrac{3}{4} \cdot \pi \cdot 16^2\)
\(A = \dfrac{3}{4} \cdot \pi \cdot 256\)
\(\boxed{A = 192\pi \text{ ft}^2}\)
kirk made a scale drawing of the elementary school. the scale of the drawing was 3 millimeters : 4 meters. if the actual width of the schoolyard is 60 meters, how wide is the schoolyard in the drawing?
Answers
The width of the schoolyard in the drawing is 45 millimeter.
In the given statement is ,
kirk made a scale drawing of the elementary school. the scale of the drawing was 3 millimeters : 4 meters. if the actual width of the schoolyard is 60 meters.
To find the how wide is the schoolyard in the drawing
Now, According to the question:
and, Based on the given condition
Let width of the schoolyard in the drawing be x
So, 3 : 4 = x : 60
[If b/a = d/c, ad = bc]
3/4 = x/60
3 x 60 = 4x
x = 180/4
x = 45 millimeters
Hence, The width of the schoolyard in the drawing is 45 millimeter.
Learn more about Width of the schoolyard at:
brainly.com/question/25712759
#SPJ4
in the _____ theory of interpersonal relationship satisfaction, you would feel happiest in relationships when you feel that the rewards of the relationship equal or exceed its costs.
Answers
The Social Exchange Theory of interpersonal relationship satisfaction suggests that individuals are motivated to stay in a relationship as long as they feel that the rewards of the relationship are greater than the costs.
In the Social Exchange Theory of interpersonal relationship satisfaction, you would feel happiest in relationships when you feel that the rewards of the relationship equal or exceed its costs.
The Social Exchange Theory states that people evaluate their relationships in economic terms, with rewards being the benefits of the relationship and costs being the negatives. Rewards can include affection, attention, emotional support, sex, and companionship. Costs can include time, effort, money, and negative emotions such as stress, anxiety, and sadness.
According to the theory, individuals will be motivated to stay in a relationship as long as they feel that the rewards of the relationship are greater than the costs.
The opposite is also true; if individuals feel that the costs of the relationship are greater than the rewards, they will be motivated to leave the relationship.
Therefore, people are constantly evaluating their relationships to determine whether they are getting what they need from the relationship and whether it is worth continuing.
The Social Exchange Theory of interpersonal relationship satisfaction suggests that individuals are motivated to stay in a relationship as long as they feel that the rewards of the relationship are greater than the costs.
Learn more about costs here:
https://brainly.com/question/28778430
#SPJ11
You would like to study all of the numbers that are at a distance 10 or less from a number -20. Write this using absolute value notation and use the variable x
Answers
Answer:
Step-by-step explanation:
dad hates me sorry bye
Checking for approximate normality in the population is essential for constructing a valid confidence interval, particularly when dealing with small sample sizes. This ensures the accuracy and reliability of the interval in estimating the true population parameter.
It's important to check whether the population is approximately normal before constructing a confidence interval because the accuracy and validity of the interval depend on the underlying distribution of the population. Here's a step-by-step explanation:
1. A confidence interval is a range of values within which the true population parameter (e.g., mean or proportion) is likely to fall, with a certain level of confidence (e.g., 95% or 99%).
2. The process of constructing a confidence interval relies on the Central Limit Theorem, which states that, for large sample sizes, the sampling distribution of the sample mean will be approximately normal, regardless of the population distribution.
3. However, for small sample sizes, the distribution of the population needs to be approximately normal in order to obtain an accurate confidence interval. This is because the normality assumption is crucial for the proper interpretation of the interval.
4. If the population is not approximately normal, the confidence interval may not provide a reliable estimate of the true population parameter, leading to incorrect conclusions and potentially invalid results.
Learn more about Central limit theorem here: brainly.com/question/18403552
#SPJ11
4 Tan A/1-Tan^4=Tan2A + Sin2A
Answers
tan(2A) + sin(2A) = sin(2A)/cos(2A) + sin(2A)
• rewrite tan = sin/cos
… = 1/cos(2A) (sin(2A) + sin(2A) cos(2A))
• expand the functions of 2A using the double angle identities
… = 2/(2 cos²(A) - 1) (sin(A) cos(A) + sin(A) cos(A) (cos²(A) - sin²(A)))
• factor out sin(A) cos(A)
… = 2 sin(A) cos(A)/(2 cos²(A) - 1) (1 + cos²(A) - sin²(A))
• simplify the last factor using the Pythagorean identity, 1 - sin²(A) = cos²(A)
… = 2 sin(A) cos(A)/(2 cos²(A) - 1) (2 cos²(A))
• rearrange terms in the product
… = 2 sin(A) cos(A) (2 cos²(A))/(2 cos²(A) - 1)
• combine the factors of 2 in the numerator to get 4, and divide through the rightmost product by cos²(A)
… = 4 sin(A) cos(A) / (2 - 1/cos²(A))
• rewrite cos = 1/sec, i.e. sec = 1/cos
… = 4 sin(A) cos(A) / (2 - sec²(A))
• divide through again by cos²(A)
… = (4 sin(A)/cos(A)) / (2/cos²(A) - sec²(A)/cos²(A))
• rewrite sin/cos = tan and 1/cos = sec
… = 4 tan(A) / (2 sec²(A) - sec⁴(A))
• factor out sec²(A) in the denominator
… = 4 tan(A) / (sec²(A) (2 - sec²(A)))
• rewrite using the Pythagorean identity, sec²(A) = 1 + tan²(A)
… = 4 tan(A) / ((1 + tan²(A)) (2 - (1 + tan²(A))))
• simplify
… = 4 tan(A) / ((1 + tan²(A)) (1 - tan²(A)))
• condense the denominator as the difference of squares
… = 4 tan(A) / (1 - tan⁴(A))
(Note that some of these steps are optional or can be done simultaneously)
On the day of the test, the teacher instructed the students to take out no less than 2 pencils from their backpacks. determine which inequality represents this scenario. 2 ≥ p 2 ≤ p 2 > p 2 < p
Answers
The required inequality is \(p\geq2\)
What is linear inequation?
Inequation shows the comparision between two algebraic expressions by connecting the two algebraic expressions by \(> , < , \geq, \leq\)
A one degree inequation is known as linear inequation.
On the day of the test, the teacher instructed the students to take out no less than 2 pencils from their backpacks.
Let the number of pencils be p
So the required inequality is \(p\geq2\)
To learn more about linear inequation, refer to the link:
https://brainly.com/question/24372553
#SPJ4
Four friends bought an extra-large $12 popcorn. Each person bought their own drink. The total cost of the food can be represented by the expression 4x + $12.
Answers
Answer:
True
Step-by-step explanation:
This equation correctly represents the total cost of the food that the friends ended up paying for the popcorn and sodas. In this expression, the cost of each individual soda is represented by the variable x which is multiplied by 4 since each one of the four friend's purchased their own soda. After making this multiplication process that product is added to the initial $12 that they all spent together on the popcorn.
Each friend paid $4 for popcorn.
The total cost of the food can be represented by the expression 4x + $12.
Given
Four friends bought an extra-large $12 popcorn.
Each person bought their own drink.
Let x be the number of friends.
The total cost of food= Total number of friends + cost of popcorn.
The total cost of the food can be represented by the expression 4x + $12.
Therefore;
The amount paid by each friend is;
\(\rm 4x=12\\\\x=\dfrac{12}{3}\\\\x=4\)
Hence, each friend paid $4 for popcorn.
To know more about Equation click the link given below.
https://brainly.com/question/2272409
please help last question 50 X 2 - 10
Answers
the answer is 90
50 ×2 = 100
100 - 10 = 90
A situation in which conclusions based upon aggregated crosstabulation are different fromunaggregated crosstabulation is known asa.wrong crosstabulationb.Simpson's rulec.Simpson's paradoxd.aggregated crosstabulationANS: C
Answers
A situation in which conclusions based upon aggregated crosstabulation are different from unaggregated crosstabulation is known as Simpson's paradox.
Simpson’s Paradox is an example of statistics that can be wrong. The paradox is defined as averages that can be silly and misleading. Sometimes they can be just plain baffling.
It is also called the Yule-Simpson effect which is an effect that occurs when the marginal association between two categorical variables is qualitatively different from the partial association between the same two variables after controlling for one or more other variables.
This result is often encountered in social science and medical science statistics and is particularly problematic when frequency data are unduly given causal interpretations.
It is a statistical phenomenon where an association between two variables in a population emerges or reverses when the population is divided into subpopulations.
To know more about Simpson's paradox:
https://brainly.com/question/2737125
#SPJ4
PLS HELP ASAP! Ty
Let g(x)= 2x^2+3x-9 and h(x)=x^2+2x-6
Find (h-g)(2.1)
Answers
\( {\qquad\qquad\huge\underline{{\sf Answer}}} \)
Let's solve ~
\(\qquad \sf \dashrightarrow \: (h - g)(x) \)
\(\qquad \sf \dashrightarrow \: h(x) - g(x) \)
\(\qquad \sf \dashrightarrow \: {x}^{2} + 2x - 6 - (2 {x}^{2} + 3x - 9)\)
\(\qquad \sf \dashrightarrow \: {x}^{2} + 2x - 6 - 2 {x}^{2} - 3x + 9\)
\(\qquad \sf \dashrightarrow \: {x}^{2} - 2 {x}^{2} + 2x - 3x - 6 + 9\)
\(\qquad \sf \dashrightarrow \: - {x}^{2} - x + 3\)
For, (h - g)(2.1), put x = 2.1
\(\qquad \sf \dashrightarrow \: - {(2.1)}^{2} - (2.1) + 3\)
\(\qquad \sf \dashrightarrow \: - 4.41 - 2.1 + 3\)
\(\qquad \sf \dashrightarrow \: - 6.51 + 3\)
\(\qquad \sf \dashrightarrow \: - 3.51\)
Johnny can build 3 1/2 lego planes in 60 minutes. How many can he build in 40 minutes?
the answer needs to be a mixed fraction
Answers
2 1/3 Lego planes every 40 minutes
Who can help.? Feel lazy to do my homework

Answers
SO anyways :))))
Thanks and please make sure you comment only if you are pretty sure you are right! Thanks

Answers
Answer:
1 / 8², B
Step-by-step explanation:
8 / 8³
8³ = 8*8*8
So, the equation can also be written as:
8 / 8*8*8
divide the numerator and denominator by 8.
1 / 8*8
This is equal to:
1 / 8²
The answer is B.
help ! do you know the answer to this?

Answers
Answer:
Step-by-step explanation:
9t = 9 We can't see the entire image, but I assume 9 and the number of ts are of the same length. If this assumption is incorrect, please leave a note.
You went 160 miles in 20 hours. What is the constant of proportionality that relates the y, the distance in miles to x, the time in hours?
Answers
Answer:
X = Y times 8
Step-by-step explanation:
You need to figure out how many miles you can go in an hour.
160/x and 20/1
20x=160
x=8
You can go 8 miles in 1 hour.
Therefore, if X is 1 and Y is 1
1 = 8
1 hour equals 8 miles
these marbles are placed in a bag and two of them are randomly drawn. yellow=2 pink=3 blue=5 what is the probability of drawing 2 yellow marbles if the first one is not placed back into the bag before the second one. Give your answer as a rational number, reduced to simplest terms.

Answers
Answer: 1/45
Step-by-step explanation:
From thw question, we are told that there are:
Yellow marbles =2
Pink marbles =3
Blue marbles = 5
Total marbles = 2+3+5 = 10
The probability of drawing the first yellow ball will be= 2/10
Since the ball is not replaced, that means there will be 9 balls left and 1 yellow ball left. Therefore, the probability of drawing the second yellow ball will be = 1/9
The probability of drawing two yellow balls if they're not replaced will now be:
= 2/10 × 1/9
= 2/90
= 1/45
A stock just paid a dividend of $1.55. The dividend is expected to grow at 26.56% for three years and then grow at 3.42% thereafter. The required return on the stock is 14.40%. What is the value of the stock?
Answers
Here, we are supposed to find the value of the stock. Let's begin by determining the expected dividends: Expected dividends1st year dividend (D1)
= $1.55(1 + 26.56%)
= $1.96Second-year dividend (D2) = $1.96(1 + 26.56%) = $2.48Third-year dividend (D3)
= $2.48(1 + 26.56%)
= $3.
= D1/(1+r)^1 + D2/(1+r)^2 + D3/(1+r)^3 + D4/(1+r)^4...∞Where r
= required rate of return Let us substitute the values now PV of the future dividends
= $1.96/(1 + 14.40%)^1 + $2.48/(1 + 14.40%)^2 + $3.14/(1 + 14.40%)^3 + $3.25/(1 + 14.40%)^4...∞PV of the future dividends = $1.96/1.1440^1 + $2.48/1.1440^2 + $3.14/1.1440^3 + $3.25/1.1440^4...∞PV of the future dividends
= $1.72 + $1.92 + $2.04 + $1.86...∞PV of the future dividends
= $7.54We know that the value of the stock is the present value of the expected dividends, so we can calculate it as follows: Value of the stock
= PV of the future dividends Value of the stock
= $7.54
To know more about future visit:
https://brainly.com/question/1621783
#SPJ11
please help determine if these are functions and explain why or why not

Answers
Answer:The first is a function but the second isn’t.
Step-by-step explanation: The numbers in the first circle (domain) should only point to one number in the second circle (range). Meaning the domain should only have one arrow for it to be a function. Therefore the first problem is a function, but the second problem is not. For
example, in the first problem, the 0 is only pointing to one number, the 2. However, in the second problem the 6 is pointing to two different numbers, the 3 and the 5. -Let me know if this helps you or if you want me to explain it more. Hope this helps! :)
$En la cima del Chopicalqui se encuentra un alpinista, si la cima está a una altura de 6746 m. desciende 429 m. Otro alpinista se encuentra a 280 m antes de la cima y luego asciende 115 m. ¿Cuál es la diferencia entre las alturas en las que se encuentran los 2 alpinistas? por fa doy corona y corazon
Answers
Answer:
La diferencia entre las alturas en que se encuentran los 2 alpinistas es de 264 metros.
Step-by-step explanation:
Dado que en la cima del Chopicalqui se encuentra un alpinista, si la cima está a una altura de 6746 m y éste desciende 429 m, mientras que otro alpinista se encuentra a 280 m antes de la cima y luego asciende 115 m, para determinar cuál es la diferencia entre las alturas en las que se encuentran los 2 alpinistas se debe realizar el siguiente calculo:
(6746 - 429) - (6746 - 280 + 115) = X
6317 - (6466 + 115) = X
6317 - 6581 = X
-264 = X
Por lo tanto, la diferencia entre las alturas en que se encuentran los 2 alpinistas es de 264 metros.
Calculate ∬f(x,y,z)dS For x^2+y^2=25,0≤z≤8;f(x,y,z)=e^(−z) ∬f(x,y,z)dS
Answers
The double integral ∬ f(x, y, z) dS is equal to (-e^(-8) + 1) (25π).
To calculate the double integral ∬ f(x, y, z) dS, we need to evaluate the integral over the surface defined by x^2 + y^2 = 25, and 0 ≤ z ≤ 8, where f(x, y, z) = e^(-z).
We can express the surface in cylindrical coordinates, where x = r cos(θ), y = r sin(θ), and z = z. The bounds for the variables are r ∈ [0, 5] (since x^2 + y^2 = 25 corresponds to r = 5), θ ∈ [0, 2π], and z ∈ [0, 8].
The differential element of surface area in cylindrical coordinates is given by dS = r dz dr dθ. Thus, the double integral becomes:
∬ f(x, y, z) dS = ∫∫∫ f(x, y, z) r dz dr dθ
Substituting f(x, y, z) = e^(-z) and the bounds, we have:
∬ f(x, y, z) dS = ∫[0,2π] ∫[0,5] ∫[0,8] e^(-z) r dz dr dθ
Now, let's evaluate the integral step by step:
∫[0,2π] ∫[0,5] ∫[0,8] e^(-z) r dz dr dθ
= ∫[0,2π] ∫[0,5] [-e^(-z)] [0,8] r dr dθ
= ∫[0,2π] ∫[0,5] (-e^(-8) + e^(-0)) r dr dθ
= ∫[0,2π] ∫[0,5] (-e^(-8) + 1) r dr dθ
= (-e^(-8) + 1) ∫[0,2π] ∫[0,5] r dr dθ
Now, evaluate the inner integral:
∫[0,5] r dr = [(1/2) r^2] [0,5] = (1/2) (5^2 - 0^2) = (1/2) (25) = 12.5
Substitute this result back into the expression:
(-e^(-8) + 1) ∫[0,2π] 12.5 dθ
= (-e^(-8) + 1) (12.5θ) [0,2π]
= (-e^(-8) + 1) (12.5)(2π - 0)
= (-e^(-8) + 1) (25π)
Therefore, the double integral ∬ f(x, y, z) dS is equal to (-e^(-8) + 1) (25π).
Learn more about double integral here:
https://brainly.com/question/2289273
#SPJ11
a survey of 25 grocery stores revealed that the average price of a gallon of milk was $2.98, with a standard error of $0.10. what is the 98% confidence interval to estimate the true cost of a gallon of milk? $2.85 to $3.11 $2.73 to $3.23 $2.95 to $3.01 $2.94 to $3.02
Answers
The 98% confidence interval to estimate the true cost of a gallon of milk is $2.73 to $3.23.
What do you mean by standard error?The standard error is a measure of the variability or spread of a set of sample values. In statistics, the standard error is used to quantify the precision of an estimate of a population parameter, such as the mean or standard deviation. It is calculated as the standard deviation of the sampling distribution of a statistic, usually the mean. The standard error is a useful measure because it provides a way to compare different estimates of a population parameter, and it can be used to construct confidence intervals, which provide a range of values within which the true population parameter is likely to lie with a specified degree of confidence.
The 98% confidence interval to estimate the true cost of a gallon of milk can be calculated using the following formula:
CI = (sample mean) ± (critical value) * (standard error)
Where critical value can be obtained from a standard normal distribution table, corresponding to a given level of confidence.
For 98% confidence, the critical value is 2.33.
CI = $2.98 ± 2.33 * $0.10 = $2.98 ± $0.23 = $2.73 to $3.23
Therefore, the 98% confidence interval to estimate the true cost of a gallon of milk is $2.73 to $3.23
To know more about mean visit:
https://brainly.com/question/20393049
#SPJ4
Find a basis for the eigenspace corresponding to each listed
eigenvalue of A below.
a=
[4 16 -10]
[0 0 1 ]
[0 -2 3]
A basis for the eigenspace corresponding to λ=4 is :
A basis for the eigenspace corresponding to λ=1 is "
Answers
A basis for the eigenspace corresponding to λ=1 is any non-zero vector of the form:
[-2 3 -3]^T, where ^T denotes transpose
To find a basis for the eigenspace corresponding to an eigenvalue λ, we need to solve the equation:
(A - λI)x = 0
where A is the matrix, I is the identity matrix of the same size as A, and x is the eigenvector. The solutions to this equation form a vector space called the eigenspace corresponding to the eigenvalue λ.
For the given matrix A,
A = [4 16 -10]
[0 0 1 ]
[0 -2 3]
For λ=4, we need to solve the equation (A-4I)x=0:
(A-4I) = [0 16 -10]
[0 -4 1 ]
[0 -2 -1]
So, we need to solve the system of linear equations:
0x1 + 16x2 - 10x3 = 0
0x1 - 4x2 + 1x3 = 0
0x1 - 2x2 - 1*x3 = 0
We can use row reduction to solve this system of equations and obtain the following row echelon form:
[0 8 -3]
[0 0 1]
[0 0 0]
The solution to this system is x2 = 3/8 and x3 = 1, with x1 being a free variable. Therefore, a basis for the eigenspace corresponding to λ=4 is any non-zero vector of the form:
[1/8 3/8 1]^T, where ^T denotes transpose.
For λ=1, we need to solve the equation (A-I)x=0:
(A-I) = [3 16 -10]
[0 -1 1]
[0 -2 2]
So, we need to solve the system of linear equations:
3x1 + 16x2 - 10x3 = 0
0x1 - 1x2 + 1x3 = 0
0x1 - 2x2 + 2*x3 = 0
We can use row reduction to solve this system of equations and obtain the following row echelon form:
[3 0 2]
[0 -1 1]
[0 0 0]
The solution to this system is x1 = -2/3, x2 = -1, and x3 being a free variable. Therefore, a basis for the eigenspace corresponding to λ=1 is any non-zero vector of the form:
[-2 3 -3]^T, where ^T denotes transpose
Learn more about eigenspace here:
https://brainly.com/question/28564799
#SPJ11
x²-13x=0 T
he solution set to the given equation is ___ (Type an integer or a simplified fraction. Use a comma to separate answers as needed.)
Answers
To find the solution set to the equation x² - 13x = 0, we can factor out the common factor x: x(x - 13) = 0
Now we have two factors, x and (x - 13), which multiply to give zero. To find the solutions, we set each factor equal to zero and solve for x: x = 0
x - 13 = 0. The first equation gives us x = 0, and the second equation gives us x = 13.Hence the answer is {0,13}.
Therefore, the solution set to the equation x² - 13x = 0 is {0, 13}.
To learn more about common factor click here: brainly.com/question/30961988
#SPJ11
B) Arturo compró un automóvil usado y pago $2.500.000. Si este automóvil se devalúa (baja su precio) en un 20% anual. ¿Cuánto se devalúa el primer año el precio del automóvil?
Answers
Answer:
$500.000
Step-by-step explanation:
Lo que debemos hacer es calcular el porcentaje de devaluación del precio total del automóvil, es decir calcular el 20% de $2.500.000, y eso lo podemos hacer de la siguiente manera:
2500000*0.2 = 500000
Lo que quiere decir que el primer año el automóvil de devaluó en $500.000
Find the segment length indicated. Assume that lines which appear tobe tangent are tangent.?1220

Answers
Answer:
16
Explanation:
The segment with a length equal to 12 is tangent to the circle, It means that it forms an angle of 90 degrees with the line of the missing length.
So, the formed triangle is a right triangle and we can apply the Pythagorean theorem to solve the question.
Then, since 20 is the hypotenuse of the triangle, we get that the missing side can be calculated as:
\(\text{? = }\sqrt[]{20^2-12^2}\)So, solving the expression, we get:
\(\begin{gathered} \text{? = }\sqrt[]{400-144} \\ \text{? = }\sqrt[]{256} \\ \text{? = 16} \end{gathered}\)Therefore, the segment length is 16.
Someone please answer this

Answers
Answer:
(x - 1)(5x - 6)
Step-by-step explanation:
Given
5x² - 11x + 6
Consider the factors of the product of the coefficient of the x² term and the constant term (ac) which sum to give the coefficient of the x- term (b)
ac = 5 × 6 = 30 and b = - 11
The factors are - 5 and - 6
Use these factors to split the x- term
5x² - 5x - 6x + 6 ( factor the first/second and third/fourth terms )
= 5x(x - 1) - 6(x - 1) ← factor out (x - 1) from each term
= (x - 1)(5x - 6)
Which is equivalent to 3√8x?
Answers
It is equivalent to 8^x/3
What is equivalent equations?OBJECTIVES FOR EQUALITY EQUATIONSAfter finishing this section, you need to be able to:Sort an equation into a conditional or an identity category.Mentally solve simple equations.Check to see if some equations are equal.A mathematical equation is a statement that two numerical expressions are equal.Equations go into one of two categories.acc to our question-
to write a square root into fraction. To do that, you just have to apply the formula :
x^power/root
Then, here it gives . 8^x/3
learn more about equivalent equations click here:
https://brainly.com/question/2972832
#SPJ13
Solve 12x + 5 = 4x + 37
Answers
Step-by-step explanation:
12x-4x=37-5
8x=32
x=32/8
x=4
someone rolls a fair six-sided die and you win points equal to the number shown. what is the expected number of points after one roll?
Answers
If you roll a fair six-sided die, you will receive the indicated number of points. After one roll, 3.5 points should be expected.
A six-sided cube with the digits 1-6 printed on the faces is the most popular type of die. The value of the roll is indicated by the number of "spots" that are visible at the top. The opposite faces on the six-sided die are positioned so that they always add up to seven. The word "die" is derived from Old French and Latin datum, which means "anything provided or played." The names of the numbers are preferable, but some seasoned gamblers still refer to the different sides of the dice by using the terms ace, deuce, trey, cater, cinque, and dice.
Add up all of the die's pips., \(1+2+3+4+5+6=21\).
The likelihood of a specific face being up on any roll of a single die is \(1/6\).
After one roll, the anticipated number of points is \(21/6= 3.5\)
Learn more about angle of elevation here
https://brainly.com/question/29804056
#SPJ4
Need help please will give brainliest


Answers
Answer:
x = 60
Step-by-step explanation:
The formula for this kind of question is
Area of sector = x / 360 * pi * r^2 = 24*pi
x/360 * pi * 12^2 = 24 pi
x/360 * pi * 144 = 24 pi the pi s cancel out
x / 360 = 24/144 Multiply both sides by 360
x = 24 * 360/144
x = 60